Mapping the Earth
+8
Dan-cer
TG123
Hauchfer
outerworlds44
leotruther
k4t
Safesteef
RedorBlue
12 posters
IFERS - Exposing the 'Global' Conspiracy From Atlantis to Zion :: Reference Materials, eBooks, Articles, Videos, Maps
Page 3 of 5
Page 3 of 5 •  1, 2, 3, 4, 5
1, 2, 3, 4, 5 
 Re: Mapping the Earth
Re: Mapping the Earth
leotruther has shown an obvious issue with my calculation of Latitude.
This is because I have been going about the calculation in an indirect manner and without completing the final step.
Let me explain.
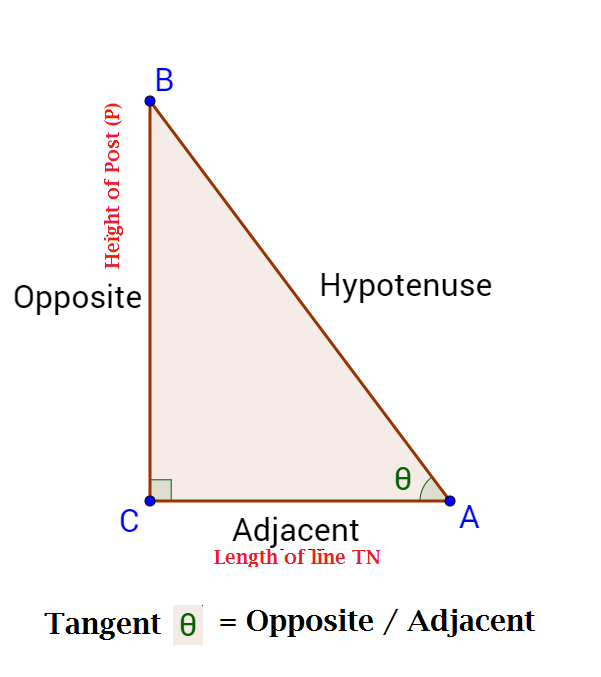
For the indirect calculation of Latitude, I have been using the angle .
.
This is okay if I complete the calculation by subtracting from 90 degrees to get the value for the angle γ (gamma) .
from 90 degrees to get the value for the angle γ (gamma) .
See the following:

γ (gamma) = 90 minus
which equals the latitude plus or minus the declination of the Sun at your location
So, in leotruther's calculation of Latitude, he arrived at: 66° 52' 47.48" South Latitude
Since the calculation is based on the Indirect method I laid out, to complete the calculation for Latitude we will subtract 66° 52' 47.48" from 90 degrees to obtain the value of angle γ gamma.
90 minus 66° 52' 47.48" = 23° 07' 12.52" South Latitude. So, in leotruther's case, he must live very close to the Tropic of Capricorn!! Any signage for the Tropic of Capricorn in your neighborhood?
Note: The larger degree of angle puts one closer to the Equator. I did not see this obvious issue as I reside very near to the 45th degree North latitude!
puts one closer to the Equator. I did not see this obvious issue as I reside very near to the 45th degree North latitude!
The Indirect Method of Finding Latitude has additions and subtractions whereas the Direct Method which I will describe next will simply reverse those subtractions and additions since we will be dealing with the angle of most consequence -- γ (gamma)
BDH
This is because I have been going about the calculation in an indirect manner and without completing the final step.
Let me explain.

For the indirect calculation of Latitude, I have been using the angle
 .
.This is okay if I complete the calculation by subtracting
 from 90 degrees to get the value for the angle γ (gamma) .
from 90 degrees to get the value for the angle γ (gamma) .See the following:

γ (gamma) = 90 minus

which equals the latitude plus or minus the declination of the Sun at your location
So, in leotruther's calculation of Latitude, he arrived at: 66° 52' 47.48" South Latitude
Since the calculation is based on the Indirect method I laid out, to complete the calculation for Latitude we will subtract 66° 52' 47.48" from 90 degrees to obtain the value of angle γ gamma.
90 minus 66° 52' 47.48" = 23° 07' 12.52" South Latitude. So, in leotruther's case, he must live very close to the Tropic of Capricorn!! Any signage for the Tropic of Capricorn in your neighborhood?
Note: The larger degree of angle
 puts one closer to the Equator. I did not see this obvious issue as I reside very near to the 45th degree North latitude!
puts one closer to the Equator. I did not see this obvious issue as I reside very near to the 45th degree North latitude! The Indirect Method of Finding Latitude has additions and subtractions whereas the Direct Method which I will describe next will simply reverse those subtractions and additions since we will be dealing with the angle of most consequence -- γ (gamma)
BDH
Last edited by bdhfe on Thu Aug 12, 2021 5:30 am; edited 1 time in total
bdhfe- Posts : 77
Points : 1896
Reputation : 6
Join date : 2019-08-12
 Re: Mapping the Earth
Re: Mapping the Earth
RedorBlue wrote:You can check true N against the pole star if you can see it - if it aligns with sun position on those days you mention then that's good .
RedorBlue,
Absolutely a fantastic way to CONFIRM one's True North setting. I am thinking of ways to construct a mechanism that will sit on the True North line and project up AT NIGHT so that one can view the trajectory towards the North Star.
BDH
bdhfe- Posts : 77
Points : 1896
Reputation : 6
Join date : 2019-08-12
 Re: Mapping the Earth
Re: Mapping the Earth
Safesteef, k4t , etal
Let me know if you are setting up your own apparatus for this mapping project.
If you or ANYONE does not want to perform their own calculations, I will be happy to do that on this forum if you give me the following data:
1. The current Date.
2. The number of hours you are West or East of the Universal Meridian at Greenwich, England based on the Gleason map
3. The height of your Post (P)
4. The length of the line TN, which is the True North line found by discovering the direction of the meridian above your head. Remember, this is the line that is the shortest distance between the Post (P) and the arc described by the Sun's progress across your surface.
5. The current local time that the Sun crossed the local meridian at your location. This will normally happen on Day Two.
This is how simple it is.
BDH
Let me know if you are setting up your own apparatus for this mapping project.
If you or ANYONE does not want to perform their own calculations, I will be happy to do that on this forum if you give me the following data:
1. The current Date.
2. The number of hours you are West or East of the Universal Meridian at Greenwich, England based on the Gleason map
3. The height of your Post (P)
4. The length of the line TN, which is the True North line found by discovering the direction of the meridian above your head. Remember, this is the line that is the shortest distance between the Post (P) and the arc described by the Sun's progress across your surface.
5. The current local time that the Sun crossed the local meridian at your location. This will normally happen on Day Two.
This is how simple it is.
BDH
bdhfe- Posts : 77
Points : 1896
Reputation : 6
Join date : 2019-08-12
Bro likes this post
 Re: Mapping the Earth
Re: Mapping the Earth
Find Latitude Version 3.0 -- The Direct Method
This is the most straightforward method of finding Latitude using:
-- the Height of the Post
-- the length of the line TN
-- the current declination of the Sun north/south of the equator on the current day
Declination Table

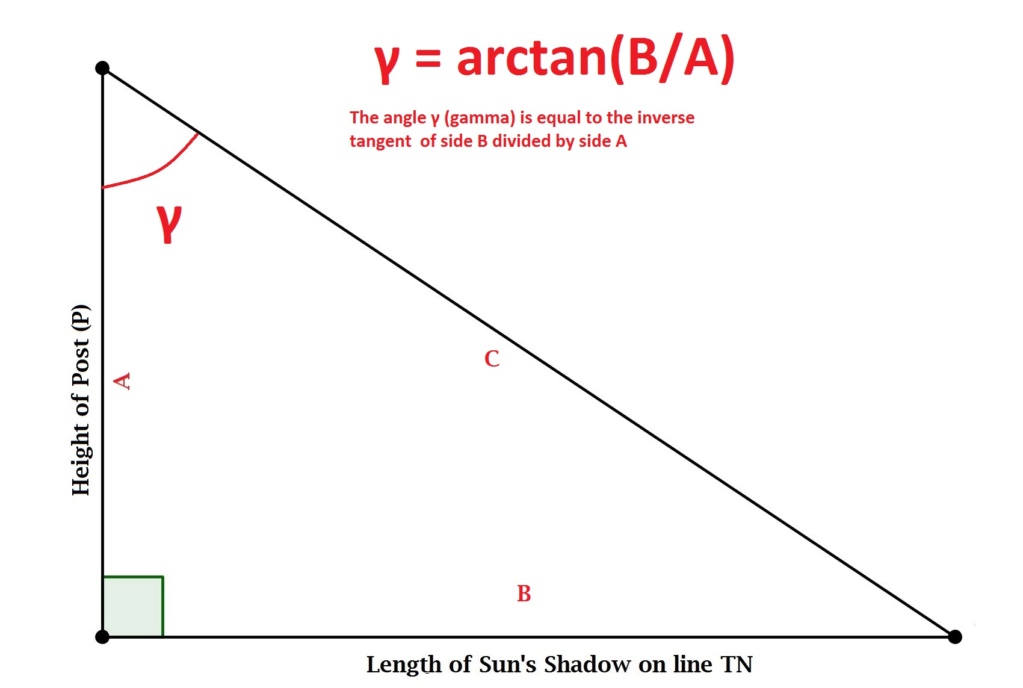
First, the angle Gamma (γ) is calculated by taking the arctangent of the Length of line TN divided by the Height of Post (P).
If one is north of the equator and the declination of the Sun is north of the equator, we will ADD the declination of the Sun on the current day to the angle γ to arrive at the current Latitude of our location. Likewise, if one is south of the equator and the declination of the Sun is south of the equator, we will ADD the angle of declination to γ to arrive at the local Latitude.
If one is north of the equator and the declination of the Sun is south of the equator, then we subtract one from the other to arrive at our local Latitude.
And vice-versa. Subtract if our location is on the opposite side of the equator than the declination of the Sun.
Taking my example from an earlier post:
Height of Post P = 9 and Length of line TN = 4.5.
4.5 divided by 9 = .5
The angle Gamma (γ) = The Arctangent of .5 = 26.56505118°
The Declination of the Sun on that day (July 18th) was 21 degrees 08 minutes or 21.13333°
As both my Location and the Declination of the Sun are North of the equator, we add the Declination 21.13333° to Gamma (γ) 26.56505118° to arrive at 47.69838° = 47° 41' 54.17" North Latitude
BDH
This is the most straightforward method of finding Latitude using:
-- the Height of the Post
-- the length of the line TN
-- the current declination of the Sun north/south of the equator on the current day
Declination Table


First, the angle Gamma (γ) is calculated by taking the arctangent of the Length of line TN divided by the Height of Post (P).
If one is north of the equator and the declination of the Sun is north of the equator, we will ADD the declination of the Sun on the current day to the angle γ to arrive at the current Latitude of our location. Likewise, if one is south of the equator and the declination of the Sun is south of the equator, we will ADD the angle of declination to γ to arrive at the local Latitude.
If one is north of the equator and the declination of the Sun is south of the equator, then we subtract one from the other to arrive at our local Latitude.
And vice-versa. Subtract if our location is on the opposite side of the equator than the declination of the Sun.
Taking my example from an earlier post:
Height of Post P = 9 and Length of line TN = 4.5.
4.5 divided by 9 = .5
The angle Gamma (γ) = The Arctangent of .5 = 26.56505118°
The Declination of the Sun on that day (July 18th) was 21 degrees 08 minutes or 21.13333°
As both my Location and the Declination of the Sun are North of the equator, we add the Declination 21.13333° to Gamma (γ) 26.56505118° to arrive at 47.69838° = 47° 41' 54.17" North Latitude
BDH
bdhfe- Posts : 77
Points : 1896
Reputation : 6
Join date : 2019-08-12
 Re: Mapping the Earth
Re: Mapping the Earth
Another example using leotruther's data with Version 3.0 of Finding Latitude
The data:
Date of calculation = Aug 5th
Sun declination = N 17" 8'
Height of Post = 30 cm
Length of line = 25.4 cm
-------------
The angle gamma (γ) = arctan( 25.4 / 30 ) = 40.25458926°
The Sun's Declination is 17 degrees 8 minutes = 17.13333° North
As leotruther is South of the equator and the Sun's declnation is North of the equator, we will subtract the Declination from the angle gamma (γ)
So, 40.25458926° minus 17.13333° = 23.12128926 = 23° 7' 16.64" South Latitude
BDH
The data:
Date of calculation = Aug 5th
Sun declination = N 17" 8'
Height of Post = 30 cm
Length of line = 25.4 cm
-------------
The angle gamma (γ) = arctan( 25.4 / 30 ) = 40.25458926°
The Sun's Declination is 17 degrees 8 minutes = 17.13333° North
As leotruther is South of the equator and the Sun's declnation is North of the equator, we will subtract the Declination from the angle gamma (γ)
So, 40.25458926° minus 17.13333° = 23.12128926 = 23° 7' 16.64" South Latitude
BDH
bdhfe- Posts : 77
Points : 1896
Reputation : 6
Join date : 2019-08-12
 Re: Mapping the Earth
Re: Mapping the Earth
I would like to make a small digression in order to provide a very practical reason why mapping the Earth from a Flat Earth perspective is very important.
First, here is a handful of experiences, the source of which I used to know (LOL) but at present cannot recall.
35) If the Earth were truly a globe, then every line of latitude south of the equator would have to measure a gradually smaller and smaller circumference the farther South travelled. If, however, the Earth is an extended plane, then every line of latitude south of the equator should measure a gradually larger and larger circumference the farther South travelled. The fact that many captains navigating south of the equator assuming the globular theory have found themselves drastically out of reckoning, moreso the farther South travelled, testifies to the fact that the Earth is not a ball.
36) During Captain James Clark Ross’s voyages around the Antarctic circumference, he often wrote in his journal perplexed at how they routinely found themselves out of accordance with their charts
37) Lieutenant Charles Wilkes commanded a United States Navy exploration expedition to the Antarctic from 1838 to 1842, and in his journals also mentioned being consistently east of his reckoning, sometimes over 20 miles in less than 18 hours.
38) To quote Reverend Thomas Milner, “In the southern hemisphere, navigators to India have often fancied themselves east of the Cape when still west, and have been driven ashore on the African coast, which, according to their reckoning, lay behind them.
39) Practical distance measurements taken from “The Australian Handbook, Almanack, Shippers’ and Importers’ Directory” state that the straight line distance between Sydney and Nelson is 1550 statute miles. Their given difference in longitude is 22 degrees 2’14”. Therefore if 22 degrees 2’14” out of 360 is 1550 miles, the entirety would measure 25,182 miles. This is not only larger than the ball-Earth is said to be at the equator, but a whole 4262 miles greater than it would be at Sydney’s southern latitude on a globe of said proportions.
40) From near Cape Horn, Chile to Port Philip in Melbourne, Australia the distance is 10,500 miles, or 143 degrees of longitude away. Factoring in the remaining degrees to 360 makes for a total distance of 26,430 miles around this particular latitude, which is over 1500 miles wider than Earth is supposed to be at the equator, and many more thousands of miles wider than it is supposed to be at such Southern latitudes.
41) Similar calculations made from the Cape of Good Hope, South Africa to Melbourne, Australia at an average latitude of 35.5 degrees South, have given an approximate figure of over 25,000 miles, which is again equal to or greater than the Earth’s supposed greatest circumference at the equator. Calculations from Sydney, Australia to Wellington, New Zealand at an average of 37.5 degrees South have given an approximate circumference of 25,500 miles, greater still! According to the ball-Earth theory, the circumference of the Earth at 37.5 degrees Southern latitude should be only 19,757 statute miles, almost six thousand miles less than such practical measurements.
42) In the ball-Earth model Antarctica is an ice continent which covers the bottom of the ball from 78 degrees South latitude to 90 and is therefore not more than 12,000 miles in circumference. Many early explorers including Captain Cook and James Clark Ross, however, in attempting Antarctic circumnavigation took 3 to 4 years and clocked 50-60,000 miles around. The British ship Challenger also made an indirect but complete circumnavigation of Antarctica traversing 69,000 miles. This is entirely inconsistent with the ball model.
One other quick example: An acquaintance of mine who is a submariner in the US Navy became a Flat Earther after experiencing these absurdities in distances in the Southern Seas on board a submarine.
Summarizing, ALL of these examples illustrates a very important point. The distances calculated by the Greece Ballers for the Southern latitudes are completely absurd. By compressing the distance between degrees of Longitude on the Globe, the distances become more and more meaningless as one travels further South. Instead, as we know from the Flat Earth, the distance between degrees of Longitude increase as we traverse South.
Secondly, the failure of the Globe model to accurately provide good bearings in the South, translates to a skewing or EXTORTION (Gleason's own word) of the land masses on Gleason Map since Gleason essentially used the data provided him by the Freemasons at the Royal Observatory in Greenwich, England.
This mapping project will necessarily correct the land mass distortions in Gleason's and provide a very real and geographically correct view of Earth.
Consequently, once we have a fairly complete map of the world AS IT REALLY IS, we can provide the US Navy, at least, and international airlines as well, a proper and true picture of the land and sea they travel in and over.
BDH
First, here is a handful of experiences, the source of which I used to know (LOL) but at present cannot recall.
35) If the Earth were truly a globe, then every line of latitude south of the equator would have to measure a gradually smaller and smaller circumference the farther South travelled. If, however, the Earth is an extended plane, then every line of latitude south of the equator should measure a gradually larger and larger circumference the farther South travelled. The fact that many captains navigating south of the equator assuming the globular theory have found themselves drastically out of reckoning, moreso the farther South travelled, testifies to the fact that the Earth is not a ball.
36) During Captain James Clark Ross’s voyages around the Antarctic circumference, he often wrote in his journal perplexed at how they routinely found themselves out of accordance with their charts
37) Lieutenant Charles Wilkes commanded a United States Navy exploration expedition to the Antarctic from 1838 to 1842, and in his journals also mentioned being consistently east of his reckoning, sometimes over 20 miles in less than 18 hours.
38) To quote Reverend Thomas Milner, “In the southern hemisphere, navigators to India have often fancied themselves east of the Cape when still west, and have been driven ashore on the African coast, which, according to their reckoning, lay behind them.
39) Practical distance measurements taken from “The Australian Handbook, Almanack, Shippers’ and Importers’ Directory” state that the straight line distance between Sydney and Nelson is 1550 statute miles. Their given difference in longitude is 22 degrees 2’14”. Therefore if 22 degrees 2’14” out of 360 is 1550 miles, the entirety would measure 25,182 miles. This is not only larger than the ball-Earth is said to be at the equator, but a whole 4262 miles greater than it would be at Sydney’s southern latitude on a globe of said proportions.
40) From near Cape Horn, Chile to Port Philip in Melbourne, Australia the distance is 10,500 miles, or 143 degrees of longitude away. Factoring in the remaining degrees to 360 makes for a total distance of 26,430 miles around this particular latitude, which is over 1500 miles wider than Earth is supposed to be at the equator, and many more thousands of miles wider than it is supposed to be at such Southern latitudes.
41) Similar calculations made from the Cape of Good Hope, South Africa to Melbourne, Australia at an average latitude of 35.5 degrees South, have given an approximate figure of over 25,000 miles, which is again equal to or greater than the Earth’s supposed greatest circumference at the equator. Calculations from Sydney, Australia to Wellington, New Zealand at an average of 37.5 degrees South have given an approximate circumference of 25,500 miles, greater still! According to the ball-Earth theory, the circumference of the Earth at 37.5 degrees Southern latitude should be only 19,757 statute miles, almost six thousand miles less than such practical measurements.
42) In the ball-Earth model Antarctica is an ice continent which covers the bottom of the ball from 78 degrees South latitude to 90 and is therefore not more than 12,000 miles in circumference. Many early explorers including Captain Cook and James Clark Ross, however, in attempting Antarctic circumnavigation took 3 to 4 years and clocked 50-60,000 miles around. The British ship Challenger also made an indirect but complete circumnavigation of Antarctica traversing 69,000 miles. This is entirely inconsistent with the ball model.
One other quick example: An acquaintance of mine who is a submariner in the US Navy became a Flat Earther after experiencing these absurdities in distances in the Southern Seas on board a submarine.
Summarizing, ALL of these examples illustrates a very important point. The distances calculated by the Greece Ballers for the Southern latitudes are completely absurd. By compressing the distance between degrees of Longitude on the Globe, the distances become more and more meaningless as one travels further South. Instead, as we know from the Flat Earth, the distance between degrees of Longitude increase as we traverse South.
Secondly, the failure of the Globe model to accurately provide good bearings in the South, translates to a skewing or EXTORTION (Gleason's own word) of the land masses on Gleason Map since Gleason essentially used the data provided him by the Freemasons at the Royal Observatory in Greenwich, England.
This mapping project will necessarily correct the land mass distortions in Gleason's and provide a very real and geographically correct view of Earth.
Consequently, once we have a fairly complete map of the world AS IT REALLY IS, we can provide the US Navy, at least, and international airlines as well, a proper and true picture of the land and sea they travel in and over.
BDH
bdhfe- Posts : 77
Points : 1896
Reputation : 6
Join date : 2019-08-12
 Re: Mapping the Earth
Re: Mapping the Earth
The following is from this website:
Distances in Southern Hemisphere
I quote:
Nowadays there is some support from science that in fact the southern part of the “globe” is larger than the northern part and we have Neil De Grass Tyson declaring that the earth is “pear shaped”.
From Wikipedia:
Neil deGrasse Tyson (US: /dəˈɡræs/ or UK: /dəˈɡrɑːs/; born October 5, 1958) is an American astrophysicist, planetary scientist, author, and science communicator. Tyson studied at Harvard University, the University of Texas at Austin, and Columbia University. From 1991 to 1994, he was a postdoctoral research associate at Princeton University. In 1994, he joined the Hayden Planetarium as a staff scientist and the Princeton faculty as a visiting research scientist and lecturer. In 1996, he became director of the planetarium and oversaw its $210 million reconstruction project, which was completed in 2000. Since 1996, he has been the director of the Hayden Planetarium at the Rose Center for Earth and Space in New York City. The center is part of the American Museum of Natural History, where Tyson founded the Department of Astrophysics in 1997 and has been a research associate in the department since 2003.
From interestingengineering.com:
Neil deGrasse Tyson is a highly accomplished astrophysicist, planetary scientist, author, and something of a celebrity science educator to millions of people around the world.
Neil says: earth is pear shaped
NASA gives us:
Stunning image of Earth, taken by the DSCOVR Satellite

More Greece Ball nonsense provide by individuals and organizations that can't keep their stories straight.
As an aside, using a fish eye lens, I can make the fruit on my pear trees look just that NASA photo!!
Note: the reason Neil says the earth is pear shaped is because of Australia!!
And this is another good reason to provide the REAL picture of Earth -- to finally help the poor Australian figure out what the country looks like, AND WHERE IT REALLY RESIDES on Earth. :-)
They can then cast aside the Greece Ball propaganda, and free themselves from the imaginary strain of hanging by their toes!!
BDH
Distances in Southern Hemisphere
I quote:
Nowadays there is some support from science that in fact the southern part of the “globe” is larger than the northern part and we have Neil De Grass Tyson declaring that the earth is “pear shaped”.
From Wikipedia:
Neil deGrasse Tyson (US: /dəˈɡræs/ or UK: /dəˈɡrɑːs/; born October 5, 1958) is an American astrophysicist, planetary scientist, author, and science communicator. Tyson studied at Harvard University, the University of Texas at Austin, and Columbia University. From 1991 to 1994, he was a postdoctoral research associate at Princeton University. In 1994, he joined the Hayden Planetarium as a staff scientist and the Princeton faculty as a visiting research scientist and lecturer. In 1996, he became director of the planetarium and oversaw its $210 million reconstruction project, which was completed in 2000. Since 1996, he has been the director of the Hayden Planetarium at the Rose Center for Earth and Space in New York City. The center is part of the American Museum of Natural History, where Tyson founded the Department of Astrophysics in 1997 and has been a research associate in the department since 2003.
From interestingengineering.com:
Neil deGrasse Tyson is a highly accomplished astrophysicist, planetary scientist, author, and something of a celebrity science educator to millions of people around the world.
Neil says: earth is pear shaped
NASA gives us:
Stunning image of Earth, taken by the DSCOVR Satellite

More Greece Ball nonsense provide by individuals and organizations that can't keep their stories straight.
As an aside, using a fish eye lens, I can make the fruit on my pear trees look just that NASA photo!!
Note: the reason Neil says the earth is pear shaped is because of Australia!!
And this is another good reason to provide the REAL picture of Earth -- to finally help the poor Australian figure out what the country looks like, AND WHERE IT REALLY RESIDES on Earth. :-)
They can then cast aside the Greece Ball propaganda, and free themselves from the imaginary strain of hanging by their toes!!
BDH
bdhfe- Posts : 77
Points : 1896
Reputation : 6
Join date : 2019-08-12
 Re: Mapping the Earth
Re: Mapping the Earth
And speaking of Australia.....
Sorry, one more side track to illustrate the importance of this project.
In the United States, here are the Time Zones. The United States is North of the Equator and the lines of Longitude diverge at one progresses South.
See how the Time Zones follow this:
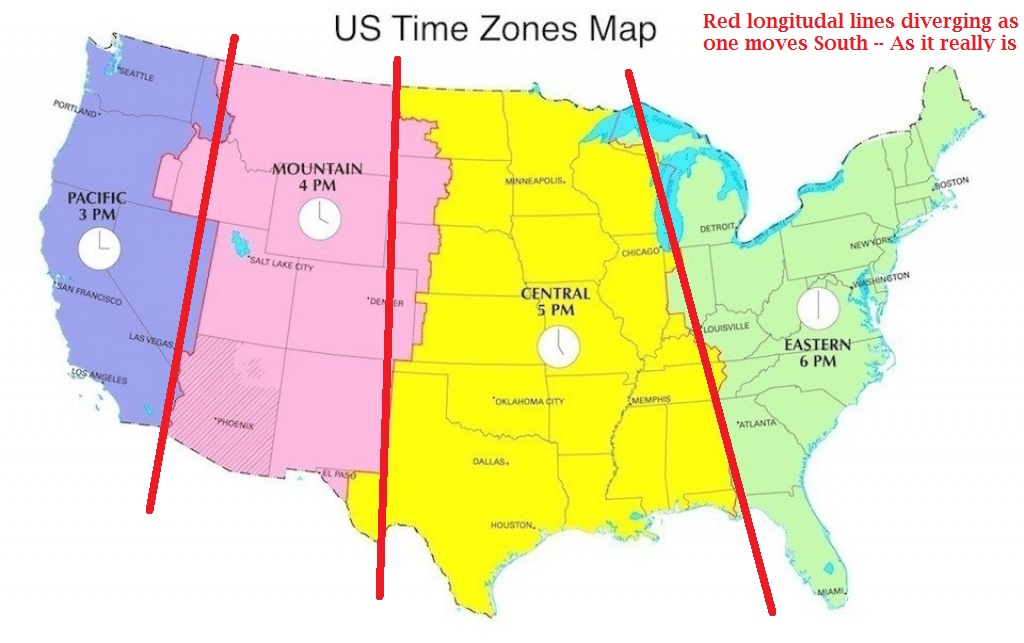
Now, contrast this with Australia. Australia is in the Southern latitudes, and of course, based on the Globe theory, the lines of Longitude should Converge as one moves South. However, see this:

The opposite is obviously true as there are more time zones in southern Australia than there are in the northern part of the country.
When the time comes, and it is coming, our mapping project will shed the light on what is really going on in the Land NOT down under.
BDH
Sorry, one more side track to illustrate the importance of this project.
In the United States, here are the Time Zones. The United States is North of the Equator and the lines of Longitude diverge at one progresses South.
See how the Time Zones follow this:

Now, contrast this with Australia. Australia is in the Southern latitudes, and of course, based on the Globe theory, the lines of Longitude should Converge as one moves South. However, see this:

The opposite is obviously true as there are more time zones in southern Australia than there are in the northern part of the country.
When the time comes, and it is coming, our mapping project will shed the light on what is really going on in the Land NOT down under.
BDH
bdhfe- Posts : 77
Points : 1896
Reputation : 6
Join date : 2019-08-12
Hauchfer and tycho_brahe like this post
 Re: Mapping the Earth
Re: Mapping the Earth
BDH,
How much data will you need before you can start making your first map? Will this take years?
How much data will you need before you can start making your first map? Will this take years?

Hauchfer- Posts : 15
Points : 1482
Reputation : 2
Join date : 2020-07-23
tycho_brahe likes this post
 Re: Mapping the Earth
Re: Mapping the Earth
Hi Hauchfer,
Years? LOL I hope not.
I figure we have most the information we need based on maps already in existence.
Our project will need the fundamentals of Latitude and Longitude to place the continents in their correct orientation and size.
We know, based on experience, that the globe calculations for Latitude and Longitude are incorrect.
The question is: which ones, and how much are the calcs wrong for any given land mass?
So, the scheme of this exercise/project is to confirm the correctness of some and show the incorrectness of others, since we know that the Earth is Flat and the devils have been distorting it into something unrecognizable. That distortion is what we want to ERADICATE.
And, depending on how many mappers are involved and where they reside, the project will take that long.
Any given mapper can perform the operation in two days, and if they are into travelling, they can perform the two day operation with their handy dandy travel kit. [Travel Kit not yet available ]
]
We know there are Flat Earthers on every continent in the world, so the amount of individual effort should be minimal as well.
BDH
Years? LOL I hope not.
I figure we have most the information we need based on maps already in existence.
Our project will need the fundamentals of Latitude and Longitude to place the continents in their correct orientation and size.
We know, based on experience, that the globe calculations for Latitude and Longitude are incorrect.
The question is: which ones, and how much are the calcs wrong for any given land mass?
So, the scheme of this exercise/project is to confirm the correctness of some and show the incorrectness of others, since we know that the Earth is Flat and the devils have been distorting it into something unrecognizable. That distortion is what we want to ERADICATE.
And, depending on how many mappers are involved and where they reside, the project will take that long.
Any given mapper can perform the operation in two days, and if they are into travelling, they can perform the two day operation with their handy dandy travel kit. [Travel Kit not yet available
We know there are Flat Earthers on every continent in the world, so the amount of individual effort should be minimal as well.
BDH
bdhfe- Posts : 77
Points : 1896
Reputation : 6
Join date : 2019-08-12
Hauchfer likes this post
 Re: Mapping the Earth
Re: Mapping the Earth
It is September 1st and I have performed another Latitude test using Finding Latitude Version 3.0.
My numbers are:
TN = 7.563 inches
P = 9.250 inches
Sun's Declination = 8.5 degrees
Angle gamma (γ) = Arctan(TN/P) = 39.269 degrees
My Latitude = 8.5 + 39.269 = 47.769 degree OR 47° 46' 8.4" North Latitude
Comparing this to the Latitude calculated from July 18th -- 47° 41' 54.17" North Latitude
I am within 5 minutes of a degree difference!!
This is a very acceptable margin of error considering my setup uses a wooden table, some cardboard, a standard size ruler, and a No. 2 pencil.
Tomorrow, my Longitude calculation.
BDH
My numbers are:
TN = 7.563 inches
P = 9.250 inches
Sun's Declination = 8.5 degrees
Angle gamma (γ) = Arctan(TN/P) = 39.269 degrees
My Latitude = 8.5 + 39.269 = 47.769 degree OR 47° 46' 8.4" North Latitude
Comparing this to the Latitude calculated from July 18th -- 47° 41' 54.17" North Latitude
I am within 5 minutes of a degree difference!!
This is a very acceptable margin of error considering my setup uses a wooden table, some cardboard, a standard size ruler, and a No. 2 pencil.
Tomorrow, my Longitude calculation.
BDH
bdhfe- Posts : 77
Points : 1896
Reputation : 6
Join date : 2019-08-12
Hauchfer and tycho_brahe like this post
 Re: Mapping the Earth
Re: Mapping the Earth
Just a suggestion - using a larger pole would reduce any measurement errors.
RedorBlue- Posts : 97
Points : 2304
Reputation : 38
Join date : 2018-08-19
 Re: Mapping the Earth
Re: Mapping the Earth
For Finding Longitude Version 2.0 on September 3 (I missed the 2nd)
The Sun crossed the Zenith at 12:47:10 pm
I am 7 hours West of Greenwich Meridian
Analemma chart shows that the Sun is about 15 seconds fast to the Zenith today.
Hence my local Longitude comes to
116 ° 50' 59" W Longitude.
My previous Longitude calculation on July 20 gave me
116° 47' 13.2" W Longitude.
Which is about 3.75 minutes difference between the two.
BDH
The Sun crossed the Zenith at 12:47:10 pm
I am 7 hours West of Greenwich Meridian
Analemma chart shows that the Sun is about 15 seconds fast to the Zenith today.
Hence my local Longitude comes to
116 ° 50' 59" W Longitude.
My previous Longitude calculation on July 20 gave me
116° 47' 13.2" W Longitude.
Which is about 3.75 minutes difference between the two.
BDH
bdhfe- Posts : 77
Points : 1896
Reputation : 6
Join date : 2019-08-12
tycho_brahe likes this post
 Re: Mapping the Earth
Re: Mapping the Earth
RedorBlue,
I agree, a larger setup i.e. a taller Post (P), will definitely improve accuracy in both Latitude and Longitude.
Regards,
BDH
I agree, a larger setup i.e. a taller Post (P), will definitely improve accuracy in both Latitude and Longitude.
Regards,
BDH
bdhfe- Posts : 77
Points : 1896
Reputation : 6
Join date : 2019-08-12
 Re: Mapping the Earth
Re: Mapping the Earth
I like your enthusiasm and all but I do not understand what your problem is with the gleason map? "It Is" exact and "It Is" precise. I've inserted a blown up hi res version of the map into a geometry program and fitted the map precisely to the exact degrees (x / y axis and 90° being equator). I can plot the coordinates of any location on earth and I can tell you ~ it is precise!! Right down to the second.. it doesn't get any better than that. You can figure out the exact time and distance of any location from any given location. That being said.. there is way more to that map thats NOT being noticed or understood in my opinion. I've been studying it and rackin my brain on and off for the last five years. People just look at it and pass it off, without appreciating the detailed information it provides. It's a shame.
TG123- Posts : 3
Points : 1769
Reputation : 0
Join date : 2019-09-26
StillWakingUp likes this post
 Re: Mapping the Earth
Re: Mapping the Earth
Hi TLG123,
As part of the mapping project, I am using the Gleason map. So, I am not dismissing it and its usefulness. I would like to ask you some questions in a follow up post regarding how you measure the "distance of any location from any given location".
For this post, I am going to give my reasons for why the Gleason map is not presenting the world's land masses South of the Equator reasonably.
1. In Gleason's patent Letter Specifications for Patent No. 497,917, dated May 23, 1893 it is stated:
"The map is not so extorted as to lose the relative latitude and longitude of any places on the land or sea, but retains all latitudes and longitudes of places agreeing with other recognized authors; and as the proper relations of continents and countries all stand in their relative position to each other, they are thus impressed upon the mind of the student. The extorsion of the map from that of a globe consists, mainly in the straightening out of the meridian lines allowing each to retain their original value from Greenwich, the equator to the two poles."
My comment: Gleason is saying that the map is extorted, since he straightened the meridian lines, which the globe theory curves from North pole to South pole. This tells me that he is using the globe theories values for latitude and longitude.
2. I posted earlier some snippets from the book Why the Earth is not a Globe:
Here are some of those snippets:
"
36) During Captain James Clark Ross’s voyages around the Antarctic circumference, he often wrote in his journal perplexed at how they routinely found themselves out of accordance with their charts
37) Lieutenant Charles Wilkes commanded a United States Navy exploration expedition to the Antarctic from 1838 to 1842, and in his journals also mentioned being consistently east of his reckoning, sometimes over 20 miles in less than 18 hours.
38) To quote Reverend Thomas Milner, “In the southern hemisphere, navigators to India have often fancied themselves east of the Cape when still west, and have been driven ashore on the African coast, which, according to their reckoning, lay behind them.
"
These snippets describe issues of navigation South of the equator. In particular, looking at number 38 above, the navigators, going by the charts (likely originating from Greenwich) put the navigators further East than where they were actually located. If the charts said they were at 30 degrees East Longitude when in fact they were more like 15 degrees East Longitude, the charts were wrong and they were wrong by the calculation of LONGITUDE calculated by the Globe theory.
Note: This fact tells me that it is likely that Longitude in the South needs to be corrected to provide accuracy in navigation for the Southern climes. One cannot use the Globe calculations to get the accuracy. We need to supply new calcs based on Flat Earth Longitude calculations.
3. The Gleason map states at the top that it is based on the projection of J. S. Christopher, Modern College, Blackheath, England
There is no Modern College in Blackheath, but there is a Morden College in Blackheath, England.
J.S. Christopher was likely a resident at that place.
Secondly, Blackheath, England is a stone's throw from the Royal Observatory at Greenwich England, home to the Freemasons that gave us the Globe Lats and Longs we go by today.
Hence, it is highly probable that the Latitude and Longitude figures for locations on the Gleason map are Latitude and Longitude figures from Greenwich England.
And hence, the Extortion described in Gleason's Specifications in his Patent application.
Summarizing then, Gleason acknowledges using Globe theory calcs for Latitude and Longitude; the Southern areas from the Equator are where these calcs go completely wrong and get worse the further South one navigates.
Three more snippets from my earlier post:
9) Practical distance measurements taken from “The Australian Handbook, Almanack, Shippers’ and Importers’ Directory” state that the straight line distance between Sydney and Nelson is 1550 statute miles. Their given difference in longitude is 22 degrees 2’14”. Therefore if 22 degrees 2’14” out of 360 is 1550 miles, the entirety would measure 25,182 miles. This is not only larger than the ball-Earth is said to be at the equator, but a whole 4262 miles greater than it would be at Sydney’s southern latitude on a globe of said proportions.
40) From near Cape Horn, Chile to Port Philip in Melbourne, Australia the distance is 10,500 miles, or 143 degrees of longitude away. Factoring in the remaining degrees to 360 makes for a total distance of 26,430 miles around this particular latitude, which is over 1500 miles wider than Earth is supposed to be at the equator, and many more thousands of miles wider than it is supposed to be at such Southern latitudes.
41) Similar calculations made from the Cape of Good Hope, South Africa to Melbourne, Australia at an average latitude of 35.5 degrees South, have given an approximate figure of over 25,000 miles, which is again equal to or greater than the Earth’s supposed greatest circumference at the equator. Calculations from Sydney, Australia to Wellington, New Zealand at an average of 37.5 degrees South have given an approximate circumference of 25,500 miles, greater still! According to the ball-Earth theory, the circumference of the Earth at 37.5 degrees Southern latitude should be only 19,757 statute miles, almost six thousand miles less than such practical measurements.
Now, these are practical distance measurements. What distance does the Globe theory provide for each above?
Likely something different? Correct?
So, what is wrong then? We know on the Flat Earth, that distance between degrees of Longitude become greater and greater and one progresses further South, as opposed to the Globe theory where degrees of Longitude become smaller and smaller as they progress further South.
What is wrong is the mileage and it is based on the Longitude being INCORRECT. Maybe Latitude to some degree (no pun intended) but mostly Longitude.
Hence the purpose of this mapping project.
BDH
As part of the mapping project, I am using the Gleason map. So, I am not dismissing it and its usefulness. I would like to ask you some questions in a follow up post regarding how you measure the "distance of any location from any given location".
For this post, I am going to give my reasons for why the Gleason map is not presenting the world's land masses South of the Equator reasonably.
1. In Gleason's patent Letter Specifications for Patent No. 497,917, dated May 23, 1893 it is stated:
"The map is not so extorted as to lose the relative latitude and longitude of any places on the land or sea, but retains all latitudes and longitudes of places agreeing with other recognized authors; and as the proper relations of continents and countries all stand in their relative position to each other, they are thus impressed upon the mind of the student. The extorsion of the map from that of a globe consists, mainly in the straightening out of the meridian lines allowing each to retain their original value from Greenwich, the equator to the two poles."
My comment: Gleason is saying that the map is extorted, since he straightened the meridian lines, which the globe theory curves from North pole to South pole. This tells me that he is using the globe theories values for latitude and longitude.
2. I posted earlier some snippets from the book Why the Earth is not a Globe:
Here are some of those snippets:
"
36) During Captain James Clark Ross’s voyages around the Antarctic circumference, he often wrote in his journal perplexed at how they routinely found themselves out of accordance with their charts
37) Lieutenant Charles Wilkes commanded a United States Navy exploration expedition to the Antarctic from 1838 to 1842, and in his journals also mentioned being consistently east of his reckoning, sometimes over 20 miles in less than 18 hours.
38) To quote Reverend Thomas Milner, “In the southern hemisphere, navigators to India have often fancied themselves east of the Cape when still west, and have been driven ashore on the African coast, which, according to their reckoning, lay behind them.
"
These snippets describe issues of navigation South of the equator. In particular, looking at number 38 above, the navigators, going by the charts (likely originating from Greenwich) put the navigators further East than where they were actually located. If the charts said they were at 30 degrees East Longitude when in fact they were more like 15 degrees East Longitude, the charts were wrong and they were wrong by the calculation of LONGITUDE calculated by the Globe theory.
Note: This fact tells me that it is likely that Longitude in the South needs to be corrected to provide accuracy in navigation for the Southern climes. One cannot use the Globe calculations to get the accuracy. We need to supply new calcs based on Flat Earth Longitude calculations.
3. The Gleason map states at the top that it is based on the projection of J. S. Christopher, Modern College, Blackheath, England
There is no Modern College in Blackheath, but there is a Morden College in Blackheath, England.
J.S. Christopher was likely a resident at that place.
Secondly, Blackheath, England is a stone's throw from the Royal Observatory at Greenwich England, home to the Freemasons that gave us the Globe Lats and Longs we go by today.
Hence, it is highly probable that the Latitude and Longitude figures for locations on the Gleason map are Latitude and Longitude figures from Greenwich England.
And hence, the Extortion described in Gleason's Specifications in his Patent application.
Summarizing then, Gleason acknowledges using Globe theory calcs for Latitude and Longitude; the Southern areas from the Equator are where these calcs go completely wrong and get worse the further South one navigates.
Three more snippets from my earlier post:
9) Practical distance measurements taken from “The Australian Handbook, Almanack, Shippers’ and Importers’ Directory” state that the straight line distance between Sydney and Nelson is 1550 statute miles. Their given difference in longitude is 22 degrees 2’14”. Therefore if 22 degrees 2’14” out of 360 is 1550 miles, the entirety would measure 25,182 miles. This is not only larger than the ball-Earth is said to be at the equator, but a whole 4262 miles greater than it would be at Sydney’s southern latitude on a globe of said proportions.
40) From near Cape Horn, Chile to Port Philip in Melbourne, Australia the distance is 10,500 miles, or 143 degrees of longitude away. Factoring in the remaining degrees to 360 makes for a total distance of 26,430 miles around this particular latitude, which is over 1500 miles wider than Earth is supposed to be at the equator, and many more thousands of miles wider than it is supposed to be at such Southern latitudes.
41) Similar calculations made from the Cape of Good Hope, South Africa to Melbourne, Australia at an average latitude of 35.5 degrees South, have given an approximate figure of over 25,000 miles, which is again equal to or greater than the Earth’s supposed greatest circumference at the equator. Calculations from Sydney, Australia to Wellington, New Zealand at an average of 37.5 degrees South have given an approximate circumference of 25,500 miles, greater still! According to the ball-Earth theory, the circumference of the Earth at 37.5 degrees Southern latitude should be only 19,757 statute miles, almost six thousand miles less than such practical measurements.
Now, these are practical distance measurements. What distance does the Globe theory provide for each above?
Likely something different? Correct?
So, what is wrong then? We know on the Flat Earth, that distance between degrees of Longitude become greater and greater and one progresses further South, as opposed to the Globe theory where degrees of Longitude become smaller and smaller as they progress further South.
What is wrong is the mileage and it is based on the Longitude being INCORRECT. Maybe Latitude to some degree (no pun intended) but mostly Longitude.
Hence the purpose of this mapping project.
BDH
bdhfe- Posts : 77
Points : 1896
Reputation : 6
Join date : 2019-08-12
 Re: Mapping the Earth
Re: Mapping the Earth
I guess I could have summarized the whole of my last post by simply saying:
If Gleason straightened out the meridians used by Greenwich to get his map, and if Greenwich is wrong in their Latitudes and Longitudes, then Gleason straightened out erroneous meridians South of the Equator.
Therefore, let us straighten out correct meridians South of the Equator.
Note: This is not to say that all the meridians South of the equator are wrong, because if they were, the Globe Earther's game would be kaput. The erroneous ones are being kept from popular places like Rio de Janeiro for example. If I had to guess, if one took a trip up to the source of the Amazon River, one would get some wild differences between the GE Longitudes and the FE Longitudes.
Speaking of which, while I took at look at Australia a few posts ago, I think it is time to look at Brazil.
BDH
If Gleason straightened out the meridians used by Greenwich to get his map, and if Greenwich is wrong in their Latitudes and Longitudes, then Gleason straightened out erroneous meridians South of the Equator.
Therefore, let us straighten out correct meridians South of the Equator.
Note: This is not to say that all the meridians South of the equator are wrong, because if they were, the Globe Earther's game would be kaput. The erroneous ones are being kept from popular places like Rio de Janeiro for example. If I had to guess, if one took a trip up to the source of the Amazon River, one would get some wild differences between the GE Longitudes and the FE Longitudes.
Speaking of which, while I took at look at Australia a few posts ago, I think it is time to look at Brazil.
BDH
bdhfe- Posts : 77
Points : 1896
Reputation : 6
Join date : 2019-08-12
 Re: Mapping the Earth
Re: Mapping the Earth
Hi, BDHFE
Thanks for the reply. This is a quick reply but with respect to the gleason map, 1° is equal to 60 nautical miles or rather 69.36 statute miles. When i say distance between 2 points, I'm referring to the distance as the "crow flies". To verify this, one can only take 2 points along the same longitude.. and measure. Once you have that distance, you can verify it by cross referencing it with their coordinates. I believe what you're not taking into account with your examples is that you are measuring a distance that is on the same latitude. However, even though the 2 points are on the same latitude, that does not equate to a straight line. A straight line would give considerably less distance than shown but if you account for the arc on the same latitude, the distance would be much greater. For example; you're stating a distance of 25000 miles between cape of good hope and melbourne Aus. Now without putting in the precise coordinates of each place, i went to my map and measured from the 2 points on map and i got 15587 miles (as the crow flies ~ straight line). If you measure on the same latitude with the arc into consideration, you get a much greater distance. Also the further south you go, the larger the arc. "They" state the circumference of earth is approx. 24900 miles.. Gleason says 1° = 69.36 miles in which 360° = 24969.6 miles.
I get what you're saying.. a 2d map is distorted and not representative of how it is. Obviously Australia is not larger than Canada but VISUALLY on the map it is. If you look at the lines of longitude, you will see more lines run through Canada than Australia making Canada larger even though it "appears" smaller. The problem in the mapping is we are trying to map celestial points in relation to land mass.. and when it comes to a 2d map there is no other way, other than to converge the longitude lines to the center point. I dunno if that makes sense or not, I'm not the best at articulating my thoughts..
**Edit: Elevation is a variable that plays a big factor in which you cannot display on a 2d map.
Thanks for the reply. This is a quick reply but with respect to the gleason map, 1° is equal to 60 nautical miles or rather 69.36 statute miles. When i say distance between 2 points, I'm referring to the distance as the "crow flies". To verify this, one can only take 2 points along the same longitude.. and measure. Once you have that distance, you can verify it by cross referencing it with their coordinates. I believe what you're not taking into account with your examples is that you are measuring a distance that is on the same latitude. However, even though the 2 points are on the same latitude, that does not equate to a straight line. A straight line would give considerably less distance than shown but if you account for the arc on the same latitude, the distance would be much greater. For example; you're stating a distance of 25000 miles between cape of good hope and melbourne Aus. Now without putting in the precise coordinates of each place, i went to my map and measured from the 2 points on map and i got 15587 miles (as the crow flies ~ straight line). If you measure on the same latitude with the arc into consideration, you get a much greater distance. Also the further south you go, the larger the arc. "They" state the circumference of earth is approx. 24900 miles.. Gleason says 1° = 69.36 miles in which 360° = 24969.6 miles.
I get what you're saying.. a 2d map is distorted and not representative of how it is. Obviously Australia is not larger than Canada but VISUALLY on the map it is. If you look at the lines of longitude, you will see more lines run through Canada than Australia making Canada larger even though it "appears" smaller. The problem in the mapping is we are trying to map celestial points in relation to land mass.. and when it comes to a 2d map there is no other way, other than to converge the longitude lines to the center point. I dunno if that makes sense or not, I'm not the best at articulating my thoughts..
**Edit: Elevation is a variable that plays a big factor in which you cannot display on a 2d map.
TG123- Posts : 3
Points : 1769
Reputation : 0
Join date : 2019-09-26
 Re: Mapping the Earth
Re: Mapping the Earth
I'm not sure what you're trying to say here..
"If Gleason straightened out the meridians used by Greenwich to get his map, and if Greenwich is wrong in their Latitudes and Longitudes, then Gleason straightened out erroneous meridians South of the Equator.
Therefore, let us straighten out correct meridians South of the Equator."
The Prime meridian is the Greenwich Meridian or longitude, which is the fictitious y axis or 0 point. All lines of longitude are straight..? When you measure from a center point outward in a circle, its a given that the lines are going to be greater distance apart at the outer portion versus the center..? You have to get the globular version out of your head.
"If Gleason straightened out the meridians used by Greenwich to get his map, and if Greenwich is wrong in their Latitudes and Longitudes, then Gleason straightened out erroneous meridians South of the Equator.
Therefore, let us straighten out correct meridians South of the Equator."
The Prime meridian is the Greenwich Meridian or longitude, which is the fictitious y axis or 0 point. All lines of longitude are straight..? When you measure from a center point outward in a circle, its a given that the lines are going to be greater distance apart at the outer portion versus the center..? You have to get the globular version out of your head.
TG123- Posts : 3
Points : 1769
Reputation : 0
Join date : 2019-09-26
 Re: Mapping the Earth
Re: Mapping the Earth
Hi TG123,
Re: Greenwich is wrong
When I say "Greenwich" is am referring to the system of coordinates devised by the Royal Observatory that is based on the Globe. And this includes Latitude and Longitude.
And excellent reference for this information is found in "The Greenwich Meridian" by Malin and Stott:
Re: Gleason's extorsion
From Gleason's Patent application:
"The extorsion of the map from that of a globe consists, mainly in the straightening out of the meridian lines allowing each to retain their original value from Greenwich, the equator to the two poles."
Gleason is using the values of Latitudes and Longitudes devised by Greenwich which is based on the Globe.
Re: Meridians
Meridians are the lines of Longitude that exist over our heads and which stretch from the North Pole to a spot on the Southern Ice Wall. which spot is unique to each meridian. These meridians are not based on the Globe idea but are very real as the Earth is a Flat plane that has a center point (the North Pole) from which all meridians of Longitude emanate.
BDH
Re: Greenwich is wrong
When I say "Greenwich" is am referring to the system of coordinates devised by the Royal Observatory that is based on the Globe. And this includes Latitude and Longitude.
And excellent reference for this information is found in "The Greenwich Meridian" by Malin and Stott:
Re: Gleason's extorsion
From Gleason's Patent application:
"The extorsion of the map from that of a globe consists, mainly in the straightening out of the meridian lines allowing each to retain their original value from Greenwich, the equator to the two poles."
Gleason is using the values of Latitudes and Longitudes devised by Greenwich which is based on the Globe.
Re: Meridians
Meridians are the lines of Longitude that exist over our heads and which stretch from the North Pole to a spot on the Southern Ice Wall. which spot is unique to each meridian. These meridians are not based on the Globe idea but are very real as the Earth is a Flat plane that has a center point (the North Pole) from which all meridians of Longitude emanate.
BDH
bdhfe- Posts : 77
Points : 1896
Reputation : 6
Join date : 2019-08-12
 Re: Mapping the Earth
Re: Mapping the Earth
TG123 wrote:I'm not sure what you're trying to say here..
"If Gleason straightened out the meridians used by Greenwich to get his map, and if Greenwich is wrong in their Latitudes and Longitudes, then Gleason straightened out erroneous meridians South of the Equator.
Therefore, let us straighten out correct meridians South of the Equator."
The Prime meridian is the Greenwich Meridian or longitude, which is the fictitious y axis or 0 point. All lines of longitude are straight..? When you measure from a center point outward in a circle, its a given that the lines are going to be greater distance apart at the outer portion versus the center..? You have to get the globular version out of your head.
Hi TG123,
Following up on your post in a little different way, let me address what you are saying so that there is no confusion in what I am saying.
1. Straighten out the correct meridians South of the Equator
What I am trying to say here is that the Latitude and Longitude associated with locations South of the Equator as calculated by the Royal Observatory are incorrect as they have made these calculations for locations South of the Equator based on a Globe.
We know by testimony from the past and present, that navigation in the Southern climes is not accurate in terms of distance between lines of longitude.
For example, travelling East from South America, when one thinks they have passed the Cape of Africa, they find that they have yet to reach the western side of Africa.
Who has provided these navigators with the LATs and LONGs for their navigation? The Royal Observatory. These folks are basing their calcs on a globe and so instead of correctly issuing LATs / LONGs based on DIVERGING meridians, they are doing just the opposite..
However, one can say that if I took a reading of the location at Rio of Janeiro, which someone on this thread did perform, it was very accurate and it jived with the data provided by the Royal Observatory. Would this not contradict what I just said above? No.
So, back to my point. I still say that the calcs for the Southern climes, as provided by the Royal Observatory are incorrect, EXCEPT for those areas that are fairly well populated. They are wrong when one is in the open ocean and in those parts of South America, Australia, and Africa that are less populated.
Take for example the LAT/LONG at Rio de Janeiro calculated in this thread by the method I provided. Very accurate at Rio, but I am theorizing that as one proceeds into the interior of Brazil, that the LAT/LONGs become more incorrect. Now, why do I say this? Because of the inaccurate shape and distances of the continent itself as shown on Gleason.
To re-iterate, the Royal Observatory is getting away with murder with their data based on the Globe by taking advantage of the fact that one will not be testing their numbers in the open ocean in the South, or in those parts of the interiors of the southern continents that are virtually unpopulated.
Let me clarify this last: There have been cases reported in the open ocean that testify to the inaccuracy of the data provided by the Royal Observatory, but does not get much air time in terms of news.
So, I think we are saying the same thing.... getting the Globe out of our head. We just need to get the Globe data out of Gleason, and we will have an accurate picture of the Earth. Inputting the Flat Earth data, via the calculation method provided in this Subject, into Gleason will adjust the continents South of the Equator to more accurate shapes and distances. Distances that will match what people every day experience who live on those continents..
BDH
bdhfe- Posts : 77
Points : 1896
Reputation : 6
Join date : 2019-08-12
 Re: Mapping the Earth
Re: Mapping the Earth
TG123 wrote:Hi, BDHFE
Thanks for the reply. This is a quick reply but with respect to the gleason map, 1° is equal to 60 nautical miles or rather 69.36 statute miles. When i say distance between 2 points, I'm referring to the distance as the "crow flies". To verify this, one can only take 2 points along the same longitude.. and measure. Once you have that distance, you can verify it by cross referencing it with their coordinates. I believe what you're not taking into account with your examples is that you are measuring a distance that is on the same latitude. However, even though the 2 points are on the same latitude, that does not equate to a straight line. A straight line would give considerably less distance than shown but if you account for the arc on the same latitude, the distance would be much greater. For example; you're stating a distance of 25000 miles between cape of good hope and melbourne Aus. Now without putting in the precise coordinates of each place, i went to my map and measured from the 2 points on map and i got 15587 miles (as the crow flies ~ straight line). If you measure on the same latitude with the arc into consideration, you get a much greater distance. Also the further south you go, the larger the arc. "They" state the circumference of earth is approx. 24900 miles.. Gleason says 1° = 69.36 miles in which 360° = 24969.6 miles.
I get what you're saying.. a 2d map is distorted and not representative of how it is. Obviously Australia is not larger than Canada but VISUALLY on the map it is. If you look at the lines of longitude, you will see more lines run through Canada than Australia making Canada larger even though it "appears" smaller. The problem in the mapping is we are trying to map celestial points in relation to land mass.. and when it comes to a 2d map there is no other way, other than to converge the longitude lines to the center point. I dunno if that makes sense or not, I'm not the best at articulating my thoughts..
**Edit: Elevation is a variable that plays a big factor in which you cannot display on a 2d map.
Hi TG123,
You make a great point here. Especially with respect to the difference between Straight Line and Arc lengths between points of Longitude at the same Latitude.
However, let's talk about Canada and Australia
As for Canada, 2900 miles across, and Australia, 2400 miles across, there should be some discussion about distance between lines of Longitude.
The Straight line distance between degrees of Longitude in Canada's Latitudes is much less than the Straight line distance between degrees of Longitude in the Latitudes of Australia. However, the Arc distances will proportionally be the same for both South and North as the curvature of Arc does not vary.
Degrees of Latitude are concentric circles and so there would be no variation in the Arc distance versus Straight Line distance between two points of Longitude at any Latitude in the world. I.E., the ratio between Arc distances and Straight Line distances on the same Latitude is the same everywhere.
So, the Arc distance will not explain fully the reason why the distortion exists on the the Two Dimensional map. If the Arc distance is proportionally the same in both North and South as the Straight Line distance, then the appearance that Australia is larger on the 2D map does not hold.
The appearance on the Gleason map that Australia is larger than Canada is based on the theory given by the Royal Observatory that the Earth is a Globe and lines of Longitude Converge as one proceeds further South of the Equator.
We know that lines of Longitude DIVERGE as one goes further South and so the major issue between Flat Earth and Globe South of the Equator is Latitude and Longitude calculation.
If the distance between the western most edge of Australia and the east coast is a little over 2400 miles, and the distance between degrees of Longitude are greater than the distance between degrees of Longitude in the northern hemisphere like in Canada where it is 2900 miles across from west to east, and if the Straight Line and Arc line distances are proportionally the same, then there is some problem with the latitude and longitude values being used to provide the location of places in the south.... Australia in this case.
This necessarily points to the fact that the values provided for Latitude and Longitude are not correct.
This is currently proven by the severe miscalculation of location reported by seafarers' testimony when travelling in the the southern latitudes.
I have a 2nd person testimony of this provided to me by an US Navy submariner.
BDH
bdhfe- Posts : 77
Points : 1896
Reputation : 6
Join date : 2019-08-12
TyrannicalSawdustRex likes this post
 Re: Mapping the Earth
Re: Mapping the Earth
I like this project very much and find it exciting how we can come to an exact map of the earth.
Unfortunately, I am a layman in this field, so just one question:
Independent of correct longitudes and latitudes, it should be possible to get correct (straight) distances for Australia or Canada, also in north / "south" direction.
With this, depending on the amount of data, a true-to-scale outline of the two areas could be drawn. And this outline could then be compared with the outline of the Gleason map, for example. Either Australia is as elongated as in Gleason's map, or not.
Am I reasonably correct?
Update:
The approach of the topic starter is a relatively simple method to determine the location on land surfaces.
I only know that sextants are used at sea. However, not whether they can also be used on land.
Nor do I know how the altitude is determined, whether for Mount Everest or Lake Baikal.
However, at least it has been done many times to determine the coastlines or individual coastal locations with the sextant.
With it the correct outlines of e.g. Australia can be determined, or am I wrong there?
Does the sextant also work correctly south of the equator?
Unfortunately, I am a layman in this field, so just one question:
Independent of correct longitudes and latitudes, it should be possible to get correct (straight) distances for Australia or Canada, also in north / "south" direction.
With this, depending on the amount of data, a true-to-scale outline of the two areas could be drawn. And this outline could then be compared with the outline of the Gleason map, for example. Either Australia is as elongated as in Gleason's map, or not.
Am I reasonably correct?
Update:
The approach of the topic starter is a relatively simple method to determine the location on land surfaces.
I only know that sextants are used at sea. However, not whether they can also be used on land.
Nor do I know how the altitude is determined, whether for Mount Everest or Lake Baikal.
However, at least it has been done many times to determine the coastlines or individual coastal locations with the sextant.
With it the correct outlines of e.g. Australia can be determined, or am I wrong there?
Does the sextant also work correctly south of the equator?

Dan-cer- Posts : 39
Points : 1382
Reputation : 0
Join date : 2020-11-28
Location : Germany
 Re: Mapping the Earth
Re: Mapping the Earth
Dan-cer wrote:I like this project very much and find it exciting how we can come to an exact map of the earth.
Unfortunately, I am a layman in this field, so just one question:
Independent of correct longitudes and latitudes, it should be possible to get correct (straight) distances for Australia or Canada, also in north / "south" direction.
With this, depending on the amount of data, a true-to-scale outline of the two areas could be drawn. And this outline could then be compared with the outline of the Gleason map, for example. Either Australia is as elongated as in Gleason's map, or not.
Am I reasonably correct?
Hi Dan-cer,
If there is another method that can provide the correct distances in both East-West and North-South, independent of Latitude and Longitude calcs, then this method would provide a complementary check on the method of calculations described in this subject.
BDH
bdhfe- Posts : 77
Points : 1896
Reputation : 6
Join date : 2019-08-12
 Re: Mapping the Earth
Re: Mapping the Earth
Dan-cer wrote:
Update:
The approach of the topic starter is a relatively simple method to determine the location on land surfaces.
I only know that sextants are used at sea. However, not whether they can also be used on land.
Nor do I know how the altitude is determined, whether for Mount Everest or Lake Baikal.
However, at least it has been done many times to determine the coastlines or individual coastal locations with the sextant.
With it the correct outlines of e.g. Australia can be determined, or am I wrong there?
Does the sextant also work correctly south of the equator?
Dan-cer,
Are you proposing the use of the sextant to aid in the mapping of the land masses?
My understanding is that Sextants will work on Land and Sea. And, as to the use of the sextant in the South, I do not find where it cannot be used in the South as well as the North in Daytime. Nighttime would necessitate the use of stars.
Dip and Refraction and Parallax
These variables come into play as a result of adjusting for distances to stars and altitudes.
There is a good explainer on these here:
http://www.siranah.de/html/sail040h.htm
As these variables are used, based on the belief of a globe earth and large distances to the stars, there is then brought into play a Nautical Almanac, of which I have the gravest suspicions. I believe the devil is in the details in this, as the Almanac is used to to Adjust what we are actually seeing to what they want us to see. IMO.
BDH
bdhfe- Posts : 77
Points : 1896
Reputation : 6
Join date : 2019-08-12
Page 3 of 5 •  1, 2, 3, 4, 5
1, 2, 3, 4, 5 
 Similar topics
Similar topics» Experiments We All Can Do
» The Earth Plane (Flat Earth Children's Book)
» Flat Earth Audiobooks and PDFs
» Eric Dubay and IFERS Banned From the Internet
» Promoting Flat Earth
» The Earth Plane (Flat Earth Children's Book)
» Flat Earth Audiobooks and PDFs
» Eric Dubay and IFERS Banned From the Internet
» Promoting Flat Earth
IFERS - Exposing the 'Global' Conspiracy From Atlantis to Zion :: Reference Materials, eBooks, Articles, Videos, Maps
Page 3 of 5
Permissions in this forum:
You cannot reply to topics in this forum|
|
|
