Mapping the Earth

Waiting for instructions on how to calculate my "BDH" position.

I am in Rio, Brazil

GPS: Latitude -22.9201 / Longitude -43.3307

Thanks leotruther!!
I will put out the steps within a day or so.
BDH

The Process of calculating Latitude and Longitude on a Flat Earth occurs by performing a number of steps over two days.
Note: These steps will be supplemented with photos and illustrations as the particular step may require.

Assumptions:
1.  The Sun rotates around the center point of the Earth -- the North Pole -- once every 24 hours.
2.  The Sun is at its most northern point on June 21st -- at 23.44 degrees North latitude
3.  The Sun is at its most southern  point on December 21st -- at 23.44 degrees South latitude
4.  The Sun is at the Equator on March 21 and September 21 -- 0 degrees latitude
5.  The Earth is Flat.


Day One
1.  Find a level area or make one.  The shadow you will be employing will be distorted on a slanted surface.
I used a table and covered it with a sheet of card board making sure the table was perfectly level.



2.  Set a post perpendicular (P) to the level surface you are using.


Find True North
3.  On day One, being a sunny day of course, start tracking the shadow of the post as it makes its way across the level surface (S) you have set up. (in my case the cardboard on the level table).  Starting about 11:00AM local time begin marking, with pen or pencil, the spot on the surface where the top of the post shadow ends.  Continue marking the surface where the top of the shadow ends every 10-20 minutes or so until about 1:30PM local time.  The marks made on the surface (S) will result in the shape of an arc (A).


4.  Connect the dots on the Surface (S) by connecting them with a smooth curved line.


5.  Find the shortest distance from the bottom of the Post (P) to the arc (A).  Place a mark N on arc (A) at the spot closest to the post (P).


6.  Draw a line from the base of Post (P) to the point N.  This line represents True North .  We will call the line TN.


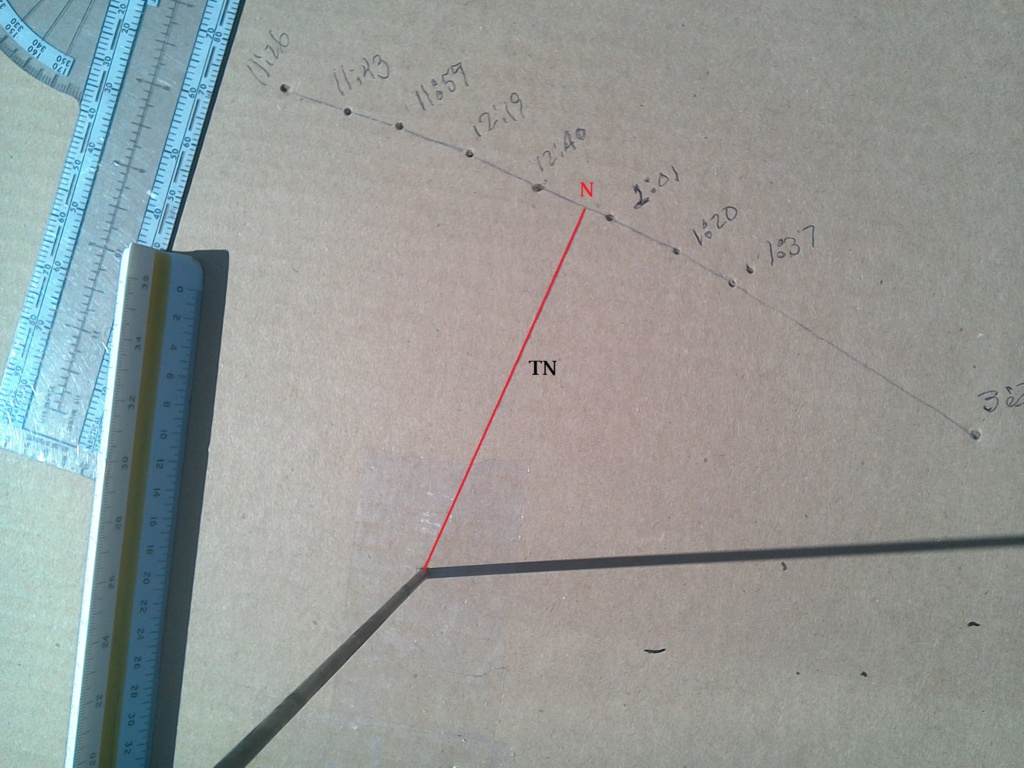
Why is this?  When the Sun is at its highest point in the sky at your location -- the zenith, the shadows will be the smallest.

Find Latitude

coming next time

BDH

Find Latitude

Continuing with Day One, we can now calculate Latitude based on the
1. Height of the Post,
2. the Length of the line TN, as well as
3. a Tangent table or Tangent calculator, and
4. the current position of the Sun latitude-wise on Day One.

Note: In an earlier post, I calculated the amount of change in degrees per day of the Sun's position between the solstices and equinoxes based on 23.5 degrees instead of 23.44 degrees.
I will now post a more correct calculation of change in degrees per day here:

Time between June 24 and September 21 = 89 days = 128,160 minutes.
23.44 degrees North to 0 degrees (Equator)

Sun moves South to Equator at 23.44 degrees / 89 days = .263 degrees/day

Time between September 21 and December 21 = 91 days  = 131,040 minutes.
0 degrees (Equator) to 23.44 degrees South

Sun moves South from equator at 23.44 degrees / 91 days = .258 degrees/day


Time between December 24 and March 21 = 87 days = 125,280 minutes.
23.44 degrees South to 0 degrees (Equator)

Sun moves North to Equator at 23.44 degrees / 87 days = .27 degrees/day

Time between March 21 and June 21 = 92 days =  132,480 minutes.
0 degrees (Equator) to 23.44 degrees North

Sun moves North from Equator to 23.44 degrees at 23.44 / 92 days = .255 degrees/day

--------------------

Tangents

To know the current latitude of our location, we will calculate the angle to the Sun at its Zenith by taking into account the current latitude of the Sun on this day, and either adding or subtracting to or from it to get the Latitude at our location.

First, here is the definition of Tangent:


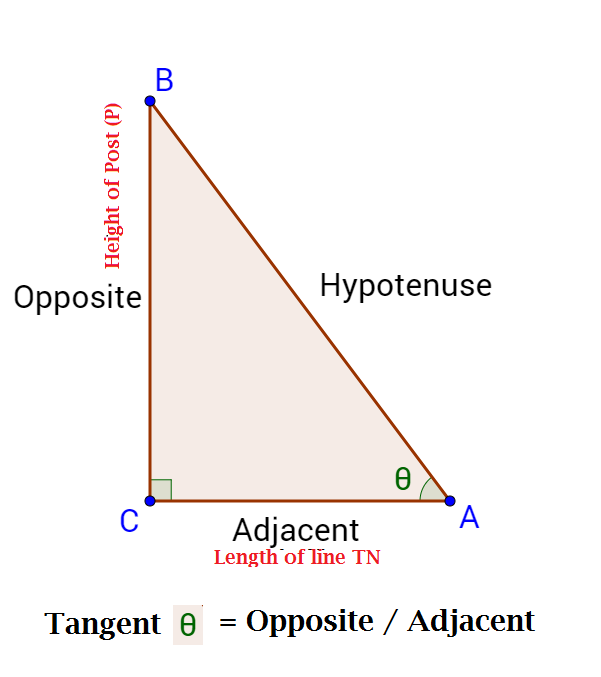
To be continued.....

Find Latitude (cont.)

To find the angle
divide the Height of the Post by the length of line TN to get Tan

To get the value of the angle
Use Arctan (Height / Length of TN) to get .

Arctan calculator here:  Arctan Calculator

--
For example, say Height of Post P = 9 and Length of line TN = 4.5.  

9 divided by 4.5 = 2

Arctan of 2 = 63.43494882 degrees

Thus = 63.43494882 degrees
--

Next, calculate the position of the Sun on Day One in terms of its Latitude.

For example, the date of the exercise was on July 18th, when the sun has started its trip South from the Tropic of Cancer.
Referring to the table in the previous post, we have:

Time between June 24 and September 21 = 89 days = 128,160 minutes.
23.44 degrees North to 0 degrees (Equator)

Sun moves South to Equator at 23.44 degrees / 89 days = .263 degrees/day

From June 24 to July 18 is 24 days.

24 days times .263 degrees/day = 6.312 degrees.
Subtract 6.312 degrees from 23.44 degrees = 17.13 degrees North Latitude

The Sun on July 18th is at 17.13 degrees North Latitude.

To get the Latitude of the location in my example where = 63.43494882 degrees,

Subtract 17.13 degrees from 63.43494882 degrees to get 46.3 degrees North Latitude

We will find Longitude on Day Two.

BDH

Note on Latitude calculations North of the Equator and South of the Equator.

In the example above, when the Sun is North of the Equator, and my location is North of the Equator.
The Latitude of the Sun will be subtracted from the angle

If the Sun is North of the Equator and the location is South of the Equator, the Latitude of the Sun will be added to the angle

If the Sun and the location are both South of the Equator, the Latitude of the Sun will be Subtracted from

Summarizing, one can say that if both Location and Sun are on the same side of the Equator, one Subtracts,
and if Location and Sun are on opposite sides of the Equator, one Adds.

BDH

Day Two

Find Longitude

To find the Longitude at one's location two items are required:

a.  The shadow of the Post (P) falling directly on the line TN (marking the Sun's zenith) and
b.  The current local  time (to the second) along with the time in London England (UTC).

Note:  UTC will be used at the base time for our Longitudes rather than adopting something completely new.

1.  The following photos are taken as the Sun approaches the line TN (its Zenith)





The time is recorded as the shadow falls completely over line TN


The time taken locally is compared to the time in London England UTC or GMT

In my example, the Sun crossed the line TN at 12:53:29 pm local time.
The time in London England was 19:53:29 pm

The difference is 7 hours. **

** Since there are parts of the world that use Daylight Savings Time and some do not, let us perform the time difference based on Gleason's map, which map provides a consistent time difference between your general location and Greenwich Mean Time.

We know that the Sun moves once around the Earth, that is 360 degrees in 24 hours.  This equates to 15 degrees per hour.

Establishing a baseline in degrees to my 12 o'clock hour, it comes to 15 degrees times 7 = 105 degrees.

So I am at some longitude greater than 105 degrees as the Sun passed the Zenith at my location at 12:53:29.

To get to my longitude then, I add the 53 minutes and 29 seconds in degrees to the 105.

53 minutes 29 seconds in decimal = 0° 53' 29" = 0° + 53'/60 + 29"/3600 = 0.8913889
Decimal converter here:  Convert Degrees to Decimals

0.8913889 of one hour (or 15 degrees) = 0.8913889° times 15 = 13.37 degrees.

Thus, the Longitude at my location comes to:  105 plus 13.37 = 118.37 degrees W Longitude.

I will follow up in my next post with the simplified version of the process of finding Latitude and Longitude locally.

BDH

Reviewing the previous section on Finding Longitude,  the question has arisen regarding the Sun coming back to the local meridian at the exact same time the following day.
If the Sun does cross the local meridian (the True North line) at the same time every day, then no further discussion is required.
However, if the Sun does not cross the local meridian at the exact same time the next day, then we will have to discuss the Analemma and the Equation of Time when arriving at the more exact Longitude reading for our locality.



I believe I have posted the Equation of Time in an earlier post and then soon dismissed it.  Not so fast.  

This question has come up due to my further testing with the Latitude and Longitude derivations.  I have found that my Latitude calcs for the Sun are matching nicely.  However, the Longitude calculation has failed to provide the precision I would like to see.  
One of two things may have occurred to upset the Longitude calc.  My setup was moved slightly in the past few days, OR  the Analemma needs to be brought into the calculation to give me the precision required for Longitude.

If it is the Analemma, then a discussion of the Equation of Time may be needed.  



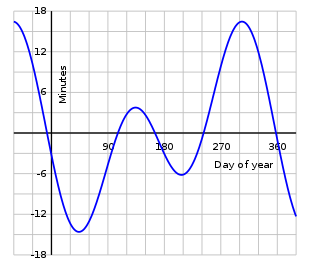
BDH

BDH - your observations are good . You are seeing and charting the change of sun position as it travels around the plane and between the Tropics.  The Analemma photo is proof that it is the sun that orbits and moves nearer or farther , higher/lower as the seasons progress.

The heliocentric model cannot account for this observed motion of the sun .

The equation of time attempts to explain this with math trickery . Actual observed sun position is mapped onto a graph and matched with the imaginary (theoretical) position of where it should be according to the heliocentric model . It's nonsense.

The sun does not move at a constant 15 degrees per hour so you might be able to adjust your calculations to factor this in . The amount of change of velocity will depend on your place of observation. Sounds complicated. Hope you can do it.

Hi RedorBlue,
Yes!  The Analemma provides the proof that the Sun is moving between the Tropics -- North to the Tropic of Cancer (June 21) and South to the Tropic of Capricorn (December 21).


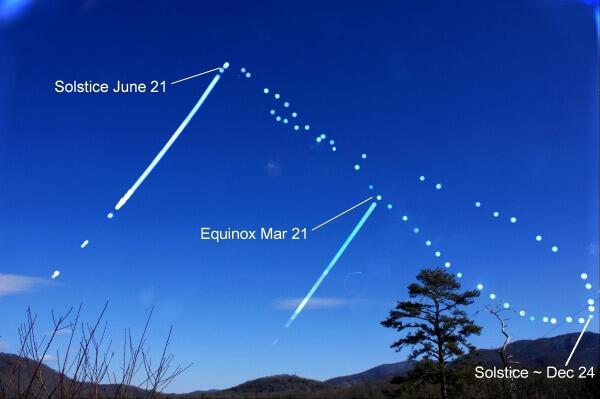
And I have calculated a table that charts this motion.  Thus, half the motion of the Analemma is explained and measured.

However, the East-West motion of the Sun as shown in the Analemma is the question.



This deviation East and West has an affect on the drawing of True North on the surface of my setup.  The arc formed each day will vary and so will change the point on the arc where it is the shortest distance to the post (P).  The shortest point will swing back and forth like the Analemma does.

Going forward, two things have to be established:
1.  Still using the Sun, the correct meridian indicating True North will have to be determined by choosing a particular date that works for this.
2.  What is the degree of deviation based on a given date for calculating True North?  That is, how many degrees do we use for any date on the calendar?

For a solution to #1:
From the following illustration, the Solstices are being used as the True North indicator on the Analemma.


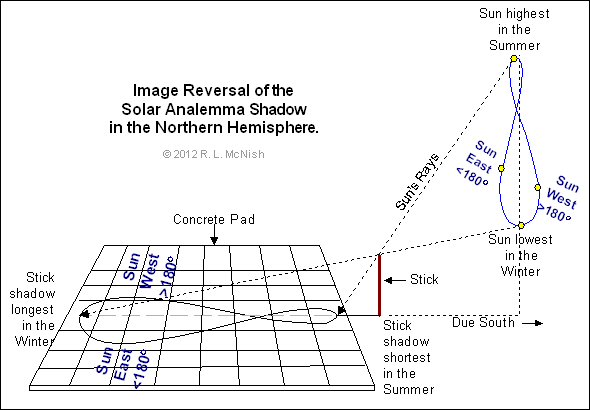
Marking True North with no deviation will be  set to June  21 and December 21.
The test will be marking the shortest distance on the arc to the post on June 21st and then doing so on December 21st.  If these line up, we have our True North line.

Next, how to determine degree of deviation on any given date in the calendar so that one does not have to wait until the Solstice to get the True reading of North?

This brings up the question of the Equation of Time and its possible usage here.  Is there a relationship between the Equation of Time and the Analemma?

See the following illustration that makes such a relationship:


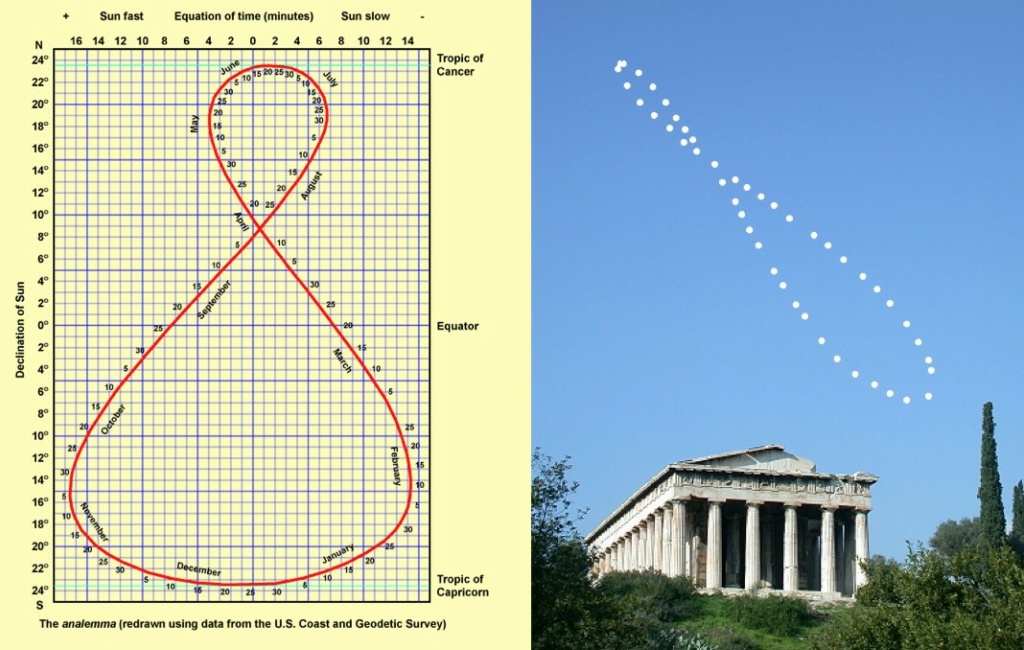
So, can we use the minutes deviation in the Equation of Time  to get the offset for determining  True North on any given day of the year?

The answer will be in the testing, which I shall perform with the setup I have in place.
I will provide the calculation next.

BDH

Another illustration of the Analemma with minute marker deviations associated with every 5 days in the year.



It would appear that December 25th and June 15th are the golden  dates for no deviation off the local meridian.

BDH

Further note on the illustrated Analemma in previous post:

The compressed scale of the shape of the Analemma indicates a skewing of the Sun's Declination values to fit the globe model.  Testing via real world experience shows a marked difference in the values of the Sun's Declination as compared to the chart in the previous post.
Likewise, note the difference in shape between the Analemma illustration and the photographic evidence.  The illustration is a fat exaggeration of the real Analemma evidenced by photographs.

As such, the illustrations showing the numbers of the Sun's declination indicate that the values are coming from somewhere else, as they do not fit Real World data.

Summarizing, one can use the Deviation values on the Analemma chart but dismiss the Sun's Declination values.  A more representative chart can be constructed which will more perfectly fit what we see in reality.  
Like this real world analemmatic sundial !!


BDH

Further research is indicating the following:
-- The Analemma is accurate both for the North-South procession of the Sun and the East-West deviation as the Sun speeds up and slows down throughout the year.
-- As such, I am adjusting my calculations to match those of the Analemma as regards North-South and East-West.
Yes, this is contradicting what I stated earlier about dismissing the Sun's Declination values in the Analemma table. But I can see now that the North-South procession of the Sun is not a constant linear procession but in fact forms the curved path one sees in the Analemma.
-- Consequently, the known data from the Analemma will be used as the measuring base for our calculations of Latitude and Longitude.

How is Latitude affected by using the Analemma?
The calculation for Latitude will utilize the Declination of the Sun found in the Analemma tables.
This will vary to some degree from the table I had constructed earlier.

How will Longitude by affected by using the Analemma?
The East-West deviations found in the Analemma table will be incorporated in the calculation of Longitude as well.

How about True North?
True North, being the most important consideration in calculating Latitude and Longitude, does have 4 dates on the Analemma that show NO deviation from the local Meridian -- April 17, September 2, December 25, and June 15.
That is, on those dates, when the Sun reaches the Zenith of the local meridian, its shadow will point True North with no Deviation.  Of course we can get True North on any date using the deviations provided in the Analemma table.

Going forward then, we will use the Analemma table for calculating local Latitude and Longitude as the Analemma is found all around the world and as such is consistent for our use in helping map out the flat Earth.


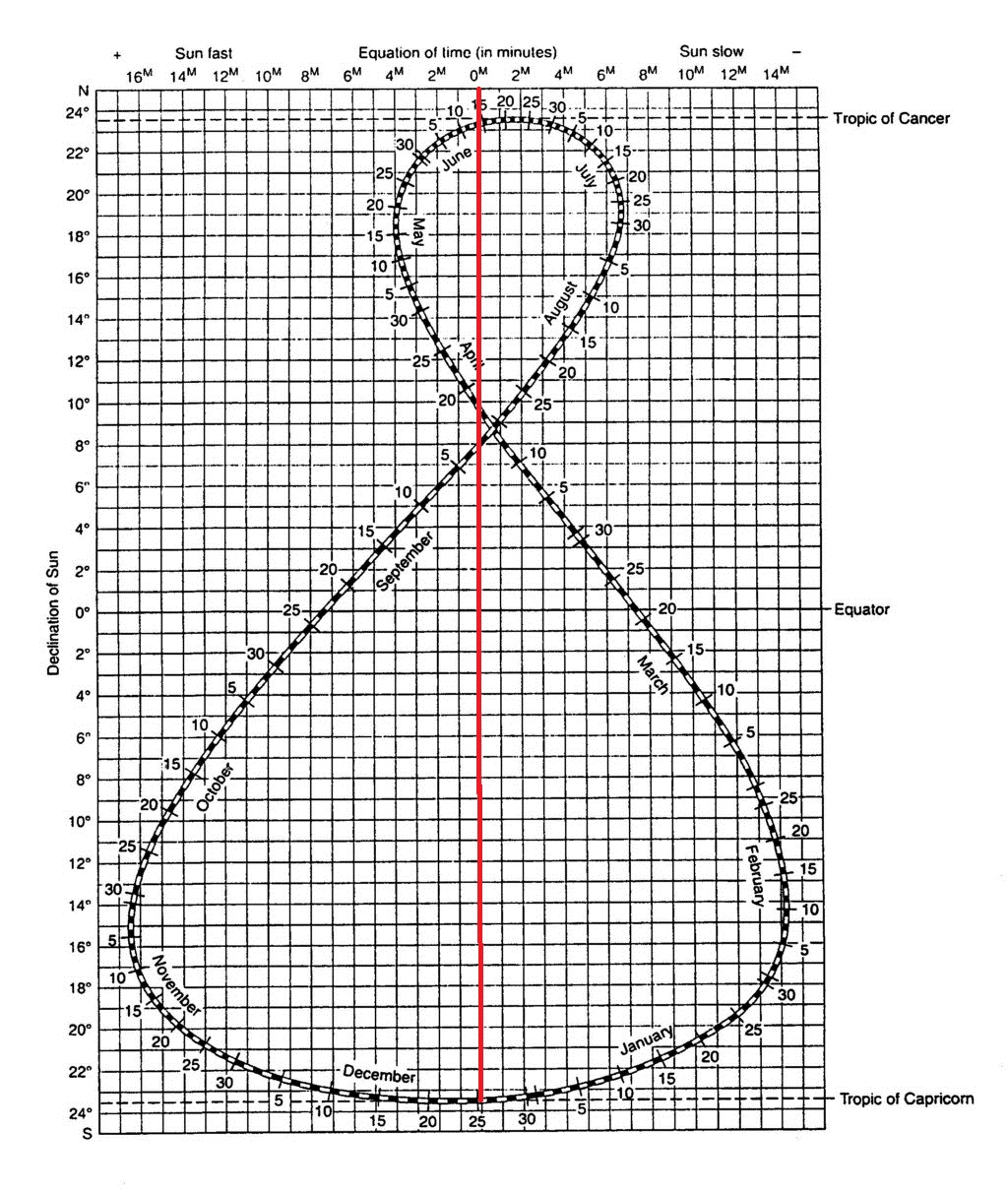
Handy table for degrees of Declination throughout the year



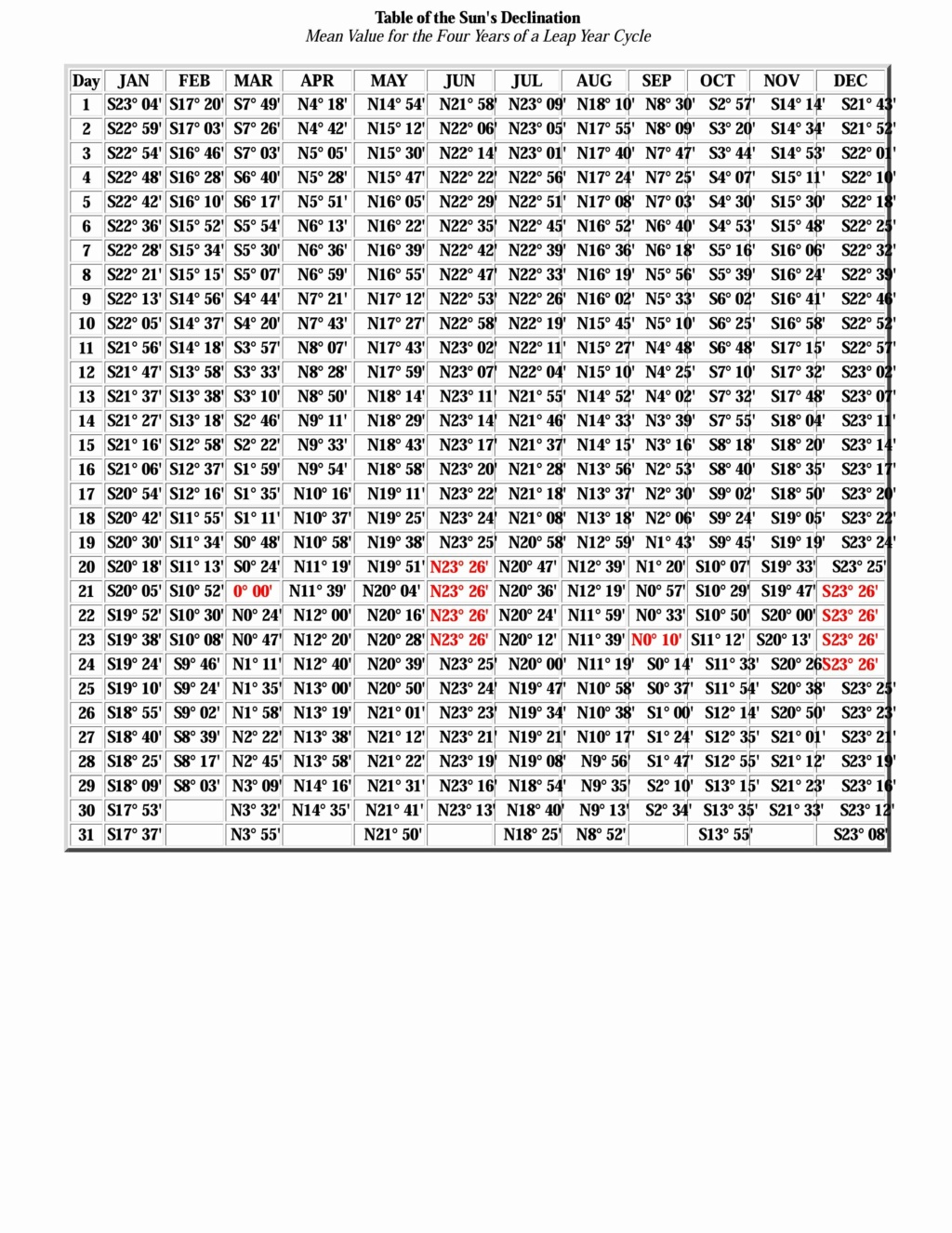
BDH

You can check true N against the pole star if you can see it - if it aligns with sun position on those days you mention then that's good .

Final note on True North

As I more fully understand the workings of the Analemma with the fact that the Sun slows down and speeds up East and West and North and South, one thing is unchanging and is always easily calculable -- the finding of True North.

Finding True North does not need any table with deviations or declinations to arrive at its calculation.
All is needed, and I have shown that in the earliest post on finding True North is the fact that the True North line will be that line drawn from the Post to the point on the arc that is the smallest distance from the Post.  
This means that the Sun has just hit the local meridian (above one's head) and the shadow case by the Post will be the smallest in comparison with all other shadows made that day.

True North will never change, no matter what speed the Sun is taking to get to the meridian the next day -- be it faster or slower than before.  The fact is, when it hits the local meridian, its shadow cast will be the smallest.
It does not matter either how far North or how far South the Sun is in the sky or what time of year it is.  As soon as the Sun hits the local meridian, the shadow cast by the Post will be the smallest.

BDH

Find Latitude Version 2.0

Finding Latitude 2.0 is literally the same as my earlier post on Finding Latitude save for the fact that I will use the Table of Sun's Declination (from the Analemma) in my calculation for local Latitude instead of the estimations I had constructed.

In my example Latitude will be found thusly:

To find the angle
divide the Height of the Post by the length of line TN to get Tan

To get the value of the angle
Use Arctan (Height / Length of TN) to get .

Arctan calculator here:  Arctan Calculator

--
For example, say Height of Post P = 9 and Length of line TN = 4.5.  

9 divided by 4.5 = 2

Arctan of 2 = 63.43494882 degrees

Thus = 63.43494882 degrees
--

Next, calculate the position of the Sun on Day One in terms of its Latitude.

For example, the date of the exercise was on July 18th, when the sun has started its trip South from the Tropic of Cancer.
Referring to the table of Declination Values, the Declination of the Sun on July 18 is:
21 degrees 8 minutes

To get the Latitude of the location in my example where = 63.43494882 degrees,

Subtract 21 degrees 8 minutes from 63.43494882 degrees (63° 26' 5.82") to get  42° 18' 5.82"  North Latitude

BDH

Finding Longitude Version 2.0

Finding the Longitude is essentially the same as I earlier posted except now we use the Deviation values found in the Analemma.


In my example:

The time is recorded as the shadow falls completely over line TN

The time taken locally is compared to the time in London England UTC or GMT

In my example, the Sun crossed the line TN at 12:53:29 pm local time.

The time in London England was 19:53:29 pm

The difference is 7 hours. **

** Since there are parts of the world that use Daylight Savings Time and some do not, let us perform the time difference based on Gleason's map, which map provides a consistent time difference between your general location and Greenwich Mean Time.

Next, depending on how much faster or slower the Sun arrived at the meridian, we take the time deviation value for the particular date and subtract or add.  In this case, the date was July 20th.

The deviation for July 20 from the Analemma is:  6.33 minutes.  The Sun was 6.33 minutes slower in arriving to the meridian.
As such, we subtract 6.33 minutes from the current time to arrive at:
12:53:29 - 6:20 = 12:47:09 pm local time


We know that the Sun moves once around the Earth, that is 360 degrees in 24 hours.  This equates to 15 degrees per hour.

Establishing a baseline in degrees to my 12 o'clock hour, it comes to 15 degrees times 7 = 105 degrees.

So I am at some longitude greater than 105 degrees as the Sun passed the Zenith at my location at 12:47:09.

To get to my longitude then, I add the 53 minutes and 29 seconds in degrees to the 105.

47 minutes 09 seconds in decimal = 0° 47' 09" = 0° + 47'/60 + 9"/3600 = 0.7858333


0.7858333 of one hour (or 15 degrees) = 0.7858333 times 15 = 11.787 degrees.

105 degrees PLUS 11.787 degrees = 116.787 degrees.

Thus, the Longitude at my location comes to:   116° 47' 13.2" W Longitude.

I will summarize what I have established as the basics for mapping the Earth in its Reality using only a stick, some level ground, a calculator, the experience of hundreds of years of sun watchers, and the internet.

Based on my experimentation using a 9 inch post on a piece of cardboard taped to an old wood table set at level East-West, North-South, I can repeat what I have been doing within a DEGREE of error Latitude and Longitude -- wise.  Not bad.

Note:  Based on what I stated in the first post on this subject, I believe that the Greece-ballers had to stretch the Earth over a ball to get the 90 degrees North and South, thus skewing Latitude values.  I am making an educated guess here that the Latitudes we arrive at in our mapping will be somewhat different than the Royal Academy's Greece-ball figures.

Simple steps coming next.

For the short term, we need a group of testers to take my steps to fruition.

BDH

Here is an old saying about how to calculate the particular deviation in the Analemma:

On September one, trust the Sun.
Come Halloween, subtract sixteen.
On Christmas Day, you're OK.
For your Valentine true, add a dozen and two.
The mid of month four, add no more
At the mid of May, take four away.
On June fourteen, don't add a bean.
When August begins, add seven little mins.
The rest is easy: for any date, All you do is interpolate.
a poem by Tad Dunne


BDH

Hello BDH!

Here is my calculation on day one. Tomorrow I will send you longitude calculation as well.

Please, correct me if I'm wrong though, because if I am right then I should be feeling the nice breeze of Antarctica right now!

Date of calculation = Aug 5th
Sun declination = N 17" 8'

Height of Post = 30 cm
Lenght of line = 25.4 cm

Latitude of my location = 66° 52' 47.48" South Latitude


Pictures of the experiment = https://imgur.com/a/08iW54o

BDH

btw, when I started reading your instructions for second day calculations I found out that, by coincidence, my TN line discovered today landed right into a time demarcation point (11h 54m 53s). So I guess no second day calculation is necessary as I already have the time when the shadow of the Post fell on the TN line, right?

If so then that's my longitude = 42°13'W


I will be waiting for your eventual assistance in correcting any mistakes I might have made on my calculations.

Cheers!

Hi bdhfe, thanks for all your work throughout the year regarding this topic. I can see you spent a lot of time on the mapping technique research.
I am not particularly new to this forum but decided to create an account to participate in the FE mapping. Been watching Eric since ´16, so thanks to Eric as well for providing a platform for serious research. As an geographer, this mapping is very interesting for me. I will try to do the calculations in the coming week.
Greetings from Germany and keep on going.

leotruther,
Fantastic work!!   Great setup.
You have shown the problem with my Latitude calculations which I have now confirmed in using my ruler and navigation plotter.
I have stated that one adds or subtracts the ANGLE of the Sun's declination to the angle calculated from the shadow cast from the Post.  This is INCORRECT.
The addition or subtraction is not made with the angle itself, but with the length of the shadow cast on line TN.  This is because the length of the shadow is the moving variable.
Thus, a new angle to be added or subtracted is calculated using the adjusted length of the shadow cast.
I will follow up in another post to demonstrate this.

Regarding your Longitude calculation, two important items to include in the calculation:
1.  How many hours different between your time and GMT?
2.  How many minutes to be adjusted based on the Analemma?  We know the Sun is slow in getting back to the meridian right now, so subtract those minutes as well.
If these two are addressed, then Day Two is not necessary and Great work again!!

BDH

outerworlds44,
Great to hear from you and welcome to the mapping club!!
As a geographer, you are going to be a tremendous asset to this endeavor.

BDH

BDH,

Happy to be of assistance!


Regarding your questions:

1.  How many hours different between your time and GMT?

2 hours

2.  How many minutes to be adjusted based on the Analemma?  We know the Sun is slow in getting back to the meridian right now, so subtract those minutes as well.

6 minutes.

* I am in Rio de Janeiro


Cheers!