Direct Vision, Rationality, Realism and Common Sense.
+14
Realearth
liminoid
Admin
Real World
FL@T-E@RTH
Ziggy Kelleher
RelearningLogic
lizardking
Schpankme
dnawebma
thesilentone
Occamania
csp
vortexpuppy
18 posters
IFERS - Exposing the 'Global' Conspiracy From Atlantis to Zion :: The International Flat Earth Research Society
Page 3 of 5
Page 3 of 5 •  1, 2, 3, 4, 5
1, 2, 3, 4, 5 
 Theories of Right Lines (straight and level) and Parallism
Theories of Right Lines (straight and level) and Parallism
Extracts and highlighted passages from the book …
https://archive.org/details/theoriesofparall00fran
THEORIES OF PARALLELISM AN HISTORICAL CRITIQUE
BY WILLIAM BARRETT FRANKLAND, M.A.
SOMETIME FELLOW OF CLARE COLLEGE, CAMBRIDGE: VICAR OF WRAWBY, 1910
Edited by Vortexpuppy
Euclid
(A) Necessary concessions
1) Let it be conceded that from every point to every point a straight line can be drawn;
(2) And a limited straight line can be produced continually in a straight line
(3) And for every centre and distance a circle can be described;
(4) And all right angles are equal to each other;
(5) And if a straight line falling on two straight lines make the angles within and towards the same parts less than two right angles, then the two straight lines being indefinitely produced meet towards the parts where are the angles less than two right angles,
(B) Universal Ideas
1) Equals to the same are also equal to each other
2) And if to equals, equals are added, the whole are equal;
3) And if from equals, equals are subtracted, the remainders are equal;
4) And things coinciding with each other are equal to each other;
5) And the whole is greater than the part.
POSIDONIUS
(lost work on Geometry, B.C. 80)
"Posidonius says that parallel lines are such as neither converge nor diverge in one plane, but have all the perpendiculars drawn from points of one to the other equal. On the other hand such straight lines as make their perpendiculars continually greater or less will meet somewhere or other, because they converge towards each other"
GEMINUS
(Doctrine of Mathematics, B.C: 70)
"We learned from the very pioneers of the Science never to allow our minds to resort to weak plausibility’s for the advancement of geometrical reasoning
AGANIS
"If two straight lines are equidistant, the space between them is perpendicular to each of these lines."
PROCLUS
Commentary on the First book of the elements, A.D. 450
"It cannot be asserted unconditionally that straight lines produced from less than two right angles do not meet. It is of course obvious that some straight lines produced from less than two right angles do meet, but the (Euclidean) theory would require all such to intersect. But it might be urged that as the defect from two right angles increases, the straight lines continue asecant up to a certain magnitude of the defect, and for a greater magnitude than this they intersect.”
"Parallelism is similarity of position, if one may so say"
GERBERT
Mathematical works, A.D. 1000
"Two straight lines distant from each other by the same space continually, and never meeting each other when indefinitely produced, are called parallel, that is, equidistant."
BILLINGSLEY
The elements of geometrie A.D. 1570
"Parallel or equidistant right lines are such; which being in one and the selfe same superficies, and produced infinitely on both sydes, do never in any part concurre."
TACQUET
Elementa Geometriae Planae et Solidae, Amsterdam, 1654.
(11) Parallel lines have a common perpendicular,
(12) Two perpendiculars cut off equal segments from each of two parallels.
"Their truth is immediately apparent from the definition of parallelism."
HOBBES
De Corpore, 1655 : et caetera.
"There is in Euclid a definition of strait-lined parallels but I do not find that parallels in general are anywhere defined and therefore for a universal definition of them, I say that any two lines whatsoever, strait or crooked, as also any two superficies, are parallel, when two equal strait lines, wheresoever they fall upon them make equal angles with each of them.
From which definition it follows; first, that any two strait lines, not inclined opposite ways, falling upon two other strait lines, which are parallel, and intercepting equal parts in both of them, are themselves also equal and parallel" (De Corpore, c. 14, § 12).
“How shall a man know that there be strait lines which shall never meet though both ways infinitely produced?” {Collected Works, Vol. 7, page 206}.
WALLIS
Demonstratio Postulati Quinti Euclidis, 1663.
"It is known," he began, "that some of the ancient geometers, as well as the modern, have censured Euclid for having postulated, as a concession required without demonstration, the Fifth Postulate, or (as others say) the Eleventh Axiom, or, with the enumeration of Clavius, the Thirteenth Axiom... But those who discover this fault in Euclid do themselves very often (at least, as far as I have examined them) make other assumptions in place of it, and these appear to me no easier to allow than what Euclid postulates... Since nevertheless I observe that so many have attempted a proof, as if they esteemed it necessary, I have thought good to add my own effort, and to endeavour to bring forward a proof that may be less open to objection than theirs."
Wallis laid down 7 simple lemmas and then his radical 8th.
“VIII. At this stage, presupposing a knowledge of the nature of ratio and the definition of similar figures, I assume as an universal idea : To any given figure whatever, another figure, similar and of any size, is possible. Because continuous quantities are capable both of illimitable division and illimitable increase, this seems to result from the very nature of quantity; namely, that a figure can be continuously diminished or increased illimitably, the form of the figure being retained.”
LEIBNIZ
Characteristica Geometrica, 1679
"This definition seems rather to describe parallels by means of a more remote property than that which they most evidently display and one might doubt whether the relationship exists, or whether all straight lines in the plane do not ultimately intersect each other " (page 200).
DA BITONTO
Euclide Mestituto, 1680.
“The perpendiculars let fall from points of any curve upon any straight line cannot all be equal.”
SACCHERI
Euclides ah omni naevo Vivdicatus, Milan, 1733.
Saccheri protested against the procedure of early Geometers who "assume, not without great violence to strict logic, that parallel lines are equally distant from each other, as though that were given a priori, and then pass on to the proofs of the other theorems connected therewith" (page 46).
SIMSON
Euclidis elementorwn lihri priores sea;... Glasgow, 1756.
Definition 1. The distance of a point from a straight line is the perpendicular from the point upon the straight line.
Definition 2. A straight line is said to approach towards, or recede from, another straight line according as the distances of points of the former from the latter decrease or increase. Two straight lines are equidistant, if points of the one preserve the same distance from the other.
Axiom: A straight line cannot approach towards, and then recede from, a straight line without cutting it; nor can a straight line approach towards, then be equidistant to, and then recede from, a straight line; for a straight line preserves always the same direction.”
LAMBERT
Die Theorie der Parallel-linien, 1766.
These are again the parabolic, elliptic and hyperbolic hypotheses of Dr Klein. The elliptic hypothesis (ii) soon dropped out of Lambert's hands, owing to his extension to the entire (infinite) plane of results into which Euc. I. 16 enters. But the hyperbolic hypothesis (iii) was successfully worked out to conclusions implying an absolute standard of length. On this Lambert remarked (§§ 80, 81): "This consequence possesses a charm which makes one desire that the Third Hypothesis be indeed true”.
"Yet on the whole I would not wish it true, notwithstanding this advantage (of an absolute standard of length), since innumerable difficulties would be involved therewith. Our trigonometrical tables would become immeasurably vast (compare pages xviii and 57, above and below); the similitude and proportionality of geometrical figures would wholly disappear, so that no figure can be represented except in its actual size astronomy would be harassed (see our Second Additional Note); etc.
“Still these are “argumenta ah amove et invidid ducta”, which must be banished from Geometry as from every science.”
"I revert accordingly to the Third Hypothesis. In this hypothesis, as already seen, not only is the sum of the three angles of every triangle less than 180°; but this difference from 180° increases directly with the area of the triangle; that is to say, if of two triangles one has a greater area than the other, then in the first the sum of the three angles is less than in the second."
BERTRAND
Developpement Nouveau de la Partie EUmentaire des Mathematiques, Geneva, 1778.
"Geometry, like every other science, has its roots in ideas common to all. From this fount of ideas, the first originators derived those principles and germs of knowledge which they bestowed upon mankind. Hence it appears that in every science two parts can be distinguished; the first, consisting of the assemblage of principles or primitive conceptions from which the science proceeds; and a second, comprising the development of the consequences of the principles. In respect of these principles as they exist in every mind, science would seem to encounter no resistance or difficulty. Yet the choice that has to be made of first principles, the degree of simplicity and elegance to which they have to be reduced, and the necessity of enunciating them in precise terms, capable of clear comprehension, all this is very difficult."
"The spectacle of the universe displays before our eyes an immense space. In this immensity bodies exist and change continually their shape, size and position; and meanwhile space itself, invariable in all its parts, remains like a sea always calm, in everlasting repose. So the idea we form of space is that it is infinite and limitless; homogeneous and like itself at every time and in every place. Space is without bounds, for any we might assign to it would be contained in it, and therefore would not bound it. Space is homogeneous, in that the portion of space occupied by a body in one plane would not differ from that which would be occupied by it elsewhere."
Division of space into two halves identical in all but position gives the plane surface; and division of the plane similarly gives the straight line (so also Leibniz, above, page 19).
If there is no court of appeal from the verdict of "common sense," Bertrand's argument stands, and Euclid's geometry prevails.
PLAYFAIR
Elements of Geometry, Edinburgh, 1795.
"Two straight lines which intersect one another, cannot be both parallel to the same straight line."
Of this Axiom of Playfair's, Cayley said: "My own view is that Euclid's Twelfth Axiom in Playfair's form of it, does not need demonstration, but is part of our notion of space, of the physical space of our experience, which is the representation lying at the bottom of all external experience" {Presidential Address to the Britisli Association, 1883}. Dr Russell cites and criticises this interesting confession in his admirable Foundations of Geometry (Cambridge, 1899, page 41).
LAPLACE
Exposition du Systeme du Monde, Paris, 1796.
"The law of attraction, inversely as the square of the distance, is that of emanations which proceed from a centre. One of its remarkable properties is, that if the dimensions of all the bodies in the universe, their mutual distances and velocities, increase or diminish proportionally, they describe curves entirely similar to those which they at present describe; so that if the universe be successively reduced to the smallest imaginable space, it will always present the same appearance to all observers... The simplicity of the laws of nature therefore only permits us to observe the relative dimensions of space."
The translator's note (page 536) may be reproduced here
"The endeavours of Geometers to demonstrate Euclid's Twelfth Axiom about parallel lines have been hitherto unsuccessful. However, no person questions the truth of this Axiom, or of the Theorems which Euclid has deduced from it.
The perception of extension contains therefore a peculiar property which is self-evident, without which we could not rigorously establish the doctrine of parallels. The notion of a limited extension (for example, of a circle) does not involve anything that depends on its absolute magnitude; but if we conceive its radius to be diminished, we are forced to diminish in the same proportion its circumference, and the sides of all the inscribed figures. This proportionality was, according to Laplace, much more obvious than that of Euclid.
GAUSS
Letters and Reviews, 1799-1846.
"I have arrived at much which most people would regard as proved, but it is in my eyes good for nothing in this respect. For example, if it could be proved that a rectilinear triangle is possible, of area exceeding any assigned area, I should be in a position to prove rigorously the whole of (Euclidean) geometry. Now most people would regard this as axiomatic, but I do not. It would be quite possible that however distant from each other the vertices of the triangle were assumed to lie in space, the area should still be less than an assignable limit.
I have more propositions of a similar character, but in none of them do I find anything really satisfying."
Thus Gauss seems to have worked out several fundamental theorems of hyperbolic geometry but not so completely as to feel ready to publish them. His letter to Taurinus, a facsimile of which forms the frontispiece to Parallellinien von Euklid his auf Gauss, reads as follows:
"I have read not without pleasure your kind letter of October 30th with the small sketch enclosed, the more so because I have been accustomed to discover scarcely any trace of pure geometrical spirit among the majority of people who make the new attempts on the so-called Theory of Parallel Lines. With reference to your attempt I have nothing, or not much, to observe except that it is incomplete. Your presentation of the proof that the sum of the three angles of a plane triangle cannot be greater than 180° leaves much to be desired in respect of geometrical rigor. This in itself could be remedied, and beyond all doubt the impossibility can be proved most rigorously. Things stand otherwise in the second part, that the sum of the angles cannot be less than 180°; this is the crucial point, the reef on which all the wrecks take place.
I imagine that you have not been long occupied with this subject. My own interest in it has extended over 30 years, and I do not think that anyone can have occupied himself more with this second part than I, although I have never published anything on it. The assumption that the sum of the three angles of a triangle is less than 180° leads to a peculiar Geometry entirely different from ours, a geometry completely self-consistent, which I have developed for myself perfectly satisfactorily, so that I can solve any problem in it with the assumption that a constant is determinate, this constant not being capable of a priori specification.
The greater this constant is assumed to be, the more nearly is Euclidean Geometry approached; and an infinite value of the constant makes the two systems coincide. The theorems of this geometry seem somewhat paradoxical, and to the lay mind absurd; but continued steady reflection shows them to contain nothing at all impossible.
Thus, for instance, the three angles of a triangle can be as small as we please, if only the sides are taken sufficiently great; and yet the area of a triangle can never exceed a definite limit, however great the sides are taken to be, and indeed can never reach it.
All my efforts to discover a contradiction, an inconsistency, in this non- Euclidean Geometry have been unsuccessful; and the one thing in it contrary to our conceptions is that, were the system true, there must exist in space a linear magnitude, determined for itself albeit unknown to us.
But methinks, despite the say- nothing word-wisdom of the metaphysicians, we know far too little, too nearly nothing, about the true nature of space, for us to confuse what has an unnatural appearance with what is absolutely impossible.
If the non-Euclidean Geometry were true, and that constant at all comparable with such magnitudes as lie within reach of our measurements on the earth or in the heavens, it could be determined a posteriori.
Hence I have sometimes expressed in jest the wish that the Euclidean Geometry were not true, since then we should possess an absolute standard of measurement a priori. I am not afraid that a man who has shown himself to possess a thoughtful mathematical mind will misunderstand the foregoing; but in any case, please regard this as a private communication, of which public use, or use leading to publicity, is not to be made in any way. If at some future time I acquire more leisure than in my present circumstances, I shall perhaps publish my investigations.”
CARNOT
Geometrie de Position, Paris, 1803.
Page 59
Carnot advocated the Principle of Similitude as an alternative to the Parallel-Postulate, a view-point secured with greater elaboration by Wallis (above, page 18). He wrote (§ 435):
"The Theory of Parallels rests on a primitive idea which seems to me almost of the same degree of clearness as that of perfect equality or of superposition. This is the idea of Similitude. It seems to me that we may regard as a principle of the first rank that what exists on a large scale, as a ball, a house, or a picture, can be reduced in size, and vice versa and that consequently, for any figure we please to consider, it is possible to imagine others of all sizes similar to it; that is to say, such that all their dimensions continue to be in the same proportions. This idea once admitted, it is easy to establish the Theory of Parallels without resorting to the idea of infinity.
W. BOLYAI
Theoria Parallelarum, Maros-Yasarheli, 1804 Kurzer Grundriss, 1851.
In the second tract was introduced an ingenious substitute for the Parallel-Postulate: Let it be conceded that "if three points are not in a straight line, then they lie on a sphere": and therefore on a circle.
Like Lobachewski, the younger Bolyai only elaborated a hyperbolic geometry. They both however discussed the properties of curves (X-lines, horocycles) neither rectilinear nor circular, whereof the normals are parallel. Only in Euclidean Geometry is the straight line the limit of a circle of indefinitely increased radius.
THIBAUT
Grundriss der reinen Mathematik, Gottingen, 1809.
Any treatment of parallelism based upon the idea of direction assumes that translation and rotation are independent operations, and this is only so in Euclidean Geometry. The following is the easiest and most plausible way of establishing the parabolic hypothesis. From Euc.i. 32 can be deduced very readily the Euclidean theory of parallelism. Thibaut argued for this theorem.
" Let ABC be any triangle whose sides are traversed in order from A along AB, BG, GA. While going from A to B we always gaze in the direction ABb (AB being produced to b), but do not turn round. On arriving at B we turn from the direction Bb by a rotation through the angle bBG, until we gaze in the direction BGc. Then we proceed in the direction BGc as far as G, where again we turn from Gc to GAa through the angle cGA ; and at last arriving at A, we turn from the direction Aa to the first direction AB through the external angle aAB. This done, we have made a complete revolution, just as if, standing at some point, we had turned completely round ; and the measure of this rotation is 2PI. Hence the external angles of the triangle add up to 2PI, and the internal angles A+B+G = PI. Q.E.D."
TAURINUS
Theorie der Parallellinien, 1825.
Taurinus expressed eight objections to the wider range of Geometry then being manifested (Engel and Stackel, pages 208-9).
"1. It contradicts all intuition. It is true that such a system would in small figures present the same appearance as the Euclidean; but if the conception of space is to be regarded as the pure form of what is indicated by the senses, then the Euclidean system is incontestably the true one, and it cannot be assumed that a limited experience could give rise to actual illusion."
None the less, however, measurement of angles, if sufficiently exact, might deal a fatal blow to Euclid's a priori system, by subverting Euc. i. 32.
" 2. The Euclidean system is the limit of the first system, wherein the angles of a triangle are more than two right angles. With this procedure to the limit, the paradox in connexion with the axiom of the straight line ceases."
"In this theorem (51) it is proved that with the assumption that the angle-sum of a quadrilateral can be greater than four right angles (or, what comes to the same thing, if the angle-sum of a triangle can be greater than two right angles), then all the lines, perpendicular to another line, intersect in two points at equal distance on either side. Hence arises the evident contradiction of the axiom of the straight line, and so such a geometrical system cannot be rectilinear."
" 3. Were the third system the true one, there would be no Euclidean Geometry, whereas however the possibility of the latter cannot be denied."
"4. In the assumption of such a system as rectilinear, there is no continuous transition; the angles of a triangle could only make more or less than two right angles."
The Euclidean system, nevertheless, is the connecting link desired between the elliptic and hyperbolic geometries. It is the limit of each; whether it separates them or unites them is only a matter of words.
" 5. These systems would have quite paradoxical consequences, contradicting all our conceptions; we should have to assign to space properties it cannot have,"
This appeal to common sense is of value in practical work, where close approximation suffices.
" 6. All complete similarity of surfaces and bodies would be wanting; and still this idea seems to have its roots in intuition, and to be a true postulate."
The view of some of the world's greatest thinkers, and they have Wallis for spokesman.
" 7. The Euclidean system is in any case the most complete, and its truth therefore possesses the greatest plausibility."
But all three hypotheses together supply a more complete Theory of Space than any single one of them, and their common basis is the assumption that space is homogeneous. More complete still will be the Geometry of the future, contemplating a heterogeneous space (compare Clifford's speculation below, page 49).
" 8. The internal consistency of the third system is no reason for regarding it as a rectilinear system; however, there is in it, so far as we know, no contradiction of the axiom of the straight line as in the first."
These objections are mainly of an a priori character. On the other hand, our knowledge of space as an objectivity is small.
Increased precision of astronomical instruments might display antipodal images of a few bright stars, and this would then tell in favor of an elliptic hypothesis. The space-constant is large, very large; but no experiment can ever prove it infinite, as Euclideans assume it.
J. BOLYAI
Appendix scientiara spatii eochibens...Maros-YsksarheM, 1832.
Starting from the strict definition of a parallel as the limiting position of a secant, Bolyai proceeded to solid geometry, and deduced the existence of L-lines and F-surfaces (called by Lobachewski horocycles and horospheres), which are the limiting forms of circles and spheres of infinite radius in hyperbolic space. He found Euclidean geometry to obtain for horocycles drawn on horospheres; but for rectilinear triangles drawn on a plane, he proved that the area was proportional to the supplement of the angle-sum (§ 43). The work merits careful study, and comparison with the corresponding work of Lobachewski,
LEGENDRE
Reflexions sur...la Theorie des ParaUeles, Paris, 1833.
"After some researches undertaken with the aim of proving directly that the sum of the angles of a triangle is equal to two right angles, I have succeeded first in proving that this sum cannot be greater than two right angles. Here is the proof as it appeared for the first time in the 3rd edition of my Geometry published in 1800."
i.e. the straight line is not the shortest distance between the two points Qi and Qn+1
The solution of this enigma is that the elliptic hypothesis was actually assumed; and for this hypothesis, the straight line is re-entrant, and Qn+1 may fall, for instance, between Q1 and Q2.
Legendre then proceeded further..
"The first proposition being established, it remained to prove that the sum of the angles cannot be less than two right angles; but we must confess that this second proposition, though the principle of its proof was well-known (see Note ii, page 298, of the 12th Edition of the Elements of Geometry), has presented difficulties which we have not been able entirely to clear away.
This it is which caused us, in the 9th Edition, to return to Euclid's procedure; and later, in the 12th, to adopt another method of proof to be spoken of hereafter.... “It is doubtless due to the imperfection of popular language, and the difficulty of giving a good definition of the straight line, that Geometers have hitherto achieved little success, when they endeavored to deduce this Theorem (Euc. i. 32) from ideas purely based on the equality of triangles contained in the First Book of the Elements."
The notion that the definition of the straight line was in some way the nodus of the Theory of Parallelism had evidently occurred to Taurinus and others, and was expressed as a conviction by Dodgson in his New Theory of Parallels (1895).
Legendre next gave an ingenious and elaborate proof that if the angle-sum of one triangle in the plane is PI, the angle-sum of every triangle is PI. He observed further that if the angle-sum of a triangle were greater (less) than PI, then equi-distants would be convex (concave) to their bases; but failed to make cogent use of this apparent paradox, as it then was.
LOBACHEWSKI
Geometrische Untersuchungen zur Theorie der Parallel-linien, Berlin, 1840.
As Dr Whitehead has said in one of his tracts
"Metrical Geometry of the hyperbolic type was first discovered by Lobachewski in 1826, and independently by J. Bolyai in 1832, This discovery is the origin of the modern period of thought in respect of the foundations of Geometry " {Axioms of Descriptive Geometry, page 71}. The Geometrische Untersuchungen has been done into English by Halsted (Austin, Texas, 1891). Characterising Legendre's efforts as fruitless, the Russian Geometer went on to say:
"My first essay on the foundations of Geometry was published in the Kasan Messenger for the year 1829.... I will here give the substance of my investigations, remarking that, contrary to the opinion of Legendre, all other imperfections, for instance, the definition of a straight line, are foreign to the argument, and without real influence upon the Theory of Parallels."
Then followed a number of simple theorems, independent of any particular theory of parallelism, although the third presents the appearance of contradicting the elliptic hypothesis, thus
"A straight line sufficiently prolonged on both sides proceeds beyond every limit (liber jede Grenze), and so separates a limited plane into two parts."
The ninth of Lobachewski's preliminaries, however, did distinctly assume a theorem not universally valid for the elliptic hypothesis
"In the rectilinear triangle, a greater angle lies opposite the greater side."
The sixteenth step inaugurated the Hyperbolic Hypothesis
"16. All straight lines issuing from a point in a plane can be divided, with reference to a given straight line in this plane, into two classes, namely, secant and asecant. The limiting straight line between one class and the other is called parallel to the given straight line."
Thus if MH is a parallel to NB on one side of the perpendicular MN let fall from M upon NB, then the angle HMN was called the angle of parallelism. Let the length of MN be p, and denote by W the corresponding angle of parallelism HMN, then Lobachewski established rigorously the very curious result:
The complement of W is the Gudermannian of p/K, where K is constant over the plane. Thus the angle of parallelism was determined in all cases; and Lobachewski's result was identical with that obtained above on page xviii.
Lobachewski's proof was intricate, however, and involved the horocycles and horospheres mentioned already.
Very elegant is Lobachewski's application of the construction of Euc. I. 16 (itself a pattern of elegance) to prove that the angle-sum of a triangle cannot exceed two right angles.
There is nevertheless no serious difficulty, provided space is of finite size, as Riemann suggested later.
MEIKLE
Theory of Parallel Lines, Edinburgh, 1844.
"During the long succession of ages which have elapsed since the origin of Geometry, many attempts have been made and treatises written, though with little success, to demonstrate the important Theorem which Euclid, having failed to prove, has styled his 12th Axiom, and which is nearly equivalent to assuming that the three angles of every triangle amount to two right angles."
Except that the elliptic hypothesis is not excluded by the Parallel-Postulate, one might say "exactly equivalent."
By the aid of this magnificent piece of reasoning, Meikle proved that the area of a triangle is proportional to what has been called its divergence; but he rejected the hyperbolic hypothesis on the ground that it involved triangles of finite area with zero angles - the paradox which aroused Gauss' interest (above, page 33).
RIEMANN
Habilitationsrede, 1854.
Riemann's brief but brilliant and epoch-making Essay was translated by Clifford {Collected Papers, page 56}. The possible combination of finite size and unbounded extent, as properties of space, was indicated in the words:
"In the extension of space-construction to the infinitely great, we must distinguish between unboundedness and infinite extent; the former belongs to the descriptive category, the latter to the metrical. That space is an unbounded threefold manifoldness is an assumption that is developed by every conception of the outer world.... The unboundedness of space possesses... a greater empirical certainty than any external experience. But its infinite extent by no means follows from this; on the contrary, if we assume bodies independent of position, and therefore ascribe to space constant curvature, it must necessarily be finite, provided this curvature has ever so small a value."
The expression curvature of space is an unfortunate metaphor, derived from the analogy between the elliptic geometry of the plane and the parabolic geometry of surfaces of uniform curvature. The plane is not curved for the elliptic, nor for the hyperbolic hypothesis. Considerable misconception has arisen in this way. The study of Beltrami's analogies rectifies such an error.
Riemann further noted the conceivable heterogeneity of space. The space-constant may be different in different places. It may also vary with the time.
CAYLEY
Sixth Memoir upon Qualities, London, 1859.
Cayley developed an analytical theory of Metric which could be coordinated to the three hypotheses which constitute the geometry of a homogeneous space. The hypotheses presented themselves as the three cases when the straight line has no, one, or two real points at infinite distance from all other points on itself. These three cases are allied to the hypotheses of no, one, or two parallels from a given point to the straight line.
VON HELMHOLTZ
The Essential Principles of Geometry, 1866, 1868.
Von Helmhoitz endeavoured to give new and precise expression to the Axioms or Postulates upon which a science of spatial relationships could be constructed, e.g. perfectly free moveability of rigid bodies. The whole of the ground has been gone over with great thoroughness by succeeding Geometers, notably Lie. The final results of these labours have been summarised by Dr Whitehead in his Tracts.
BELTRAMI
Attempt to interpret non-Euclidean Geometry, 1868.
Beltrami's Saggio, of which a French translation was made by Hoüel {Annales de I' Ecole Normale Superieure, Vol. 6, pages 251-288}, is of supreme importance in the development of the Science of Space. The Italian Geometer proved conclusively the right of the elliptic and hyperbolic hypotheses to rank equally with the Euclidean system as theories of a homogeneous space; and, empirically, they are clearly superior.
Beltrami showed this by pointing out that all the elliptic (and hyperbolic) geometry of the plane was characterised by the same metrical relationships as hold good in the parabolic geometry of surfaces of uniform positive (and negative) curvature.
Any flaw in the former would necessarily be accompanied by a flaw in the latter. If there is no flaw in the Euclidean geometry of geodesics on a surface of uniform curvature, then there can be no flaw in the metabolic geometry of straight lines on a plane surface, for the metrical relationships are identical.
This conclusive argument can scarcely be refuted; Poincare does not meet it in his La Science et l’ Hypothese. It was therefore Beltrami's labours which first established Non-Euclidean Geometry on the firm foundation whereon it rests to-day, despite every kind of prejudice and misconception which it has encountered hitherto.
CLIFFORD
The Space-Theory of Matter, 1870.
Somewhat beyond theories of parallelism, but suggestive like everything else of his, the fragment of Clifford's here re-produced shows the freedom of the Geometer released from the fetters of traditionalism. It is chosen from a paper embodied in his Collected Works (page 22).
"I wish here to indicate a manner in which these speculations (of Riemann's) may be applied to the investigation of physical phenomena. I hold in fact:
(1) That small portions of space are of a nature analogous to little hills on a surface which is on the average flat; namely, that the ordinary laws of geometry are not valid in them.
(2) That this property if being curved or distorted is continually passed on from one portion of space to another after the manner of a wave.
(3) That this variation of the curvature if space is what really happens in that phenomenon which we call the motion of matter whether ponderable or ethereal.
(4) That in the physical world nothing else takes place but this variation, subject, possibly, to the law of continuity.
"I am endeavouring in a general way to explain the laws of double refraction on this hypothesis, but have not yet arrived at any results sufficiently decisive to be communicated."
The boldness of this speculation is surely unexcelled in the history of thought. Up to the present, however, it presents the appearance of an Icarian flight.
KLEIN
Ueber die sogenannte nicht-Euklidische Geometrie, 1871.
"The three Geometries have been called hyperbolic, elliptic, and parabolic, respectively, according as the two infinitely distant points of the straight line are real, imaginary, and coincident" (see page 47 above).
"All spatial metric rests upon two fundamental problems, as we know: the determination of the distance of two points and of the inclination of two straight lines."
Klein followed Riemann and Cayley in the use of coordinates to define position, and deduced formulae for lengths and angles from the first principles suggested above. The results, that length and angle are proportional to the logarithms of certain an-harmonic ratios estimated with reference to an Absolute formed of infinitely distant elements, cannot be assessed by Euclidean standards, but belong to a higher sphere of research than is here explored.
NEWCOMB
Elementary Theorems relating to the Geometry of a Space of three Dimensions and of uniform positive Curvature in the fourth Dimension, 1877.
The American Astronomer first assumed the homogeneity of space (Grelles Journal, Vol.83, pages 293-299). Then:
"2. I assume that this space is affected with such curvature that a right line shall always return into itself at the end of a finite and real distance '2D, without losing, in any part of its course, that symmetry with respect to space on all sides of it which constitutes the fundamental property of our conception of it."
This definition of rectilinearity and the assumption of finitude are faultless; but no more needs to be assumed. Newcomb might now have proved that area/divergence = 4D2/PI2
DODGSON
A New Theory of Parallels, London, 1895.
The amiable author of Alice in Wonderland contributed to the Theory of Parallelism a pretty substitute for the Fifth Postulate, as follows:
"In every circle, the inscribed equilateral tetragon is greater than any one of the segments which lie outside it."
Dodgson's Axiom was aimed at the exclusion of the hyperbolic hypothesis, in which the assertion is not universally correct.
https://archive.org/details/theoriesofparall00fran
THEORIES OF PARALLELISM AN HISTORICAL CRITIQUE
BY WILLIAM BARRETT FRANKLAND, M.A.
SOMETIME FELLOW OF CLARE COLLEGE, CAMBRIDGE: VICAR OF WRAWBY, 1910
Edited by Vortexpuppy
Euclid
(A) Necessary concessions
1) Let it be conceded that from every point to every point a straight line can be drawn;
(2) And a limited straight line can be produced continually in a straight line
(3) And for every centre and distance a circle can be described;
(4) And all right angles are equal to each other;
(5) And if a straight line falling on two straight lines make the angles within and towards the same parts less than two right angles, then the two straight lines being indefinitely produced meet towards the parts where are the angles less than two right angles,
(B) Universal Ideas
1) Equals to the same are also equal to each other
2) And if to equals, equals are added, the whole are equal;
3) And if from equals, equals are subtracted, the remainders are equal;
4) And things coinciding with each other are equal to each other;
5) And the whole is greater than the part.
POSIDONIUS
(lost work on Geometry, B.C. 80)
"Posidonius says that parallel lines are such as neither converge nor diverge in one plane, but have all the perpendiculars drawn from points of one to the other equal. On the other hand such straight lines as make their perpendiculars continually greater or less will meet somewhere or other, because they converge towards each other"
GEMINUS
(Doctrine of Mathematics, B.C: 70)
"We learned from the very pioneers of the Science never to allow our minds to resort to weak plausibility’s for the advancement of geometrical reasoning
AGANIS
"If two straight lines are equidistant, the space between them is perpendicular to each of these lines."
PROCLUS
Commentary on the First book of the elements, A.D. 450
"It cannot be asserted unconditionally that straight lines produced from less than two right angles do not meet. It is of course obvious that some straight lines produced from less than two right angles do meet, but the (Euclidean) theory would require all such to intersect. But it might be urged that as the defect from two right angles increases, the straight lines continue asecant up to a certain magnitude of the defect, and for a greater magnitude than this they intersect.”
"Parallelism is similarity of position, if one may so say"
GERBERT
Mathematical works, A.D. 1000
"Two straight lines distant from each other by the same space continually, and never meeting each other when indefinitely produced, are called parallel, that is, equidistant."
BILLINGSLEY
The elements of geometrie A.D. 1570
"Parallel or equidistant right lines are such; which being in one and the selfe same superficies, and produced infinitely on both sydes, do never in any part concurre."
TACQUET
Elementa Geometriae Planae et Solidae, Amsterdam, 1654.
(11) Parallel lines have a common perpendicular,
(12) Two perpendiculars cut off equal segments from each of two parallels.
"Their truth is immediately apparent from the definition of parallelism."
HOBBES
De Corpore, 1655 : et caetera.
"There is in Euclid a definition of strait-lined parallels but I do not find that parallels in general are anywhere defined and therefore for a universal definition of them, I say that any two lines whatsoever, strait or crooked, as also any two superficies, are parallel, when two equal strait lines, wheresoever they fall upon them make equal angles with each of them.
From which definition it follows; first, that any two strait lines, not inclined opposite ways, falling upon two other strait lines, which are parallel, and intercepting equal parts in both of them, are themselves also equal and parallel" (De Corpore, c. 14, § 12).
“How shall a man know that there be strait lines which shall never meet though both ways infinitely produced?” {Collected Works, Vol. 7, page 206}.
WALLIS
Demonstratio Postulati Quinti Euclidis, 1663.
"It is known," he began, "that some of the ancient geometers, as well as the modern, have censured Euclid for having postulated, as a concession required without demonstration, the Fifth Postulate, or (as others say) the Eleventh Axiom, or, with the enumeration of Clavius, the Thirteenth Axiom... But those who discover this fault in Euclid do themselves very often (at least, as far as I have examined them) make other assumptions in place of it, and these appear to me no easier to allow than what Euclid postulates... Since nevertheless I observe that so many have attempted a proof, as if they esteemed it necessary, I have thought good to add my own effort, and to endeavour to bring forward a proof that may be less open to objection than theirs."
Wallis laid down 7 simple lemmas and then his radical 8th.
“VIII. At this stage, presupposing a knowledge of the nature of ratio and the definition of similar figures, I assume as an universal idea : To any given figure whatever, another figure, similar and of any size, is possible. Because continuous quantities are capable both of illimitable division and illimitable increase, this seems to result from the very nature of quantity; namely, that a figure can be continuously diminished or increased illimitably, the form of the figure being retained.”
LEIBNIZ
Characteristica Geometrica, 1679
"This definition seems rather to describe parallels by means of a more remote property than that which they most evidently display and one might doubt whether the relationship exists, or whether all straight lines in the plane do not ultimately intersect each other " (page 200).
DA BITONTO
Euclide Mestituto, 1680.
“The perpendiculars let fall from points of any curve upon any straight line cannot all be equal.”
SACCHERI
Euclides ah omni naevo Vivdicatus, Milan, 1733.
Saccheri protested against the procedure of early Geometers who "assume, not without great violence to strict logic, that parallel lines are equally distant from each other, as though that were given a priori, and then pass on to the proofs of the other theorems connected therewith" (page 46).
SIMSON
Euclidis elementorwn lihri priores sea;... Glasgow, 1756.
Definition 1. The distance of a point from a straight line is the perpendicular from the point upon the straight line.
Definition 2. A straight line is said to approach towards, or recede from, another straight line according as the distances of points of the former from the latter decrease or increase. Two straight lines are equidistant, if points of the one preserve the same distance from the other.
Axiom: A straight line cannot approach towards, and then recede from, a straight line without cutting it; nor can a straight line approach towards, then be equidistant to, and then recede from, a straight line; for a straight line preserves always the same direction.”
LAMBERT
Die Theorie der Parallel-linien, 1766.
These are again the parabolic, elliptic and hyperbolic hypotheses of Dr Klein. The elliptic hypothesis (ii) soon dropped out of Lambert's hands, owing to his extension to the entire (infinite) plane of results into which Euc. I. 16 enters. But the hyperbolic hypothesis (iii) was successfully worked out to conclusions implying an absolute standard of length. On this Lambert remarked (§§ 80, 81): "This consequence possesses a charm which makes one desire that the Third Hypothesis be indeed true”.
"Yet on the whole I would not wish it true, notwithstanding this advantage (of an absolute standard of length), since innumerable difficulties would be involved therewith. Our trigonometrical tables would become immeasurably vast (compare pages xviii and 57, above and below); the similitude and proportionality of geometrical figures would wholly disappear, so that no figure can be represented except in its actual size astronomy would be harassed (see our Second Additional Note); etc.
“Still these are “argumenta ah amove et invidid ducta”, which must be banished from Geometry as from every science.”
"I revert accordingly to the Third Hypothesis. In this hypothesis, as already seen, not only is the sum of the three angles of every triangle less than 180°; but this difference from 180° increases directly with the area of the triangle; that is to say, if of two triangles one has a greater area than the other, then in the first the sum of the three angles is less than in the second."
BERTRAND
Developpement Nouveau de la Partie EUmentaire des Mathematiques, Geneva, 1778.
"Geometry, like every other science, has its roots in ideas common to all. From this fount of ideas, the first originators derived those principles and germs of knowledge which they bestowed upon mankind. Hence it appears that in every science two parts can be distinguished; the first, consisting of the assemblage of principles or primitive conceptions from which the science proceeds; and a second, comprising the development of the consequences of the principles. In respect of these principles as they exist in every mind, science would seem to encounter no resistance or difficulty. Yet the choice that has to be made of first principles, the degree of simplicity and elegance to which they have to be reduced, and the necessity of enunciating them in precise terms, capable of clear comprehension, all this is very difficult."
"The spectacle of the universe displays before our eyes an immense space. In this immensity bodies exist and change continually their shape, size and position; and meanwhile space itself, invariable in all its parts, remains like a sea always calm, in everlasting repose. So the idea we form of space is that it is infinite and limitless; homogeneous and like itself at every time and in every place. Space is without bounds, for any we might assign to it would be contained in it, and therefore would not bound it. Space is homogeneous, in that the portion of space occupied by a body in one plane would not differ from that which would be occupied by it elsewhere."
Division of space into two halves identical in all but position gives the plane surface; and division of the plane similarly gives the straight line (so also Leibniz, above, page 19).
If there is no court of appeal from the verdict of "common sense," Bertrand's argument stands, and Euclid's geometry prevails.
PLAYFAIR
Elements of Geometry, Edinburgh, 1795.
"Two straight lines which intersect one another, cannot be both parallel to the same straight line."
Of this Axiom of Playfair's, Cayley said: "My own view is that Euclid's Twelfth Axiom in Playfair's form of it, does not need demonstration, but is part of our notion of space, of the physical space of our experience, which is the representation lying at the bottom of all external experience" {Presidential Address to the Britisli Association, 1883}. Dr Russell cites and criticises this interesting confession in his admirable Foundations of Geometry (Cambridge, 1899, page 41).
LAPLACE
Exposition du Systeme du Monde, Paris, 1796.
"The law of attraction, inversely as the square of the distance, is that of emanations which proceed from a centre. One of its remarkable properties is, that if the dimensions of all the bodies in the universe, their mutual distances and velocities, increase or diminish proportionally, they describe curves entirely similar to those which they at present describe; so that if the universe be successively reduced to the smallest imaginable space, it will always present the same appearance to all observers... The simplicity of the laws of nature therefore only permits us to observe the relative dimensions of space."
The translator's note (page 536) may be reproduced here
"The endeavours of Geometers to demonstrate Euclid's Twelfth Axiom about parallel lines have been hitherto unsuccessful. However, no person questions the truth of this Axiom, or of the Theorems which Euclid has deduced from it.
The perception of extension contains therefore a peculiar property which is self-evident, without which we could not rigorously establish the doctrine of parallels. The notion of a limited extension (for example, of a circle) does not involve anything that depends on its absolute magnitude; but if we conceive its radius to be diminished, we are forced to diminish in the same proportion its circumference, and the sides of all the inscribed figures. This proportionality was, according to Laplace, much more obvious than that of Euclid.
GAUSS
Letters and Reviews, 1799-1846.
"I have arrived at much which most people would regard as proved, but it is in my eyes good for nothing in this respect. For example, if it could be proved that a rectilinear triangle is possible, of area exceeding any assigned area, I should be in a position to prove rigorously the whole of (Euclidean) geometry. Now most people would regard this as axiomatic, but I do not. It would be quite possible that however distant from each other the vertices of the triangle were assumed to lie in space, the area should still be less than an assignable limit.
I have more propositions of a similar character, but in none of them do I find anything really satisfying."
Thus Gauss seems to have worked out several fundamental theorems of hyperbolic geometry but not so completely as to feel ready to publish them. His letter to Taurinus, a facsimile of which forms the frontispiece to Parallellinien von Euklid his auf Gauss, reads as follows:
"I have read not without pleasure your kind letter of October 30th with the small sketch enclosed, the more so because I have been accustomed to discover scarcely any trace of pure geometrical spirit among the majority of people who make the new attempts on the so-called Theory of Parallel Lines. With reference to your attempt I have nothing, or not much, to observe except that it is incomplete. Your presentation of the proof that the sum of the three angles of a plane triangle cannot be greater than 180° leaves much to be desired in respect of geometrical rigor. This in itself could be remedied, and beyond all doubt the impossibility can be proved most rigorously. Things stand otherwise in the second part, that the sum of the angles cannot be less than 180°; this is the crucial point, the reef on which all the wrecks take place.
I imagine that you have not been long occupied with this subject. My own interest in it has extended over 30 years, and I do not think that anyone can have occupied himself more with this second part than I, although I have never published anything on it. The assumption that the sum of the three angles of a triangle is less than 180° leads to a peculiar Geometry entirely different from ours, a geometry completely self-consistent, which I have developed for myself perfectly satisfactorily, so that I can solve any problem in it with the assumption that a constant is determinate, this constant not being capable of a priori specification.
The greater this constant is assumed to be, the more nearly is Euclidean Geometry approached; and an infinite value of the constant makes the two systems coincide. The theorems of this geometry seem somewhat paradoxical, and to the lay mind absurd; but continued steady reflection shows them to contain nothing at all impossible.
Thus, for instance, the three angles of a triangle can be as small as we please, if only the sides are taken sufficiently great; and yet the area of a triangle can never exceed a definite limit, however great the sides are taken to be, and indeed can never reach it.
All my efforts to discover a contradiction, an inconsistency, in this non- Euclidean Geometry have been unsuccessful; and the one thing in it contrary to our conceptions is that, were the system true, there must exist in space a linear magnitude, determined for itself albeit unknown to us.
But methinks, despite the say- nothing word-wisdom of the metaphysicians, we know far too little, too nearly nothing, about the true nature of space, for us to confuse what has an unnatural appearance with what is absolutely impossible.
If the non-Euclidean Geometry were true, and that constant at all comparable with such magnitudes as lie within reach of our measurements on the earth or in the heavens, it could be determined a posteriori.
Hence I have sometimes expressed in jest the wish that the Euclidean Geometry were not true, since then we should possess an absolute standard of measurement a priori. I am not afraid that a man who has shown himself to possess a thoughtful mathematical mind will misunderstand the foregoing; but in any case, please regard this as a private communication, of which public use, or use leading to publicity, is not to be made in any way. If at some future time I acquire more leisure than in my present circumstances, I shall perhaps publish my investigations.”
CARNOT
Geometrie de Position, Paris, 1803.
Page 59
Carnot advocated the Principle of Similitude as an alternative to the Parallel-Postulate, a view-point secured with greater elaboration by Wallis (above, page 18). He wrote (§ 435):
"The Theory of Parallels rests on a primitive idea which seems to me almost of the same degree of clearness as that of perfect equality or of superposition. This is the idea of Similitude. It seems to me that we may regard as a principle of the first rank that what exists on a large scale, as a ball, a house, or a picture, can be reduced in size, and vice versa and that consequently, for any figure we please to consider, it is possible to imagine others of all sizes similar to it; that is to say, such that all their dimensions continue to be in the same proportions. This idea once admitted, it is easy to establish the Theory of Parallels without resorting to the idea of infinity.
W. BOLYAI
Theoria Parallelarum, Maros-Yasarheli, 1804 Kurzer Grundriss, 1851.
In the second tract was introduced an ingenious substitute for the Parallel-Postulate: Let it be conceded that "if three points are not in a straight line, then they lie on a sphere": and therefore on a circle.
Like Lobachewski, the younger Bolyai only elaborated a hyperbolic geometry. They both however discussed the properties of curves (X-lines, horocycles) neither rectilinear nor circular, whereof the normals are parallel. Only in Euclidean Geometry is the straight line the limit of a circle of indefinitely increased radius.
THIBAUT
Grundriss der reinen Mathematik, Gottingen, 1809.
Any treatment of parallelism based upon the idea of direction assumes that translation and rotation are independent operations, and this is only so in Euclidean Geometry. The following is the easiest and most plausible way of establishing the parabolic hypothesis. From Euc.i. 32 can be deduced very readily the Euclidean theory of parallelism. Thibaut argued for this theorem.
" Let ABC be any triangle whose sides are traversed in order from A along AB, BG, GA. While going from A to B we always gaze in the direction ABb (AB being produced to b), but do not turn round. On arriving at B we turn from the direction Bb by a rotation through the angle bBG, until we gaze in the direction BGc. Then we proceed in the direction BGc as far as G, where again we turn from Gc to GAa through the angle cGA ; and at last arriving at A, we turn from the direction Aa to the first direction AB through the external angle aAB. This done, we have made a complete revolution, just as if, standing at some point, we had turned completely round ; and the measure of this rotation is 2PI. Hence the external angles of the triangle add up to 2PI, and the internal angles A+B+G = PI. Q.E.D."
TAURINUS
Theorie der Parallellinien, 1825.
Taurinus expressed eight objections to the wider range of Geometry then being manifested (Engel and Stackel, pages 208-9).
"1. It contradicts all intuition. It is true that such a system would in small figures present the same appearance as the Euclidean; but if the conception of space is to be regarded as the pure form of what is indicated by the senses, then the Euclidean system is incontestably the true one, and it cannot be assumed that a limited experience could give rise to actual illusion."
None the less, however, measurement of angles, if sufficiently exact, might deal a fatal blow to Euclid's a priori system, by subverting Euc. i. 32.
" 2. The Euclidean system is the limit of the first system, wherein the angles of a triangle are more than two right angles. With this procedure to the limit, the paradox in connexion with the axiom of the straight line ceases."
"In this theorem (51) it is proved that with the assumption that the angle-sum of a quadrilateral can be greater than four right angles (or, what comes to the same thing, if the angle-sum of a triangle can be greater than two right angles), then all the lines, perpendicular to another line, intersect in two points at equal distance on either side. Hence arises the evident contradiction of the axiom of the straight line, and so such a geometrical system cannot be rectilinear."
" 3. Were the third system the true one, there would be no Euclidean Geometry, whereas however the possibility of the latter cannot be denied."
"4. In the assumption of such a system as rectilinear, there is no continuous transition; the angles of a triangle could only make more or less than two right angles."
The Euclidean system, nevertheless, is the connecting link desired between the elliptic and hyperbolic geometries. It is the limit of each; whether it separates them or unites them is only a matter of words.
" 5. These systems would have quite paradoxical consequences, contradicting all our conceptions; we should have to assign to space properties it cannot have,"
This appeal to common sense is of value in practical work, where close approximation suffices.
" 6. All complete similarity of surfaces and bodies would be wanting; and still this idea seems to have its roots in intuition, and to be a true postulate."
The view of some of the world's greatest thinkers, and they have Wallis for spokesman.
" 7. The Euclidean system is in any case the most complete, and its truth therefore possesses the greatest plausibility."
But all three hypotheses together supply a more complete Theory of Space than any single one of them, and their common basis is the assumption that space is homogeneous. More complete still will be the Geometry of the future, contemplating a heterogeneous space (compare Clifford's speculation below, page 49).
" 8. The internal consistency of the third system is no reason for regarding it as a rectilinear system; however, there is in it, so far as we know, no contradiction of the axiom of the straight line as in the first."
These objections are mainly of an a priori character. On the other hand, our knowledge of space as an objectivity is small.
Increased precision of astronomical instruments might display antipodal images of a few bright stars, and this would then tell in favor of an elliptic hypothesis. The space-constant is large, very large; but no experiment can ever prove it infinite, as Euclideans assume it.
J. BOLYAI
Appendix scientiara spatii eochibens...Maros-YsksarheM, 1832.
Starting from the strict definition of a parallel as the limiting position of a secant, Bolyai proceeded to solid geometry, and deduced the existence of L-lines and F-surfaces (called by Lobachewski horocycles and horospheres), which are the limiting forms of circles and spheres of infinite radius in hyperbolic space. He found Euclidean geometry to obtain for horocycles drawn on horospheres; but for rectilinear triangles drawn on a plane, he proved that the area was proportional to the supplement of the angle-sum (§ 43). The work merits careful study, and comparison with the corresponding work of Lobachewski,
LEGENDRE
Reflexions sur...la Theorie des ParaUeles, Paris, 1833.
"After some researches undertaken with the aim of proving directly that the sum of the angles of a triangle is equal to two right angles, I have succeeded first in proving that this sum cannot be greater than two right angles. Here is the proof as it appeared for the first time in the 3rd edition of my Geometry published in 1800."
i.e. the straight line is not the shortest distance between the two points Qi and Qn+1
The solution of this enigma is that the elliptic hypothesis was actually assumed; and for this hypothesis, the straight line is re-entrant, and Qn+1 may fall, for instance, between Q1 and Q2.
Legendre then proceeded further..
"The first proposition being established, it remained to prove that the sum of the angles cannot be less than two right angles; but we must confess that this second proposition, though the principle of its proof was well-known (see Note ii, page 298, of the 12th Edition of the Elements of Geometry), has presented difficulties which we have not been able entirely to clear away.
This it is which caused us, in the 9th Edition, to return to Euclid's procedure; and later, in the 12th, to adopt another method of proof to be spoken of hereafter.... “It is doubtless due to the imperfection of popular language, and the difficulty of giving a good definition of the straight line, that Geometers have hitherto achieved little success, when they endeavored to deduce this Theorem (Euc. i. 32) from ideas purely based on the equality of triangles contained in the First Book of the Elements."
The notion that the definition of the straight line was in some way the nodus of the Theory of Parallelism had evidently occurred to Taurinus and others, and was expressed as a conviction by Dodgson in his New Theory of Parallels (1895).
Legendre next gave an ingenious and elaborate proof that if the angle-sum of one triangle in the plane is PI, the angle-sum of every triangle is PI. He observed further that if the angle-sum of a triangle were greater (less) than PI, then equi-distants would be convex (concave) to their bases; but failed to make cogent use of this apparent paradox, as it then was.
LOBACHEWSKI
Geometrische Untersuchungen zur Theorie der Parallel-linien, Berlin, 1840.
As Dr Whitehead has said in one of his tracts
"Metrical Geometry of the hyperbolic type was first discovered by Lobachewski in 1826, and independently by J. Bolyai in 1832, This discovery is the origin of the modern period of thought in respect of the foundations of Geometry " {Axioms of Descriptive Geometry, page 71}. The Geometrische Untersuchungen has been done into English by Halsted (Austin, Texas, 1891). Characterising Legendre's efforts as fruitless, the Russian Geometer went on to say:
"My first essay on the foundations of Geometry was published in the Kasan Messenger for the year 1829.... I will here give the substance of my investigations, remarking that, contrary to the opinion of Legendre, all other imperfections, for instance, the definition of a straight line, are foreign to the argument, and without real influence upon the Theory of Parallels."
Then followed a number of simple theorems, independent of any particular theory of parallelism, although the third presents the appearance of contradicting the elliptic hypothesis, thus
"A straight line sufficiently prolonged on both sides proceeds beyond every limit (liber jede Grenze), and so separates a limited plane into two parts."
The ninth of Lobachewski's preliminaries, however, did distinctly assume a theorem not universally valid for the elliptic hypothesis
"In the rectilinear triangle, a greater angle lies opposite the greater side."
The sixteenth step inaugurated the Hyperbolic Hypothesis
"16. All straight lines issuing from a point in a plane can be divided, with reference to a given straight line in this plane, into two classes, namely, secant and asecant. The limiting straight line between one class and the other is called parallel to the given straight line."
Thus if MH is a parallel to NB on one side of the perpendicular MN let fall from M upon NB, then the angle HMN was called the angle of parallelism. Let the length of MN be p, and denote by W the corresponding angle of parallelism HMN, then Lobachewski established rigorously the very curious result:
The complement of W is the Gudermannian of p/K, where K is constant over the plane. Thus the angle of parallelism was determined in all cases; and Lobachewski's result was identical with that obtained above on page xviii.
Lobachewski's proof was intricate, however, and involved the horocycles and horospheres mentioned already.
Very elegant is Lobachewski's application of the construction of Euc. I. 16 (itself a pattern of elegance) to prove that the angle-sum of a triangle cannot exceed two right angles.
There is nevertheless no serious difficulty, provided space is of finite size, as Riemann suggested later.
MEIKLE
Theory of Parallel Lines, Edinburgh, 1844.
"During the long succession of ages which have elapsed since the origin of Geometry, many attempts have been made and treatises written, though with little success, to demonstrate the important Theorem which Euclid, having failed to prove, has styled his 12th Axiom, and which is nearly equivalent to assuming that the three angles of every triangle amount to two right angles."
Except that the elliptic hypothesis is not excluded by the Parallel-Postulate, one might say "exactly equivalent."
By the aid of this magnificent piece of reasoning, Meikle proved that the area of a triangle is proportional to what has been called its divergence; but he rejected the hyperbolic hypothesis on the ground that it involved triangles of finite area with zero angles - the paradox which aroused Gauss' interest (above, page 33).
RIEMANN
Habilitationsrede, 1854.
Riemann's brief but brilliant and epoch-making Essay was translated by Clifford {Collected Papers, page 56}. The possible combination of finite size and unbounded extent, as properties of space, was indicated in the words:
"In the extension of space-construction to the infinitely great, we must distinguish between unboundedness and infinite extent; the former belongs to the descriptive category, the latter to the metrical. That space is an unbounded threefold manifoldness is an assumption that is developed by every conception of the outer world.... The unboundedness of space possesses... a greater empirical certainty than any external experience. But its infinite extent by no means follows from this; on the contrary, if we assume bodies independent of position, and therefore ascribe to space constant curvature, it must necessarily be finite, provided this curvature has ever so small a value."
The expression curvature of space is an unfortunate metaphor, derived from the analogy between the elliptic geometry of the plane and the parabolic geometry of surfaces of uniform curvature. The plane is not curved for the elliptic, nor for the hyperbolic hypothesis. Considerable misconception has arisen in this way. The study of Beltrami's analogies rectifies such an error.
Riemann further noted the conceivable heterogeneity of space. The space-constant may be different in different places. It may also vary with the time.
CAYLEY
Sixth Memoir upon Qualities, London, 1859.
Cayley developed an analytical theory of Metric which could be coordinated to the three hypotheses which constitute the geometry of a homogeneous space. The hypotheses presented themselves as the three cases when the straight line has no, one, or two real points at infinite distance from all other points on itself. These three cases are allied to the hypotheses of no, one, or two parallels from a given point to the straight line.
VON HELMHOLTZ
The Essential Principles of Geometry, 1866, 1868.
Von Helmhoitz endeavoured to give new and precise expression to the Axioms or Postulates upon which a science of spatial relationships could be constructed, e.g. perfectly free moveability of rigid bodies. The whole of the ground has been gone over with great thoroughness by succeeding Geometers, notably Lie. The final results of these labours have been summarised by Dr Whitehead in his Tracts.
BELTRAMI
Attempt to interpret non-Euclidean Geometry, 1868.
Beltrami's Saggio, of which a French translation was made by Hoüel {Annales de I' Ecole Normale Superieure, Vol. 6, pages 251-288}, is of supreme importance in the development of the Science of Space. The Italian Geometer proved conclusively the right of the elliptic and hyperbolic hypotheses to rank equally with the Euclidean system as theories of a homogeneous space; and, empirically, they are clearly superior.
Beltrami showed this by pointing out that all the elliptic (and hyperbolic) geometry of the plane was characterised by the same metrical relationships as hold good in the parabolic geometry of surfaces of uniform positive (and negative) curvature.
Any flaw in the former would necessarily be accompanied by a flaw in the latter. If there is no flaw in the Euclidean geometry of geodesics on a surface of uniform curvature, then there can be no flaw in the metabolic geometry of straight lines on a plane surface, for the metrical relationships are identical.
This conclusive argument can scarcely be refuted; Poincare does not meet it in his La Science et l’ Hypothese. It was therefore Beltrami's labours which first established Non-Euclidean Geometry on the firm foundation whereon it rests to-day, despite every kind of prejudice and misconception which it has encountered hitherto.
CLIFFORD
The Space-Theory of Matter, 1870.
Somewhat beyond theories of parallelism, but suggestive like everything else of his, the fragment of Clifford's here re-produced shows the freedom of the Geometer released from the fetters of traditionalism. It is chosen from a paper embodied in his Collected Works (page 22).
"I wish here to indicate a manner in which these speculations (of Riemann's) may be applied to the investigation of physical phenomena. I hold in fact:
(1) That small portions of space are of a nature analogous to little hills on a surface which is on the average flat; namely, that the ordinary laws of geometry are not valid in them.
(2) That this property if being curved or distorted is continually passed on from one portion of space to another after the manner of a wave.
(3) That this variation of the curvature if space is what really happens in that phenomenon which we call the motion of matter whether ponderable or ethereal.
(4) That in the physical world nothing else takes place but this variation, subject, possibly, to the law of continuity.
"I am endeavouring in a general way to explain the laws of double refraction on this hypothesis, but have not yet arrived at any results sufficiently decisive to be communicated."
The boldness of this speculation is surely unexcelled in the history of thought. Up to the present, however, it presents the appearance of an Icarian flight.
KLEIN
Ueber die sogenannte nicht-Euklidische Geometrie, 1871.
"The three Geometries have been called hyperbolic, elliptic, and parabolic, respectively, according as the two infinitely distant points of the straight line are real, imaginary, and coincident" (see page 47 above).
"All spatial metric rests upon two fundamental problems, as we know: the determination of the distance of two points and of the inclination of two straight lines."
Klein followed Riemann and Cayley in the use of coordinates to define position, and deduced formulae for lengths and angles from the first principles suggested above. The results, that length and angle are proportional to the logarithms of certain an-harmonic ratios estimated with reference to an Absolute formed of infinitely distant elements, cannot be assessed by Euclidean standards, but belong to a higher sphere of research than is here explored.
NEWCOMB
Elementary Theorems relating to the Geometry of a Space of three Dimensions and of uniform positive Curvature in the fourth Dimension, 1877.
The American Astronomer first assumed the homogeneity of space (Grelles Journal, Vol.83, pages 293-299). Then:
"2. I assume that this space is affected with such curvature that a right line shall always return into itself at the end of a finite and real distance '2D, without losing, in any part of its course, that symmetry with respect to space on all sides of it which constitutes the fundamental property of our conception of it."
This definition of rectilinearity and the assumption of finitude are faultless; but no more needs to be assumed. Newcomb might now have proved that area/divergence = 4D2/PI2
DODGSON
A New Theory of Parallels, London, 1895.
The amiable author of Alice in Wonderland contributed to the Theory of Parallelism a pretty substitute for the Fifth Postulate, as follows:
"In every circle, the inscribed equilateral tetragon is greater than any one of the segments which lie outside it."
Dodgson's Axiom was aimed at the exclusion of the hyperbolic hypothesis, in which the assertion is not universally correct.
vortexpuppy- Posts : 167
Points : 3602
Reputation : 296
Join date : 2015-12-30
 Re: Direct Vision, Rationality, Realism and Common Sense.
Re: Direct Vision, Rationality, Realism and Common Sense.
VP Health Warning about Right Lines, Parallelism and Perspective :-)
Do not let trolls and shills engage you in debates about "measuring stuff" without agreeing on the underlying assumptions and definitions. It is bad for your health, wastes your time & effort and never reaches a conclusion.
Just because a model is a good approximation, it does not make it real.
Others may pretend that this has no relevance, or refuse to question the foundations of mathematics, gladly losing themselves in theories, equations and numbers, the origins of which they do not understand or willfully ignore.
Nobody can ever (again) convince me of a Space Ball using mathematics that assumes first principles that I deny.
Do not let trolls and shills engage you in debates about "measuring stuff" without agreeing on the underlying assumptions and definitions. It is bad for your health, wastes your time & effort and never reaches a conclusion.
Just because a model is a good approximation, it does not make it real.
Others may pretend that this has no relevance, or refuse to question the foundations of mathematics, gladly losing themselves in theories, equations and numbers, the origins of which they do not understand or willfully ignore.
Nobody can ever (again) convince me of a Space Ball using mathematics that assumes first principles that I deny.
vortexpuppy- Posts : 167
Points : 3602
Reputation : 296
Join date : 2015-12-30
 Re: Direct Vision, Rationality, Realism and Common Sense.
Re: Direct Vision, Rationality, Realism and Common Sense.
Some hangout archives that might be of interest to others.
Anybody is welcome to join. I usually try to participate on the regular Saturday meet (around 20:00 UK time)
The channel is Beyond the Imaginary Curve https://www.youtube.com/channel/UCvswlgeHodOejVN21TWweLw
Anybody is welcome to join. I usually try to participate on the regular Saturday meet (around 20:00 UK time)
The channel is Beyond the Imaginary Curve https://www.youtube.com/channel/UCvswlgeHodOejVN21TWweLw
vortexpuppy- Posts : 167
Points : 3602
Reputation : 296
Join date : 2015-12-30
 Re: Direct Vision, Rationality, Realism and Common Sense.
Re: Direct Vision, Rationality, Realism and Common Sense.
vortexpuppy wrote:
Some hangout archives that might be of interest to others.
Anybody is welcome to join.
I usually try to participate on the regular Saturday meet (around 20:00 UK time)
The channel is Beyond the Imaginary Curve https://www.youtube.com/channel/UCvswlgeHodOejVN21TWweLw
Thanks for the link, it's very nice to see that the majority of this panel is grounded in flat Earth Facts and not State Sponsored Fiction.

Schpankme- Posts : 1202
Points : 5961
Reputation : 1606
Join date : 2015-12-30
 Re: Direct Vision, Rationality, Realism and Common Sense.
Re: Direct Vision, Rationality, Realism and Common Sense.
vortexpuppy wrote:Do not let trolls and shills engage you in debates about "measuring stuff" without agreeing on the underlying assumptions and definitions. It is bad for your health, wastes your time & effort and never reaches a conclusion.
I had a couple of shills try and pull the old "distance" trick the other day over in the comments here (my name 'Chris P'):
https://www.youtube.com/watch?v=3qijp8jEfa0&lc=z13cfbbqlon5wrzkq222wxzgrtbjf1kmf
Funny thing is, before I provided irrefutable evidence of no curve, I had one of them do the math for the picture I posted. They both then ignored my evidence and tried to offer alternative "distance measuring" experiments along with the usual dose of ad homenims.
And as soon as I posted a link to IFERS, one of them warned others "not to click it because it set off his anti virus"...

csp- Posts : 424
Points : 4607
Reputation : 1054
Join date : 2016-01-04
Location : Australia
 Re: Direct Vision, Rationality, Realism and Common Sense.
Re: Direct Vision, Rationality, Realism and Common Sense.
Schpankme wrote:vortexpuppy wrote:
Some hangout archives that might be of interest to others.
Anybody is welcome to join.
I usually try to participate on the regular Saturday meet (around 20:00 UK time)
The channel is Beyond the Imaginary Curve https://www.youtube.com/channel/UCvswlgeHodOejVN21TWweLw
Thanks for the link, it's very nice to see that the majority of this panel is grounded in flat Earth Facts and not State Sponsored Fiction.
Sure. You are not alone in your disdain of fiction. Feel free to join. Or we can do our own hangout too if anybody is up for it....
vortexpuppy- Posts : 167
Points : 3602
Reputation : 296
Join date : 2015-12-30
 Re: Direct Vision, Rationality, Realism and Common Sense.
Re: Direct Vision, Rationality, Realism and Common Sense.
csp wrote:vortexpuppy wrote:Do not let trolls and shills engage you in debates about "measuring stuff" without agreeing on the underlying assumptions and definitions. It is bad for your health, wastes your time & effort and never reaches a conclusion.
I had a couple of shills try and pull the old "distance" trick the other day over in the comments here (my name 'Chris P'):
https://www.youtube.com/watch?v=3qijp8jEfa0&lc=z13cfbbqlon5wrzkq222wxzgrtbjf1kmf
Funny thing is, before I provided irrefutable evidence of no curve, I had one of them do the math for the picture I posted. They both then ignored my evidence and tried to offer alternative "distance measuring" experiments along with the usual dose of ad homenims.
And as soon as I posted a link to IFERS, one of them warned others "not to click it because it set off his anti virus"...
lol. I know exactly what you mean. I checked the comments. Usual appeal to authority of "great minds", some distractions about map projection and the appeal to mensuration: "if you don't understand how the measurements is evidence for the globe, you are the 5 year old."
Below is a mathematical definition of mensuration from a book by Halsted. This is already partly dumbed down imho, but serves at least as an example explanation of how mensuration is defined. The main point being that Standards, Units and Scales are arbitrarily CHOSEN to SUIT the PURPOSE of the INTENDED measurement.
Halsted, 1881 – "Metrical Geometry – An elementary treatise on mensuration."
“MENSURATION is that branch of mathematics which has for its object the measurement of geometrical magnitudes. It has been called, that branch of applied geometry which gives rules for finding the length of lines, the area of surfaces, and the volume of solids, from certain data of lines and angles. A magnitude is anything which can be conceived of as added to itself, or of which we can form multiples. The measurement of a magnitude consists in finding how many times it contains another magnitude of the same kind, taken as a unit of measure.
Measurement, then, is the process of ascertaining the ratio which one magnitude bears to some other chosen as the standard, and the measure of a magnitude is this ratio expressed in numbers. Hence, we must refer to some concrete standard, some actual object, to give our measures their absolute meaning. The concrete standard is arbitrary in point of theory, and its selection a question of practical convenience.”
vortexpuppy- Posts : 167
Points : 3602
Reputation : 296
Join date : 2015-12-30
 Re: Direct Vision, Rationality, Realism and Common Sense.
Re: Direct Vision, Rationality, Realism and Common Sense.
Here is my take down of Glober logic when appealing to "measurements" as proof.
A 2-Sphere cannot contain any water, being itself only a surface. The 3-Ball apparently has large bodies of water taking the shape of its exterior (held together by fictional forces). This is contrary to repeatable, observable, constant and uniform laws of nature. Water takes the shape of its container. This is an obvious fact on which we depend in all aspects of common daily life. To refute this is absurd to common reason.
Quantities can be measured in many ways depending on definitions and axioms. We should AVOID those methods of measurement based on CHOSEN axioms that are CONTRARY to self-evident truths.
- Mensuration of proper quantities (lines, surface, volumes, duration, number and ratio) is dependent on the underlying Geometry.
- Geometry itself is founded on man-made axioms and assumptions, especially those of straight and parallel lines
- Globers equate 2-Spheres (2D geometry of surfaces) with 3-Balls (3D geometry of solid bodies) because we/they were/are conditioned with globes and lied to incessantly about Space
- 2-Spheres and 3-Balls Geometry share certain equations and theorems making discernment difficult and confusing (mathematically).
- Surfaces and volumes are in fact different things that should never be equated in mathematical reasoning, and/or compared to each other.
A 2-Sphere cannot contain any water, being itself only a surface. The 3-Ball apparently has large bodies of water taking the shape of its exterior (held together by fictional forces). This is contrary to repeatable, observable, constant and uniform laws of nature. Water takes the shape of its container. This is an obvious fact on which we depend in all aspects of common daily life. To refute this is absurd to common reason.
Quantities can be measured in many ways depending on definitions and axioms. We should AVOID those methods of measurement based on CHOSEN axioms that are CONTRARY to self-evident truths.
vortexpuppy- Posts : 167
Points : 3602
Reputation : 296
Join date : 2015-12-30
 Re: Direct Vision, Rationality, Realism and Common Sense.
Re: Direct Vision, Rationality, Realism and Common Sense.
vortexpuppy wrote:
Here is my take down of Glober logic ... and mathematical reasoning
And there is always an excuse, anytime the Observer can see further across the Sphere than is mathematical possible.

Schpankme- Posts : 1202
Points : 5961
Reputation : 1606
Join date : 2015-12-30
 Some Thoughts Levelled at Rational Mensuration
Some Thoughts Levelled at Rational Mensuration
Some Thoughts Levelled at Rational Mensuration
The same deception is perpetrated on all of us. You live on a water-covered spinning ball that is not separated from a vacuous, expansive space. I laugh at the absurdity now, but it also makes me cringe in embarrassment. How easy it was to deceive me.
The fallacies and paradoxes that I entertain(ed), are exemplified by 1) That uncontained water can conform to the exterior surface of shapes where every direction is downhill, and 2) that no barrier is necessary to separate us from a vacuum.
I accepted these two paradoxes as being real. No questions asked. I allowed claims without proof to form a true belief. This is something I should never allow unless the claim is a self-evident fact. If I otherwise accept that there is no proof I can personally perform, or no experience that I can have to verify for myself, then I give up the right to judge for myself.
We should insist empirical proofs use quantities of measure that are properly defined, that they can be understood and applied by all people, and that can be evidenced by our common senses over extended durations of time. Nothing less than this should be deemed acceptable measureable proof.
If we allow surrogates and agents to dictate our beliefs, or allow ourselves to be media-ted by incessant fairy tales then we also have ourselves to blame for allowing it. That the fantasies are taken seriously by common people and academics often depends on 1) hidden or occulted knowledge, 2) sophistry in mathematical definitions and 3) an abuse of language 4) inattention to self-evident first principles.
If these are irreconcilable, then no common understanding or reasoning with others is possible, nor can conclusions and agreements be reached. You can never convince somebody of a mathematical proposition by demonstration if your proposition assumes as a first principle something which the other denies, or if you assume something in your reasoning that is for the other person, no less self-evident than the proposition that you are trying to prove to them.
In this respect we need to realise that the game is rigged. We are being thrown curve balls. They know things that we don’t, they define things we accept and they know better how we think than we do ourselves. We need to level that playing field.
We need to continue to expose the difference between acquired beliefs (e.g. globes) and self-evident truths (e.g. properties of water), between non sense and common sense, between idealism and direct reality. We must ensure we never lose or give away the authority to judge for ourselves, or allow the means thereof to be withheld from us (e.g. accepting the impossibility of scalable proofs unless you have a spare planet to play with)
The same deception is perpetrated on all of us. You live on a water-covered spinning ball that is not separated from a vacuous, expansive space. I laugh at the absurdity now, but it also makes me cringe in embarrassment. How easy it was to deceive me.
The fallacies and paradoxes that I entertain(ed), are exemplified by 1) That uncontained water can conform to the exterior surface of shapes where every direction is downhill, and 2) that no barrier is necessary to separate us from a vacuum.
I accepted these two paradoxes as being real. No questions asked. I allowed claims without proof to form a true belief. This is something I should never allow unless the claim is a self-evident fact. If I otherwise accept that there is no proof I can personally perform, or no experience that I can have to verify for myself, then I give up the right to judge for myself.
We should insist empirical proofs use quantities of measure that are properly defined, that they can be understood and applied by all people, and that can be evidenced by our common senses over extended durations of time. Nothing less than this should be deemed acceptable measureable proof.
If we allow surrogates and agents to dictate our beliefs, or allow ourselves to be media-ted by incessant fairy tales then we also have ourselves to blame for allowing it. That the fantasies are taken seriously by common people and academics often depends on 1) hidden or occulted knowledge, 2) sophistry in mathematical definitions and 3) an abuse of language 4) inattention to self-evident first principles.
If these are irreconcilable, then no common understanding or reasoning with others is possible, nor can conclusions and agreements be reached. You can never convince somebody of a mathematical proposition by demonstration if your proposition assumes as a first principle something which the other denies, or if you assume something in your reasoning that is for the other person, no less self-evident than the proposition that you are trying to prove to them.
In this respect we need to realise that the game is rigged. We are being thrown curve balls. They know things that we don’t, they define things we accept and they know better how we think than we do ourselves. We need to level that playing field.
We need to continue to expose the difference between acquired beliefs (e.g. globes) and self-evident truths (e.g. properties of water), between non sense and common sense, between idealism and direct reality. We must ensure we never lose or give away the authority to judge for ourselves, or allow the means thereof to be withheld from us (e.g. accepting the impossibility of scalable proofs unless you have a spare planet to play with)
vortexpuppy- Posts : 167
Points : 3602
Reputation : 296
Join date : 2015-12-30
 Re: Direct Vision, Rationality, Realism and Common Sense.
Re: Direct Vision, Rationality, Realism and Common Sense.
I posted a long comment in response to a question from a participant (Liam) in one of the recent street interviews.
I think it is a relevant summary to this thread and since this has been my home for a few years (and still is), I'll post it here, so you can all get to know me a little better. The same invitation extended to Liam, applies to everybody else here as well. Time permitting.
Look forward to bringing this deception to an end with you all. The quicker the fucking better.
My original comment to Liam
Hi Liam, good on you for doing the interview and coming here man. Great to see it. If only everybody was as open-minded as you and would start questioning. I'm Gav, the friend of John, whom he mentions in the interview (Maths/Physics from Glasgow Uni). If you have any questions don't hesitate to reach out. If I can give some advice for a newbie it would be this:
1) Continue to question everything and think for yourself. You will find many "beliefs" in your head, that are not your own (e.g. Space, Spinning balls, etc). Discard everything that has no basis in reality and evaluate from scratch. Don't believe anybody or anything you can't verify for yourself.
2) Don't get bogged down in "other theories or models" from many so-called flat earthers. Many are deliberate distractions to perpetuate division and strife. Alternative theories or models, should be equally dismissed if they have no proof other than mathematical ones. Mathematics is a formal science, like language. Definitions are neither true nor false, they are just definitions, so Maths is no proof of anything. This is also the reason why many clever people are duped and not that there is a conspiracy by all scientists around the world. They pull down their trousers to shite like the rest of us and are just as easily deceived (and heh, the maths works, so it must be right ;-) or indoctrinated into a belief system.
3) Concentrate on questioning obvious paradoxes, absurdities and contradictions to common sense and natural observations. You (and I) have every right to argue against a hypothesis (or theory) that contradicts the facts (e.g. level water), but to argue from a hypotheses against the facts is just bad natural philosophy / science. Your senses do not deceive you just because you don't fully understand how they work. (e.g. vision and perspective). If basic contradictions cannot be answered by modern day science, then something is horribly fucking wrong, and it isn't that all mankind is too dumb to understand.
4) The natural properties of water are undeniable (and observable in daily life by anybody). Any claims that it sticks to the exterior of shapes must provide verifiable, repeatable, testable, measurable evidence. It should also be evidence that any person can reproduce, otherwise you concede your right to judge for yourself to a higher authority (e.g. another person or institution that says it is smarter than you and is allowed to contradict your common sense). Don't allow this anymore, because otherwise I have some pink unicorns to sell you.
5) Look at the CGI produced by the institutions that we thought we could trust. (e.g. NASA). I'm sure with your background/expertise you can easily discern the difference between real photographic evidence and cartoons. Ask yourself why? Then go and have a laugh at all the shite they churn out (e.g. bubbles in space). The science of space is just sci-fi movies like Hollywood.
I realised over 3 years ago and my initial zetetic fever lasted a good 3 months. There is no physical pain but certainly some mental anguish, so just try and enjoy the ride. If you need new friends afterwards, you'll find some here :-) Arra best...
Liams response
Aha, hi Gav!
I was hoping you would be active in the comments. Thanks for getting in touch. I was wondering if you could give details on what it is you studied in Glasgow and to what level? (Undergrad/ Postgrad/ PHD etc).
I'm a firm believer that Mathematics is a universal language that with enough understanding, it should be able to describe / give definition to everything in the Universe.
I am very keen to learn more about the properties of water as I found myself stumped trying to explain why water acts the way it does on a spherical Earth, as is my belief ('belief' count continues). I think that this question will have an explanation but right now I do not have an answer, but this is something I will be reading up on.
I have some issue with the choice of images used in the video to highlight the use of "CGI" by NASA or other organisations as, yes they are obviously edited images, but they have been used here out of context. I'm referencing the images used from 31:58 onwards in the video. A lot of the images you come across on NASA's website are used to give visual eye candy to the audience, they were not used as a "proof". During the interview I was unaware that it was these kind of images that John was referring to. Here I would say, yes you cannot deny that these images are CGI, however the context is all wrong. I don't think NASA could upload any kind of image without people scrutinizing its validity and I feel like this is going to be the case with my comments on them as well. Thanks for your comment man, nice hearing from you. Liam
My reply
I am hesitant on the topic of credentials for the sole reason that I think them irrelevant and distracting to the more central questions. Background expertise does not necessarily equate to powers of discernment. An important distinction, I hope you agree?
I also forsee shill & troll nonsense for which I have no time nor any inclination to ignite. So before I tell you let me please make a few points ...
The important questions for me are: What is knowledge? How do we obtain knowledge of the external world? What are the first principles of common sense and reason?
I think we can agree that we are an integral part of nature, a hard-wired physical being of substance, having a mind-body experience that we call lived life. But what do we really know about our own constitution?
To use an analogy familiar to you, do we really know our own motherboard, CPU, RAM, graphics, storage, interfaces, sensors, and attached peripherals? What about the firmware on all our biological chips? What do we really know about our own BIOS, drivers, kernel, assembler, compiler, operating system, GUI, network, applications, real-time, in-memory, big data, and so on...?
So what can we agree on? Remember, there has to be a starting point from which we can mutually reason. You always need to bootstrap :-)
If there is no agreement between two parties about certain self-evident truths of reality, on the assumed axioms and common notions in geometry, in a measure of ratios of quantities of substance, a table of scales, in mathematics etc., then there is no point in proving a proposition to others, nor can one step be taken in mathematical reasoning. Why not? Because you cannot assume something in your proof, which the other party denies or which is no less self-evident than that which is to be proven.
Our common senses (sight, touch, sound, taste and smell) are an undeniable, natural part of our constitution and an experience shared by every sentient human being, and without which we could not survive. Whoever denies them in theories but employs them in lived life is a hypocrite.
Would you agree that the accumulated common senses of all mankind, over all time, is the correct foundation from which to reason and gain further knowledge? And that we also need to know how our mind-body-sense experience functions, so as to accurately infer knowledge of the external world?
Is fire hot? Is ice cold? Does the sun come up every day? What is a straight line? What is a perpendicular? What are parallel lines? What is level? What constitutes a unit of quantity? What is capable of being measured and how? These are fundamental questions discussed for thousands of years and still are to this very day. These are not foregone conclusions to be readily dismissed as scientism would have us think.
Only when we have agreement on such first principles can we ask questions, interpret answers and form conclusions about the external world in a way that everybody can follow and concur. It is in this respect that we are all the same. We all share the same experiences, we are all equipped with the same mental and physical apparatus and therefore all of us share EQUAL credentials.
For me the mutual content of all the sense experiences of everybody, is the ultimate authority and not that of any letters after my name or of anybody else for that matter. If you think the latter more necessary than the former, then any weight given to your overall judgement on these subjects, will depend solely on YOUR perception in YOUR mind about the importance of such credentials, rather than the original merits of the reasoning, logic or content.
I am first and foremost a man with common sense and only thereafter do I consider myself an academic. I would rather be in the pub talking common sense with your average honest joe (or with Del in his shed), than in seminar halls debating theories that contradict natural facts with deluded academics who don't understand the difference.
That said (and hopefully it's clear what I mean), here is a breakdown of my early academic life. It goes against my best instincts to tell it, but if it helps you or others to know it, then fine.
1. I grew up in the Mull of Kintyre, in a fishing town where a comprehensive school education had all the usual subjects, but also included practical navigation & astronomy, woodwork & metalwork; where sport was football, rugby & golf; and where bagpipes & drums were optional but music essential :-) School was a doddle for me. What can I say? Took to it like a fish in water. More or less straight A's w/o having to try. I skipped a year finishing with six highers (including maths & physics) at the age of 16 and a half.
I went on to Glasgow University and obtained a combined Honours degree in Mathematics & Natural Philosophy, under the Simson chair of Mathematics and the Kelvin chair of Natural Philosophy. Robert Simson was the great restorer of Euclidean Geometry and an influential figure in the Scottish Enlightenment. I doubt Lord Kelvin (William Thomson) needs much introduction.
http://www.universitystory.gla.ac.uk/chair-and-lectureship/?id=790
http://www.universitystory.gla.ac.uk/chair-and-lectureship/?id=870
http://www.universitystory.gla.ac.uk/chair-and-lectureship/?id=81
http://www.universitystory.gla.ac.uk/chair-and-lectureship/?id=64
Courses included all branches of Mathematics, Algebra, Analysis, Geometry, Logic, Topology, Mensuration, Calculus, etc, and in Natural Philosophy (nowadays Physics & Astrophysics) we covered a wide range from Metaphysics, Principles and Matter, Cosmologies, Quantum Mechanics, Optics, Electromagnetism, and various Applied Mathematics and Physics, etc. I took additional courses in computing science starting with micro-processors and punch cards and learning the basics of firmware and assembler. I also didn't forget to enjoy the student life ;-)
Entry qualifications were very high and only clever little bastards were allowed to study the combined maths and physics. There were only four of us who completed the 4 years of study. I wasn't even 21 years old when I graduated and I only had to shave once a week :-)
I won a scholarship to do a doctorate in Quantum mechanics and Group Theory at the Max Planck University in Germany, which I did not start (to the dismay of my mother, who wanted a doctor in the family :-), preferring instead to enjoy life as a (not very good) professional golfer, following in my dads more successful footsteps. At twenty-five I gave it up and started my own IT business and a life of self-employment.
2. I am also a firm believer in mathematics being a great language to describe and explain this reality, but only if the foundations are rooted in natural facts and agreed self-evident truths and first principles. It can otherwise be mis-used to present a false picture of reality w/o the actual equations or mathematical reasoning being wrong. Ever since Euclid rigorously defined the axioms of geometry used in the construction of everything we have ever built, there have been literally hundreds of different models of geometry created by tweaking and replacing the axioms of straight and parallel lines. These have given us hyperbolic, elliptic geometries all the way to the curved space-time bullshit of today. All these geometries are rigorous and faultless based only on the assumed axioms. So mathematics is not a proof of reality. Many think otherwise but I personally see that as tantamount to a belief in the religion of scientism. This is something I only realised later in life. They say youth is wasted on the young, but you only know what that means when you are older :-)
3. Regarding the images shown to you, I don't think there IS any other context. I agree that visualisations to convey an idea can be put to great effect and that if this is their sole purpose then fine. But NASA claims they are accurate composites interpreted from real data. If you can find real continuous unedited video footage of machines leaving earth and going all the way into space, then please share them. All anybody can find is cartoons or films created with green screen technology and CGI. As I am sure you are aware, that can look very realistic, at least if no mistakes are made. Unfortunately for them, they keep doing so. Again I can show you pictures of pink unicorns, but that cannot be considered proof of their real existence.
If you want to discuss anymore then I would rather have a real chat. I can be reached at vortexpuppy(at)gmail.com. Reach out and we can exchange Skype handles or whatever if you like. Otherwise take care and good luck in your quest for truth. Either way a mind expanded can never be shrunk to its original size...
I think it is a relevant summary to this thread and since this has been my home for a few years (and still is), I'll post it here, so you can all get to know me a little better. The same invitation extended to Liam, applies to everybody else here as well. Time permitting.
Look forward to bringing this deception to an end with you all. The quicker the fucking better.
My original comment to Liam
Hi Liam, good on you for doing the interview and coming here man. Great to see it. If only everybody was as open-minded as you and would start questioning. I'm Gav, the friend of John, whom he mentions in the interview (Maths/Physics from Glasgow Uni). If you have any questions don't hesitate to reach out. If I can give some advice for a newbie it would be this:
1) Continue to question everything and think for yourself. You will find many "beliefs" in your head, that are not your own (e.g. Space, Spinning balls, etc). Discard everything that has no basis in reality and evaluate from scratch. Don't believe anybody or anything you can't verify for yourself.
2) Don't get bogged down in "other theories or models" from many so-called flat earthers. Many are deliberate distractions to perpetuate division and strife. Alternative theories or models, should be equally dismissed if they have no proof other than mathematical ones. Mathematics is a formal science, like language. Definitions are neither true nor false, they are just definitions, so Maths is no proof of anything. This is also the reason why many clever people are duped and not that there is a conspiracy by all scientists around the world. They pull down their trousers to shite like the rest of us and are just as easily deceived (and heh, the maths works, so it must be right ;-) or indoctrinated into a belief system.
3) Concentrate on questioning obvious paradoxes, absurdities and contradictions to common sense and natural observations. You (and I) have every right to argue against a hypothesis (or theory) that contradicts the facts (e.g. level water), but to argue from a hypotheses against the facts is just bad natural philosophy / science. Your senses do not deceive you just because you don't fully understand how they work. (e.g. vision and perspective). If basic contradictions cannot be answered by modern day science, then something is horribly fucking wrong, and it isn't that all mankind is too dumb to understand.
4) The natural properties of water are undeniable (and observable in daily life by anybody). Any claims that it sticks to the exterior of shapes must provide verifiable, repeatable, testable, measurable evidence. It should also be evidence that any person can reproduce, otherwise you concede your right to judge for yourself to a higher authority (e.g. another person or institution that says it is smarter than you and is allowed to contradict your common sense). Don't allow this anymore, because otherwise I have some pink unicorns to sell you.
5) Look at the CGI produced by the institutions that we thought we could trust. (e.g. NASA). I'm sure with your background/expertise you can easily discern the difference between real photographic evidence and cartoons. Ask yourself why? Then go and have a laugh at all the shite they churn out (e.g. bubbles in space). The science of space is just sci-fi movies like Hollywood.
I realised over 3 years ago and my initial zetetic fever lasted a good 3 months. There is no physical pain but certainly some mental anguish, so just try and enjoy the ride. If you need new friends afterwards, you'll find some here :-) Arra best...
Liams response
Aha, hi Gav!
I was hoping you would be active in the comments. Thanks for getting in touch. I was wondering if you could give details on what it is you studied in Glasgow and to what level? (Undergrad/ Postgrad/ PHD etc).
I'm a firm believer that Mathematics is a universal language that with enough understanding, it should be able to describe / give definition to everything in the Universe.
I am very keen to learn more about the properties of water as I found myself stumped trying to explain why water acts the way it does on a spherical Earth, as is my belief ('belief' count continues). I think that this question will have an explanation but right now I do not have an answer, but this is something I will be reading up on.
I have some issue with the choice of images used in the video to highlight the use of "CGI" by NASA or other organisations as, yes they are obviously edited images, but they have been used here out of context. I'm referencing the images used from 31:58 onwards in the video. A lot of the images you come across on NASA's website are used to give visual eye candy to the audience, they were not used as a "proof". During the interview I was unaware that it was these kind of images that John was referring to. Here I would say, yes you cannot deny that these images are CGI, however the context is all wrong. I don't think NASA could upload any kind of image without people scrutinizing its validity and I feel like this is going to be the case with my comments on them as well. Thanks for your comment man, nice hearing from you. Liam
My reply
I am hesitant on the topic of credentials for the sole reason that I think them irrelevant and distracting to the more central questions. Background expertise does not necessarily equate to powers of discernment. An important distinction, I hope you agree?
I also forsee shill & troll nonsense for which I have no time nor any inclination to ignite. So before I tell you let me please make a few points ...
The important questions for me are: What is knowledge? How do we obtain knowledge of the external world? What are the first principles of common sense and reason?
I think we can agree that we are an integral part of nature, a hard-wired physical being of substance, having a mind-body experience that we call lived life. But what do we really know about our own constitution?
To use an analogy familiar to you, do we really know our own motherboard, CPU, RAM, graphics, storage, interfaces, sensors, and attached peripherals? What about the firmware on all our biological chips? What do we really know about our own BIOS, drivers, kernel, assembler, compiler, operating system, GUI, network, applications, real-time, in-memory, big data, and so on...?
So what can we agree on? Remember, there has to be a starting point from which we can mutually reason. You always need to bootstrap :-)
If there is no agreement between two parties about certain self-evident truths of reality, on the assumed axioms and common notions in geometry, in a measure of ratios of quantities of substance, a table of scales, in mathematics etc., then there is no point in proving a proposition to others, nor can one step be taken in mathematical reasoning. Why not? Because you cannot assume something in your proof, which the other party denies or which is no less self-evident than that which is to be proven.
Our common senses (sight, touch, sound, taste and smell) are an undeniable, natural part of our constitution and an experience shared by every sentient human being, and without which we could not survive. Whoever denies them in theories but employs them in lived life is a hypocrite.
Would you agree that the accumulated common senses of all mankind, over all time, is the correct foundation from which to reason and gain further knowledge? And that we also need to know how our mind-body-sense experience functions, so as to accurately infer knowledge of the external world?
Is fire hot? Is ice cold? Does the sun come up every day? What is a straight line? What is a perpendicular? What are parallel lines? What is level? What constitutes a unit of quantity? What is capable of being measured and how? These are fundamental questions discussed for thousands of years and still are to this very day. These are not foregone conclusions to be readily dismissed as scientism would have us think.
Only when we have agreement on such first principles can we ask questions, interpret answers and form conclusions about the external world in a way that everybody can follow and concur. It is in this respect that we are all the same. We all share the same experiences, we are all equipped with the same mental and physical apparatus and therefore all of us share EQUAL credentials.
For me the mutual content of all the sense experiences of everybody, is the ultimate authority and not that of any letters after my name or of anybody else for that matter. If you think the latter more necessary than the former, then any weight given to your overall judgement on these subjects, will depend solely on YOUR perception in YOUR mind about the importance of such credentials, rather than the original merits of the reasoning, logic or content.
I am first and foremost a man with common sense and only thereafter do I consider myself an academic. I would rather be in the pub talking common sense with your average honest joe (or with Del in his shed), than in seminar halls debating theories that contradict natural facts with deluded academics who don't understand the difference.
That said (and hopefully it's clear what I mean), here is a breakdown of my early academic life. It goes against my best instincts to tell it, but if it helps you or others to know it, then fine.
1. I grew up in the Mull of Kintyre, in a fishing town where a comprehensive school education had all the usual subjects, but also included practical navigation & astronomy, woodwork & metalwork; where sport was football, rugby & golf; and where bagpipes & drums were optional but music essential :-) School was a doddle for me. What can I say? Took to it like a fish in water. More or less straight A's w/o having to try. I skipped a year finishing with six highers (including maths & physics) at the age of 16 and a half.
I went on to Glasgow University and obtained a combined Honours degree in Mathematics & Natural Philosophy, under the Simson chair of Mathematics and the Kelvin chair of Natural Philosophy. Robert Simson was the great restorer of Euclidean Geometry and an influential figure in the Scottish Enlightenment. I doubt Lord Kelvin (William Thomson) needs much introduction.
http://www.universitystory.gla.ac.uk/chair-and-lectureship/?id=790
http://www.universitystory.gla.ac.uk/chair-and-lectureship/?id=870
http://www.universitystory.gla.ac.uk/chair-and-lectureship/?id=81
http://www.universitystory.gla.ac.uk/chair-and-lectureship/?id=64
Courses included all branches of Mathematics, Algebra, Analysis, Geometry, Logic, Topology, Mensuration, Calculus, etc, and in Natural Philosophy (nowadays Physics & Astrophysics) we covered a wide range from Metaphysics, Principles and Matter, Cosmologies, Quantum Mechanics, Optics, Electromagnetism, and various Applied Mathematics and Physics, etc. I took additional courses in computing science starting with micro-processors and punch cards and learning the basics of firmware and assembler. I also didn't forget to enjoy the student life ;-)
Entry qualifications were very high and only clever little bastards were allowed to study the combined maths and physics. There were only four of us who completed the 4 years of study. I wasn't even 21 years old when I graduated and I only had to shave once a week :-)
I won a scholarship to do a doctorate in Quantum mechanics and Group Theory at the Max Planck University in Germany, which I did not start (to the dismay of my mother, who wanted a doctor in the family :-), preferring instead to enjoy life as a (not very good) professional golfer, following in my dads more successful footsteps. At twenty-five I gave it up and started my own IT business and a life of self-employment.
2. I am also a firm believer in mathematics being a great language to describe and explain this reality, but only if the foundations are rooted in natural facts and agreed self-evident truths and first principles. It can otherwise be mis-used to present a false picture of reality w/o the actual equations or mathematical reasoning being wrong. Ever since Euclid rigorously defined the axioms of geometry used in the construction of everything we have ever built, there have been literally hundreds of different models of geometry created by tweaking and replacing the axioms of straight and parallel lines. These have given us hyperbolic, elliptic geometries all the way to the curved space-time bullshit of today. All these geometries are rigorous and faultless based only on the assumed axioms. So mathematics is not a proof of reality. Many think otherwise but I personally see that as tantamount to a belief in the religion of scientism. This is something I only realised later in life. They say youth is wasted on the young, but you only know what that means when you are older :-)
3. Regarding the images shown to you, I don't think there IS any other context. I agree that visualisations to convey an idea can be put to great effect and that if this is their sole purpose then fine. But NASA claims they are accurate composites interpreted from real data. If you can find real continuous unedited video footage of machines leaving earth and going all the way into space, then please share them. All anybody can find is cartoons or films created with green screen technology and CGI. As I am sure you are aware, that can look very realistic, at least if no mistakes are made. Unfortunately for them, they keep doing so. Again I can show you pictures of pink unicorns, but that cannot be considered proof of their real existence.
If you want to discuss anymore then I would rather have a real chat. I can be reached at vortexpuppy(at)gmail.com. Reach out and we can exchange Skype handles or whatever if you like. Otherwise take care and good luck in your quest for truth. Either way a mind expanded can never be shrunk to its original size...
vortexpuppy- Posts : 167
Points : 3602
Reputation : 296
Join date : 2015-12-30
vortexpuppy- Posts : 167
Points : 3602
Reputation : 296
Join date : 2015-12-30
 Beauty is in the Eye of the Beholder. lol.
Beauty is in the Eye of the Beholder. lol.
"Beauty is in the Eye of the Beholder", or "The Good, the Bad and the Ugly of Philosophy", or "Mathematics is proof of fucking Nothing" or "Riemannian space ball worship loses its way".
by Vortexpuppy.
Naive or Direct Realism and Common Sense have been deliberately overrun by ugly, stupid philosophies of Idealism / Representative Realism / Skepticism. Their definitions and understandings of WHAT Knowledge is and HOW we obtain knowledge, are ALL fundamentally flawed with the same error since their inception.
They can DENY experiential Nature (that includes you and me) and put it "in or out of the Equation", AT WILL.
This has been "overlooked" for a thousand years and is regarded by modern academics (e.g. Dan Robinson, John Searle) to be the biggest ever disaster in Philosophical history. An example of its influence and deep entrenchment in the field of Maths & Geometry is shown below, where position, direction, orientation and motion are separated from Mathematics and moved to the Physical Sciences. Too difficult. Better that nobody knows everything. WTF?
Mathematics is a modern creation about 150-200 years before Riemann. Mathematics is the Doctrine of Ratio, Quantity & Mensuration.
the abstract science of number, quantity, and space, either as abstract concepts ( pure mathematics ), or as applied to other disciplines such as physics and engineering ( applied mathematics ).
mathematic (n.)
late 14c. as singular noun, replaced by early 17c. by mathematics, from Latin mathematica (plural), from Greek mathematike tekhne "mathematical science," feminine singular of mathematikos (adj.) "relating to mathematics, scientific, astronomical; disposed to learn," from mathema (genitive mathematos) "science, knowledge, mathematical knowledge; a lesson," literally "that which is learnt;" related to manthanein "to learn," from PIE root *mendh- "to learn" (source also of Greek menthere "to care," Lithuanian mandras "wide-awake," Old Church Slavonic madru "wise, sage," Gothic mundonsis "to look at," German munter "awake, lively"). As an adjective, 1540s, from French mathématique or directly from Latin mathematicus.
When we ignore nature lol, then we are free to theorize, and admit of mathematical reasoning not just proper real quantities of natural stuff (whether visibles or tangibles), but also new definitions of quantities & measurement that have no need of substance or material impression. lol.
They are IDEAS. Fictions of the mind, like similes or analogies that are ONLY just in their use, when intended to CONVEY the REAL relations between PROPER natural things.
Mathematics can easily deliver to real physical measurements and engineer-able calculations when it deals with real proper things. Mathematics is then SEEN to "prove it". However it can also have us chasing sense data that doesn't exist, seeking impossible experiences of fictional (super/supra) unnatural things.
What the ancients called an improper quantity (an IDEA), must be defined in terms of some other REAL quantity or quantities, to be an object of mathematical reasoning (i.e. measurable) for example Speed = Distance / Time. All good so far, since the IDEA is conveying real natural quantities such as 100 feet in 20 seconds.
The fictive sense data is born when an IDEA, is allowed to exist:
a) WITHOUT any measurable definition in terms of real proper quantities (e.g. Escape Velocity = Fairy Dust / Gravitons)
b) OR where TWO or more definitions EXIST for measuring ONE and the SAME thing (see the Equivalence Principle)
c) AND where in all cases language is abused. Clever rhetoric and sophism is employed to contribute a final polish that confounds the difference, bewitches the beholder and bewilders the senses.
For example the meaning of Speed:
Q:Which of the following is correct?
a) the extension of space passed over in a given duration,
b) the length covered in a given interval,
c) the distance traveled in a given time
A: Some, All or None depending on your point of view. lol
Empiricism and the Scientific method use the formal language of Mathematics, a modern Doctrine of Ratio, Scale, Quantity and Measure, in where it is defined what these words actually mean, which Objects are admissible to measurement (and hence mathematical reasoning), which tangible and visible objects exist, and which of these objects are occult-ed, hidden or ignored for which motives.
Of course this can be willfully abused. It REALLY would be NAIVE to think otherwise. Special interest groups can choose the ratio or scale that suits the purpose of the intended measurement and define the rulers, circles of measure and spheres of geometry together with descriptions of the tools, instruments and methods of how best to "master" the process.
Don't believe any measurement you haven't defined yourself. Nor any statistic you didn't falsify yourself. Grr... Grr...
Riemannian Geometry is the current geometry bible. The basis for explaining astronomy and navigation and of modern Ein-schtick-stein relativity. The relative observers just had to be brought back in somehow after Riemann removed them. It just wouldn't quite work PROPERLY otherwise ;-)
And the final insult to our intelligence, the last fucking straw. Ladies and gentleman, we present to you.
The Magic of Curved Water in bendy Space/Time.
For fucks sake, if that doesn't make you come up for a lungful of air and gasp in disbelief, then you've already drowned.
Nuff said, have a butchers at the excerpt of an English translation of the Habilitation of Bernhard Riemann. The famous protege of Gauss, himself son of a mason and Mr Curvature himself.
You don't have to be a mathematician. You only need to be awake to common sense. You too can experience firsthand a sophisticated, metaphysical geometrical wonderland. The modern reality brought to you by abusing common words to sell you fucking fairy stories.
I look forward real soon to watching academic beliefs dissolving in real-time when it realises that EVERYBODY else already knows the error/mistake/lie/deception, the man on the street, my kids and my granny. That the answer is in all of us, that's why we feel it. And that neither can they deny it , not even in their little bubble, lest they deny reality, their role in nature and good old common sense.
This whole nonsense ought to be scoffed at, and it is at long last being ridiculed by all mankind. And quite fucking rightly so. Academics can now only choose to be the last to know. Be carried downstream with the herd of common believers. What a horrible irony.
Please my friends, tell me. It's not difficult to see is it? You don't need the equations to see the problem do you ? Help me wake them up.
On the Hypotheses which lie at the Bases of Geometry.
Bernhard Riemann
Translated by William Kingdon Clifford
Synopsis.
Plan of the Inquiry:
I. Notion of an n-ply extended magnitude.
§ 1. Continuous and discrete manifoldnesses. Defined parts of a man-ifoldness are called Quanta. Division of the theory of continuous magnitude into the theories,
(1) Of mere region-relations, in which an independence of magnitudes from position is not assumed;
(2) Of size-relations, in which such an independence must be assumed.
§ 2. Construction of the notion of a one-fold, two-fold, n-fold extended magnitude.
§ 3. Reduction of place-fixing in a given manifoldness to quantity fixings. True character of an n-fold extended magnitude.
II. Measure-relations of which a manifoldness of n-dimensions is capable on the assumption that lines have a length independent of position, and consequently that every line may be measured by every other.
§ 1. Expression for the line-element. Manifoldnesses to be called Flat in which the line-element is expressible as the square root of a sum of squares of complete differentials.
§ 2. Investigation of the manifoldness of n-dimensions in which the lineelement may be represented as the square root of a quadric differential. Measure of its deviation from flatness (curvature) at a given point in a given surface-direction. For the determination of its measure-relations it is allowable and sufficient that the curvature be arbitrarily given at every point in 1 n(n 1) surface directions.
§ 3. Geometric illustration.
§ 4. Flat manifoldnesses (in which the curvature is everywhere = 0) may be treated as a special case of manifoldnesses with constant curvature. These can also be defined as admitting an independence of n-fold extents in them from position (possibility of motion without stretching).
§ 5. Surfaces with constant curvature.
III. Application to Space.
§ 1. System of facts which suffice to determine the measure-relations of space assumed in geometry.
§ 2. How far is the validity of these empirical determinations probable beyond the limits of observation towards the infinitely great?
§ 3. How far towards the infinitely small? Connection of this question with the interpretation of nature.
by Vortexpuppy.
Naive or Direct Realism and Common Sense have been deliberately overrun by ugly, stupid philosophies of Idealism / Representative Realism / Skepticism. Their definitions and understandings of WHAT Knowledge is and HOW we obtain knowledge, are ALL fundamentally flawed with the same error since their inception.
They can DENY experiential Nature (that includes you and me) and put it "in or out of the Equation", AT WILL.
This has been "overlooked" for a thousand years and is regarded by modern academics (e.g. Dan Robinson, John Searle) to be the biggest ever disaster in Philosophical history. An example of its influence and deep entrenchment in the field of Maths & Geometry is shown below, where position, direction, orientation and motion are separated from Mathematics and moved to the Physical Sciences. Too difficult. Better that nobody knows everything. WTF?
Mathematics is a modern creation about 150-200 years before Riemann. Mathematics is the Doctrine of Ratio, Quantity & Mensuration.
the abstract science of number, quantity, and space, either as abstract concepts ( pure mathematics ), or as applied to other disciplines such as physics and engineering ( applied mathematics ).
mathematic (n.)
late 14c. as singular noun, replaced by early 17c. by mathematics, from Latin mathematica (plural), from Greek mathematike tekhne "mathematical science," feminine singular of mathematikos (adj.) "relating to mathematics, scientific, astronomical; disposed to learn," from mathema (genitive mathematos) "science, knowledge, mathematical knowledge; a lesson," literally "that which is learnt;" related to manthanein "to learn," from PIE root *mendh- "to learn" (source also of Greek menthere "to care," Lithuanian mandras "wide-awake," Old Church Slavonic madru "wise, sage," Gothic mundonsis "to look at," German munter "awake, lively"). As an adjective, 1540s, from French mathématique or directly from Latin mathematicus.
When we ignore nature lol, then we are free to theorize, and admit of mathematical reasoning not just proper real quantities of natural stuff (whether visibles or tangibles), but also new definitions of quantities & measurement that have no need of substance or material impression. lol.
They are IDEAS. Fictions of the mind, like similes or analogies that are ONLY just in their use, when intended to CONVEY the REAL relations between PROPER natural things.
Mathematics can easily deliver to real physical measurements and engineer-able calculations when it deals with real proper things. Mathematics is then SEEN to "prove it". However it can also have us chasing sense data that doesn't exist, seeking impossible experiences of fictional (super/supra) unnatural things.
What the ancients called an improper quantity (an IDEA), must be defined in terms of some other REAL quantity or quantities, to be an object of mathematical reasoning (i.e. measurable) for example Speed = Distance / Time. All good so far, since the IDEA is conveying real natural quantities such as 100 feet in 20 seconds.
The fictive sense data is born when an IDEA, is allowed to exist:
a) WITHOUT any measurable definition in terms of real proper quantities (e.g. Escape Velocity = Fairy Dust / Gravitons)
b) OR where TWO or more definitions EXIST for measuring ONE and the SAME thing (see the Equivalence Principle)
c) AND where in all cases language is abused. Clever rhetoric and sophism is employed to contribute a final polish that confounds the difference, bewitches the beholder and bewilders the senses.
For example the meaning of Speed:
Q:Which of the following is correct?
a) the extension of space passed over in a given duration,
b) the length covered in a given interval,
c) the distance traveled in a given time
A: Some, All or None depending on your point of view. lol
Empiricism and the Scientific method use the formal language of Mathematics, a modern Doctrine of Ratio, Scale, Quantity and Measure, in where it is defined what these words actually mean, which Objects are admissible to measurement (and hence mathematical reasoning), which tangible and visible objects exist, and which of these objects are occult-ed, hidden or ignored for which motives.
Of course this can be willfully abused. It REALLY would be NAIVE to think otherwise. Special interest groups can choose the ratio or scale that suits the purpose of the intended measurement and define the rulers, circles of measure and spheres of geometry together with descriptions of the tools, instruments and methods of how best to "master" the process.
Don't believe any measurement you haven't defined yourself. Nor any statistic you didn't falsify yourself. Grr... Grr...
Riemannian Geometry is the current geometry bible. The basis for explaining astronomy and navigation and of modern Ein-schtick-stein relativity. The relative observers just had to be brought back in somehow after Riemann removed them. It just wouldn't quite work PROPERLY otherwise ;-)
And the final insult to our intelligence, the last fucking straw. Ladies and gentleman, we present to you.
The Magic of Curved Water in bendy Space/Time.
For fucks sake, if that doesn't make you come up for a lungful of air and gasp in disbelief, then you've already drowned.
Nuff said, have a butchers at the excerpt of an English translation of the Habilitation of Bernhard Riemann. The famous protege of Gauss, himself son of a mason and Mr Curvature himself.
You don't have to be a mathematician. You only need to be awake to common sense. You too can experience firsthand a sophisticated, metaphysical geometrical wonderland. The modern reality brought to you by abusing common words to sell you fucking fairy stories.
I look forward real soon to watching academic beliefs dissolving in real-time when it realises that EVERYBODY else already knows the error/mistake/lie/deception, the man on the street, my kids and my granny. That the answer is in all of us, that's why we feel it. And that neither can they deny it , not even in their little bubble, lest they deny reality, their role in nature and good old common sense.
This whole nonsense ought to be scoffed at, and it is at long last being ridiculed by all mankind. And quite fucking rightly so. Academics can now only choose to be the last to know. Be carried downstream with the herd of common believers. What a horrible irony.
Please my friends, tell me. It's not difficult to see is it? You don't need the equations to see the problem do you ? Help me wake them up.
On the Hypotheses which lie at the Bases of Geometry.
Bernhard Riemann
Translated by William Kingdon Clifford
Synopsis.
Plan of the Inquiry:
I. Notion of an n-ply extended magnitude.
§ 1. Continuous and discrete manifoldnesses. Defined parts of a man-ifoldness are called Quanta. Division of the theory of continuous magnitude into the theories,
(1) Of mere region-relations, in which an independence of magnitudes from position is not assumed;
(2) Of size-relations, in which such an independence must be assumed.
§ 2. Construction of the notion of a one-fold, two-fold, n-fold extended magnitude.
§ 3. Reduction of place-fixing in a given manifoldness to quantity fixings. True character of an n-fold extended magnitude.
II. Measure-relations of which a manifoldness of n-dimensions is capable on the assumption that lines have a length independent of position, and consequently that every line may be measured by every other.
§ 1. Expression for the line-element. Manifoldnesses to be called Flat in which the line-element is expressible as the square root of a sum of squares of complete differentials.
§ 2. Investigation of the manifoldness of n-dimensions in which the lineelement may be represented as the square root of a quadric differential. Measure of its deviation from flatness (curvature) at a given point in a given surface-direction. For the determination of its measure-relations it is allowable and sufficient that the curvature be arbitrarily given at every point in 1 n(n 1) surface directions.
§ 3. Geometric illustration.
§ 4. Flat manifoldnesses (in which the curvature is everywhere = 0) may be treated as a special case of manifoldnesses with constant curvature. These can also be defined as admitting an independence of n-fold extents in them from position (possibility of motion without stretching).
§ 5. Surfaces with constant curvature.
III. Application to Space.
§ 1. System of facts which suffice to determine the measure-relations of space assumed in geometry.
§ 2. How far is the validity of these empirical determinations probable beyond the limits of observation towards the infinitely great?
§ 3. How far towards the infinitely small? Connection of this question with the interpretation of nature.
vortexpuppy- Posts : 167
Points : 3602
Reputation : 296
Join date : 2015-12-30
 Time to get real ...
Time to get real ...
Time to get real ... It's all over bar the shouting.
vortexpuppy- Posts : 167
Points : 3602
Reputation : 296
Join date : 2015-12-30

lizardking- Posts : 1673
Points : 7480
Reputation : 2604
Join date : 2015-12-30
Age : 30
Location : United Kingdom
 Re: Direct Vision, Rationality, Realism and Common Sense.
Re: Direct Vision, Rationality, Realism and Common Sense.
Excellent playlist!
Most relevent part to gravity myth is video#11.
Most likely by now the author is aware of flat earth.
Most relevent part to gravity myth is video#11.
Most likely by now the author is aware of flat earth.
RelearningLogic- Posts : 18
Points : 2926
Reputation : 13
Join date : 2016-08-21
 Euclids Geometry and its uses
Euclids Geometry and its uses
Euclid and his Geometry are revered in certain societies and held in high esteem.
Why is that? Is there another reason apart from the obvious one?
Mastering Euclid's geometry enabled certain groups to build expensive cathedrals and other complex constructions, most likely making them rich and powerful in the process. So that's probably one reason at least to a certain degree, as to why Euclid is revered in these circles.
To a higher degree, I could also conceive of Euclid being revered because his Geometry is cleverly used to "occult" the following self-evident truths about our mind-body reality.
The self-evident truths are:
• Visible & Tangible Space both exist in this reality. They are always there. Independent of us. A view exists irrespective of whether an Eye is situated in such a position so as to see it.
• Visible & Tangible Space are conjoined, in that, without a Tangible real object in our real Tangible Space there can be no Visible object (Object of Sight) in our Visible Space. If there is no object then nothing can be seen.
• Visible & Tangible Space have different qualities and properties distinct from each other. For example Visible railway lines appear to converge, whereas in tangible space they never do.
• A Visible is an Object of Sight. It is conveyed in a natural (unspoken) language and is the "sign" seen, by the eye, of a real external object, when light makes a material impression on our retina.
• A Tangible is an Object of Touch. It is the thing signified (by the “sign”) conveyed in the language of touch, taste, smell and sound, an overall sentient experience of a real external object, that generally includes all our common senses, again via material impressions.
• Visible Geometry & Space is correctly described by spherical geometry. This has not been researched or discussed in mathematics or physics in all of known history (at least the last two thousand years).
• Tangible Geometry & Space is planar. It is everywhere experiential and in plain sight, overwhelming the senses (and the mind) daily. Even when we see a different visible figure, we think of the same tangible object
If we also know that
• Visibles (objects of sight) are positioned as visible figure and extension on the surface of a sphere when the eye is conceived to be at the centre.
• Visibles when seen directly, and as if at the centre of a sphere, will appear perfectly rectilinear (planar) unless very close or high in the sky.
• Visible & Tangible Geometry (spherical and planar) are both 2D Geometries sharing common roots within a framework where the principle of duality applies. They can be interchanged by tweaking certain assumptions and definitions.
The clever use of Euclidean geometry can render us inattentive to the above. It can all be hidden in plain sight.
The incessant use of (perspective) planes to describe reality is not only used in mathematical geometry and the physical sciences (e.g. level is the tangent plane to a radial from the centre of a space ball) but also in the constant bombardment of images and moving pictures on planar devices (e.g. Hollywood on flat screen TVs).
We are naturally constituted to go from the signs seen (by the eye), to the things signified by them (in our minds). This hard-wired faculty is a part of our common-sense package. Our survival kit. Our instincts if you like. We need it to learn stuff, to study nature, to observe our environment. We need it to function in daily lived life. We’ve been doing it since we were born. We don't attend to it anymore. We don’t recognize that we do it all the time. We don't notice that visible figure and extension change continuously but that the thing signified (by those signs) in our minds seldom does.
There are those who know this and use it to their advantage. They know how it works and how it can be used to manipulate. For them, visual perception and its operations of the mind are well understood. By presenting signs to our eyes, they can make us believe things. By manipulating words and definitions they can make it all seem to work.
Maybe they thought to themselves:
• Let’s draw geometric figures with mathematical definitions and make believe its real,
• Let’s render images on screens computing in binary arithmetic,
• Let’s paint by numbers and enforce a belief by incessant repetition,
• Let’s make sure they ignore reality and look only at ideas in pictures,
• Then they will never see what’s really in front of their eyes all the time.
The self-evident truths and facts mentioned above also go entirely unnoticed by Science. Its been that way for thousands of years.
You will not find any proposition anywhere in mathematics or physics that deals with the Geometry of Visible Space.
Yet that very (spherical) Geometry is the same as that of Riemann geometry which is used in all of modern science.
So an obvious question to ask is:
Why is the Geometry of Visible Space (our natural perspective) not properly researched or discussed in mathematical scientific circles?
For me it remains a fucking elephant in the room that has to be urgently addressed.
Why is that? Is there another reason apart from the obvious one?
Mastering Euclid's geometry enabled certain groups to build expensive cathedrals and other complex constructions, most likely making them rich and powerful in the process. So that's probably one reason at least to a certain degree, as to why Euclid is revered in these circles.
To a higher degree, I could also conceive of Euclid being revered because his Geometry is cleverly used to "occult" the following self-evident truths about our mind-body reality.
The self-evident truths are:
• Visible & Tangible Space both exist in this reality. They are always there. Independent of us. A view exists irrespective of whether an Eye is situated in such a position so as to see it.
• Visible & Tangible Space are conjoined, in that, without a Tangible real object in our real Tangible Space there can be no Visible object (Object of Sight) in our Visible Space. If there is no object then nothing can be seen.
• Visible & Tangible Space have different qualities and properties distinct from each other. For example Visible railway lines appear to converge, whereas in tangible space they never do.
• A Visible is an Object of Sight. It is conveyed in a natural (unspoken) language and is the "sign" seen, by the eye, of a real external object, when light makes a material impression on our retina.
• A Tangible is an Object of Touch. It is the thing signified (by the “sign”) conveyed in the language of touch, taste, smell and sound, an overall sentient experience of a real external object, that generally includes all our common senses, again via material impressions.
• Visible Geometry & Space is correctly described by spherical geometry. This has not been researched or discussed in mathematics or physics in all of known history (at least the last two thousand years).
• Tangible Geometry & Space is planar. It is everywhere experiential and in plain sight, overwhelming the senses (and the mind) daily. Even when we see a different visible figure, we think of the same tangible object
If we also know that
• Visibles (objects of sight) are positioned as visible figure and extension on the surface of a sphere when the eye is conceived to be at the centre.
• Visibles when seen directly, and as if at the centre of a sphere, will appear perfectly rectilinear (planar) unless very close or high in the sky.
• Visible & Tangible Geometry (spherical and planar) are both 2D Geometries sharing common roots within a framework where the principle of duality applies. They can be interchanged by tweaking certain assumptions and definitions.
The clever use of Euclidean geometry can render us inattentive to the above. It can all be hidden in plain sight.
The incessant use of (perspective) planes to describe reality is not only used in mathematical geometry and the physical sciences (e.g. level is the tangent plane to a radial from the centre of a space ball) but also in the constant bombardment of images and moving pictures on planar devices (e.g. Hollywood on flat screen TVs).
We are naturally constituted to go from the signs seen (by the eye), to the things signified by them (in our minds). This hard-wired faculty is a part of our common-sense package. Our survival kit. Our instincts if you like. We need it to learn stuff, to study nature, to observe our environment. We need it to function in daily lived life. We’ve been doing it since we were born. We don't attend to it anymore. We don’t recognize that we do it all the time. We don't notice that visible figure and extension change continuously but that the thing signified (by those signs) in our minds seldom does.
There are those who know this and use it to their advantage. They know how it works and how it can be used to manipulate. For them, visual perception and its operations of the mind are well understood. By presenting signs to our eyes, they can make us believe things. By manipulating words and definitions they can make it all seem to work.
Maybe they thought to themselves:
• Let’s draw geometric figures with mathematical definitions and make believe its real,
• Let’s render images on screens computing in binary arithmetic,
• Let’s paint by numbers and enforce a belief by incessant repetition,
• Let’s make sure they ignore reality and look only at ideas in pictures,
• Then they will never see what’s really in front of their eyes all the time.
The self-evident truths and facts mentioned above also go entirely unnoticed by Science. Its been that way for thousands of years.
You will not find any proposition anywhere in mathematics or physics that deals with the Geometry of Visible Space.
Yet that very (spherical) Geometry is the same as that of Riemann geometry which is used in all of modern science.
So an obvious question to ask is:
Why is the Geometry of Visible Space (our natural perspective) not properly researched or discussed in mathematical scientific circles?
For me it remains a fucking elephant in the room that has to be urgently addressed.
vortexpuppy- Posts : 167
Points : 3602
Reputation : 296
Join date : 2015-12-30
 Re: Direct Vision, Rationality, Realism and Common Sense.
Re: Direct Vision, Rationality, Realism and Common Sense.
Light does not bend it can only change angles.
Prisms
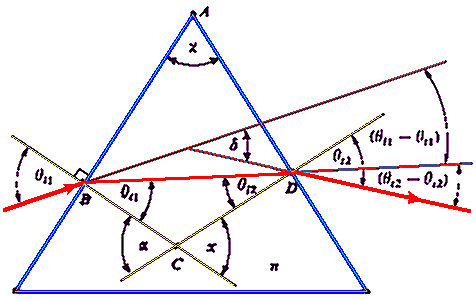
Optics (Convex Lens)

Fiber Optics use "mirrored core" to reflect the light signal down the length.
Patent US20100195678
Laser - Internal reflection through liquid medium
Patent US3810041
"The laser beam passes longitudinally through the liquid laser medium sheet
in an off-axial direction and undergoes multiple total internal reflections in its
passage therethrough."

Prisms

Optics (Convex Lens)

Fiber Optics use "mirrored core" to reflect the light signal down the length.
Patent US20100195678

Laser - Internal reflection through liquid medium
Patent US3810041
"The laser beam passes longitudinally through the liquid laser medium sheet
in an off-axial direction and undergoes multiple total internal reflections in its
passage therethrough."


Schpankme- Posts : 1202
Points : 5961
Reputation : 1606
Join date : 2015-12-30
 Re: Direct Vision, Rationality, Realism and Common Sense.
Re: Direct Vision, Rationality, Realism and Common Sense.
Absolutely Schpankme. Thanks for contributing. My take is:
Light refracts when crossing the boundaries of different mediums and can be made to internally reflect, so as to be constrained with the same medium, but light always travels in straight lines before, during and after any directional change.
A stick in water will appear bent and to have a different visible position in your field of view, but the stick is not bent, nor are your optical senses playing tricks or untrustworthy. They are in fact accurately alerting you to the fact that another medium other than air is involved in that particular instance of your visual sensory perception. Makes perfect sense to me.
The place/position/bearing of visible figure & extension, their intervals and divisions, that are our objects of sight, are only accurately described with spherical geometry with the eye supposed at the centre of its own visual sphere. This in no way implies that light is curved. Just like bendy water, this is impossible and contradicts all know facts of lived life. Or does somebody want to show me how to cast a curved shadow with a straight stick?
However spherical geometry does correctly captures the totality of visual experience. For a person in a given location and the ability to do a half/full turn, look up and down, change the rake of his head and the angle of the eye, thereby changing the situation of the eye, then all the visibles that are seen, will find their shape and position accurately on a visual sphere, in a way similar to concepts in celestial astronomy.
If you should ask why this visual sphere is never seen and shapes do not appear curved, I say it is because with our direct vision we look directly and straight at an object and so for an eye at the centre, the outward curve is not discernible and since every part of an edged figure on a sphere is made up of arc segments of some great circle of that sphere, these arc segments and the figure of which they are the outline, appear more or less exactly as Euclidean straight lines. This is similar to a plane piece of paper appearing as a straight line when held straight at eye level.
We do not see in pictures. Nor do we see in sequences of images packaged in film rolls. Pictorial perspective captures only one instance of a visual scene for an eye in a particular situation, but spherical geometry describes the true appearances and positions of ALL visibles for an eye in a particular location.

Apropos Newtons prisms. They apparently "split white light" in to component rays by refracting different frequencies more or less. However when two prisms are laid back to back, white light will pass straight through. I ask myself how does that work then?
Light refracts when crossing the boundaries of different mediums and can be made to internally reflect, so as to be constrained with the same medium, but light always travels in straight lines before, during and after any directional change.
A stick in water will appear bent and to have a different visible position in your field of view, but the stick is not bent, nor are your optical senses playing tricks or untrustworthy. They are in fact accurately alerting you to the fact that another medium other than air is involved in that particular instance of your visual sensory perception. Makes perfect sense to me.
The place/position/bearing of visible figure & extension, their intervals and divisions, that are our objects of sight, are only accurately described with spherical geometry with the eye supposed at the centre of its own visual sphere. This in no way implies that light is curved. Just like bendy water, this is impossible and contradicts all know facts of lived life. Or does somebody want to show me how to cast a curved shadow with a straight stick?
However spherical geometry does correctly captures the totality of visual experience. For a person in a given location and the ability to do a half/full turn, look up and down, change the rake of his head and the angle of the eye, thereby changing the situation of the eye, then all the visibles that are seen, will find their shape and position accurately on a visual sphere, in a way similar to concepts in celestial astronomy.
If you should ask why this visual sphere is never seen and shapes do not appear curved, I say it is because with our direct vision we look directly and straight at an object and so for an eye at the centre, the outward curve is not discernible and since every part of an edged figure on a sphere is made up of arc segments of some great circle of that sphere, these arc segments and the figure of which they are the outline, appear more or less exactly as Euclidean straight lines. This is similar to a plane piece of paper appearing as a straight line when held straight at eye level.
We do not see in pictures. Nor do we see in sequences of images packaged in film rolls. Pictorial perspective captures only one instance of a visual scene for an eye in a particular situation, but spherical geometry describes the true appearances and positions of ALL visibles for an eye in a particular location.

Apropos Newtons prisms. They apparently "split white light" in to component rays by refracting different frequencies more or less. However when two prisms are laid back to back, white light will pass straight through. I ask myself how does that work then?
vortexpuppy- Posts : 167
Points : 3602
Reputation : 296
Join date : 2015-12-30
 The videos I did on the Las Vegas Hoax.They are portraying multiple sun positions ,shown by the casted shadows.This is commonsense direct observation and a psyop on the cosmos.
The videos I did on the Las Vegas Hoax.They are portraying multiple sun positions ,shown by the casted shadows.This is commonsense direct observation and a psyop on the cosmos.
The videos I did on the Las Vegas Hoax.They are portraying multiple sun positions ,shown by the casted shadows.This is commonsense, direct observation and a psyop on the cosmos.
Ziggy Kelleher- Posts : 16
Points : 2675
Reputation : 8
Join date : 2017-04-23
 The Enthymemme
The Enthymemme
This video deserves it's own thread,
https://www.youtube.com/watch?v=6TkaySc-Hsc
*wrong section, can a mod please move to an appropriate thread!
https://www.youtube.com/watch?v=6TkaySc-Hsc
*wrong section, can a mod please move to an appropriate thread!

FL@T-E@RTH- Posts : 216
Points : 3351
Reputation : 268
Join date : 2016-10-12
Age : 51
Location : Certainly Not On A Globe
 Re: Direct Vision, Rationality, Realism and Common Sense.
Re: Direct Vision, Rationality, Realism and Common Sense.
Thought I'd just post my recent comment to a video https://www.youtube.com/watch?v=tcMU_kNZTj4
because it summarizes some of the information in this thread...
Lets keep at them online but don't forget to hit the streets and talk to real people.
cheers, Gav.
Dear oh dear, Mr duped ones and/or sophist deceivers ...Let me list some of your fallacies:
1. not correctly differentiating between visible and tangible objects and their measures. Just because pool balls are round it doesn't make the billiard table a sphere.
2. believing you are spinning and upside down on a ball that contradicts our everyday common sense experience of a level motionless plane with orbiting luminaries. (The attempt above to use observed behavior as a positive claim of curvature is downright ludicrous)
3. trying to tie natural science observations to religious bullshit to deliberately discredit scientific objections, and to associate natural researchers with religious cults
4. believing that large bodies of water can conform to the exterior of shapes or that water can "schtickstein" to the outside of a ball. lol. Even Newtons analogous demonstrations with spinning buckets of water needed a container...bucket ..Duh.
5. shifting the burden of proof from those that claim we live on a spinning testicle in a vacuum to cries of "prove its flat", when nobody is making a claim, simply rejecting bullshit. Typical sophist crap. Ignoratio elenchi. Ignorance of the nature of refutation (of an argument).
6. Rely on common sense in every day life to stay alive, yet ignore/reject it in favour of a theory. LMFAO. Just hypocritical.
7. Relying on a formal science such as Mathematics (a man-made language of formal definitions) rather than Natural science to believe theories that contradict lived life.
Lets be clear about this:
It is CORRECT science to argue FROM the FACTS (motionless level water) AGAINST any absurd hypothesis that contradicts those facts (spinning water-covered testicles).
It is FALSE Pseudo-Science to argue FROM a Hypothesis (water can curve and we are spinning) AGAINST the known facts of lived life.
So show us your practical demonstration of the globe claim, with something other than cartoon imagery and plastic toys. If you can't it is pure shite that you are talking.
Dude, you better get a grip. Either you have not yet deconstructed your conditioned belief systems (try the socratic method on yourself and re-learn to think critically) or you are just a sophist troll deliberately perpetuating deceit for personal gain, in which case your days are numbered as people wake up and smell the bullshit.
Study the dynamics and behaviour of liquids/fluids, learn the Trivium (grammar, logic, rhetoric), trust your common senses and understand the difference between direct realism vs idealism.
So are you a deliberate Sophist, or just a conditioned unawake innocent globe-cult member born into this belief system like we all were?
Which one are you? I guess your next post will make it clear.
Come on over to Beyond the Imaginary Curve and bring practical, repeatable, testable proof of your extraordinary claims.
Either way I'm done here and yes this video is still fucking drivel...lol
because it summarizes some of the information in this thread...
Lets keep at them online but don't forget to hit the streets and talk to real people.
cheers, Gav.
Dear oh dear, Mr duped ones and/or sophist deceivers ...Let me list some of your fallacies:
1. not correctly differentiating between visible and tangible objects and their measures. Just because pool balls are round it doesn't make the billiard table a sphere.
2. believing you are spinning and upside down on a ball that contradicts our everyday common sense experience of a level motionless plane with orbiting luminaries. (The attempt above to use observed behavior as a positive claim of curvature is downright ludicrous)
3. trying to tie natural science observations to religious bullshit to deliberately discredit scientific objections, and to associate natural researchers with religious cults
4. believing that large bodies of water can conform to the exterior of shapes or that water can "schtickstein" to the outside of a ball. lol. Even Newtons analogous demonstrations with spinning buckets of water needed a container...bucket ..Duh.
5. shifting the burden of proof from those that claim we live on a spinning testicle in a vacuum to cries of "prove its flat", when nobody is making a claim, simply rejecting bullshit. Typical sophist crap. Ignoratio elenchi. Ignorance of the nature of refutation (of an argument).
6. Rely on common sense in every day life to stay alive, yet ignore/reject it in favour of a theory. LMFAO. Just hypocritical.
7. Relying on a formal science such as Mathematics (a man-made language of formal definitions) rather than Natural science to believe theories that contradict lived life.
Lets be clear about this:
It is CORRECT science to argue FROM the FACTS (motionless level water) AGAINST any absurd hypothesis that contradicts those facts (spinning water-covered testicles).
It is FALSE Pseudo-Science to argue FROM a Hypothesis (water can curve and we are spinning) AGAINST the known facts of lived life.
So show us your practical demonstration of the globe claim, with something other than cartoon imagery and plastic toys. If you can't it is pure shite that you are talking.
Dude, you better get a grip. Either you have not yet deconstructed your conditioned belief systems (try the socratic method on yourself and re-learn to think critically) or you are just a sophist troll deliberately perpetuating deceit for personal gain, in which case your days are numbered as people wake up and smell the bullshit.
Study the dynamics and behaviour of liquids/fluids, learn the Trivium (grammar, logic, rhetoric), trust your common senses and understand the difference between direct realism vs idealism.
So are you a deliberate Sophist, or just a conditioned unawake innocent globe-cult member born into this belief system like we all were?
Which one are you? I guess your next post will make it clear.
Come on over to Beyond the Imaginary Curve and bring practical, repeatable, testable proof of your extraordinary claims.
Either way I'm done here and yes this video is still fucking drivel...lol
vortexpuppy- Posts : 167
Points : 3602
Reputation : 296
Join date : 2015-12-30
 Re: Direct Vision, Rationality, Realism and Common Sense.
Re: Direct Vision, Rationality, Realism and Common Sense.
Vortexpuppy (Gav) it is a big pleasure to read all those informations from you about geometry, vision and common sense. Your materials helped me a lot to understand more technically this problem. Many thanks to for all your time and work, currently i'm translating best of this to polish language, to publicate it on polish version of IFERS.
Below video showing how perspecitve, from which we are viewing, can trick our eyes:
Below video showing how perspecitve, from which we are viewing, can trick our eyes:
 Re: Direct Vision, Rationality, Realism and Common Sense.
Re: Direct Vision, Rationality, Realism and Common Sense.
Last edited by Admin on Thu Jun 28, 2018 7:10 am; edited 1 time in total
 Re: Direct Vision, Rationality, Realism and Common Sense.
Re: Direct Vision, Rationality, Realism and Common Sense.
A high level discussion/summary of various things already in this thread.
Hope these discernments further more inquiry and support the necessary relentless dismantling of cult belief systems.
If empirical science is that of observation and experiment, and the Eye is arguably 80% + of our overall sensory experience necessary for lived life, and obviously 100% of every observation ever made, then it is an intricate part of ALL empirical science. Any part of the overall empirical process, from the making of a precision instrument, up to and including the observer of the experiment involves visual perception.
Why then is the geometry of visual perception ignored?
Why is there no mathematics describing visual space?
Why are there are no error ranges defined?
Why is it never openly discussed in academia?
That would be like ...
- Ignoring the role of language when two people communicate
- Or ignoring the role of the the ear in understanding sounds or the act of balancing
- Or ignoring the role of nerves of skin organ in determining and understanding hot and cold, rough and smooth
Keep at them and See you all on the level ...
Hope these discernments further more inquiry and support the necessary relentless dismantling of cult belief systems.
If empirical science is that of observation and experiment, and the Eye is arguably 80% + of our overall sensory experience necessary for lived life, and obviously 100% of every observation ever made, then it is an intricate part of ALL empirical science. Any part of the overall empirical process, from the making of a precision instrument, up to and including the observer of the experiment involves visual perception.
Why then is the geometry of visual perception ignored?
Why is there no mathematics describing visual space?
Why are there are no error ranges defined?
Why is it never openly discussed in academia?
That would be like ...
- Ignoring the role of language when two people communicate
- Or ignoring the role of the the ear in understanding sounds or the act of balancing
- Or ignoring the role of nerves of skin organ in determining and understanding hot and cold, rough and smooth
Keep at them and See you all on the level ...
vortexpuppy- Posts : 167
Points : 3602
Reputation : 296
Join date : 2015-12-30
Page 3 of 5 •  1, 2, 3, 4, 5
1, 2, 3, 4, 5 
 Similar topics
Similar topics» CoronaVirus and Forced Vaccination Manipulation
» CoronaVirus and Forced Vaccination Manipulation
» No Curvature on the Flat Earth
» Devin Madgy and Quasiluminous Blood Over Intent Non-Sense
» World Space Agencies
» CoronaVirus and Forced Vaccination Manipulation
» No Curvature on the Flat Earth
» Devin Madgy and Quasiluminous Blood Over Intent Non-Sense
» World Space Agencies
IFERS - Exposing the 'Global' Conspiracy From Atlantis to Zion :: The International Flat Earth Research Society
Page 3 of 5
Permissions in this forum:
You cannot reply to topics in this forum